Last Updated on July 28, 2025 by Maged kamel
CB-Bending coefficient-moment gradient factor part 1 for steel beams.
How do we estimate the CB-bending coefficient values for different loading types and bracing locations?
We will discuss a new subject: determining the Cb-bending coefficient, also called the moment gradient. We will calculate it for different loading and bracing. For all the previous examples, we have considered the value of Cb=1, whether for the estimation of nominal design strength or the design of a given section.

The different values of the CB-bending coefficient or moment gradient factor Cb are shown for the various types of loading for a simple beam, as quoted from Prof. McCormack’s book.
The first figure shows a simply supported beam with a uniformly distributed load W-ult k/ft.
The bracings are at the support left end and the proper support; each bracing is shown as an X mark.
For the unbraced length =l, the value of Cb = 1.14 for the first case.
The following figure shows a supported beam similar to the first case, but this time, a bracing is added in the middle of the span.
So, in this case, we have three bracings: on the left side, the middle side, and the right edge. The middle bracing is located at a distance =L/2 from the left support.
The uniformly distributed Load =W as the Ultimate load Kips/Ft; in that case, the cb-bending coefficient value is =1.30.
The third case is for a simply supported beam with a concentrated load acting in the middle. The CB-bending coefficient value for this case is =1.32 for two bracings only.
The fourth case is for a supported beam, similar to the third case, but with three bracings. The acting concentrated load is Pult. The Cb-bending coefficient-moment gradient for this case is 1.67.
Several cases involve two concentrated loads and bending moments acting at the supports. The Cb-bending coefficient value for the last case is 2.27.
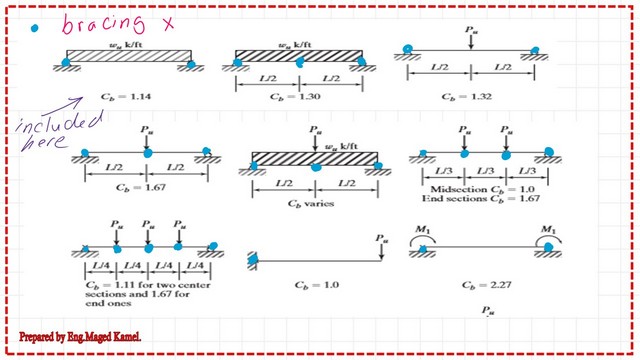
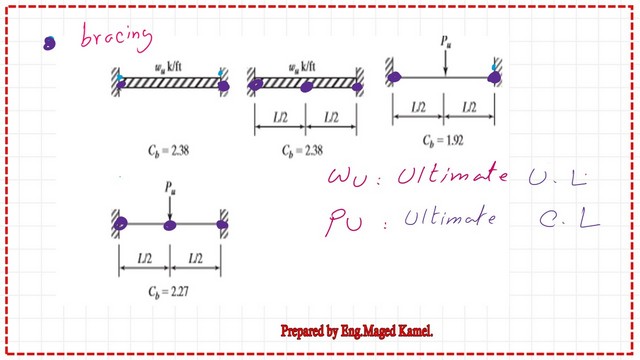
For statically indeterminate structures, we have a fixed-end beam under W-ult as a uniform load, with two bracings at the two edges. The CB-bending coefficient value is 2.38.
We have the CB-bending coefficient value =2.38 for the same beam but with three bracings.
The third case is for the fixed-end supported beam with a Pu load acting in the middle; the CB-bending coefficient value is =1.92. The last case is for two fixed-end supported beams with a Pu load acting in the middle but with three bracings; the CB-bending coefficient value is = 2.27.
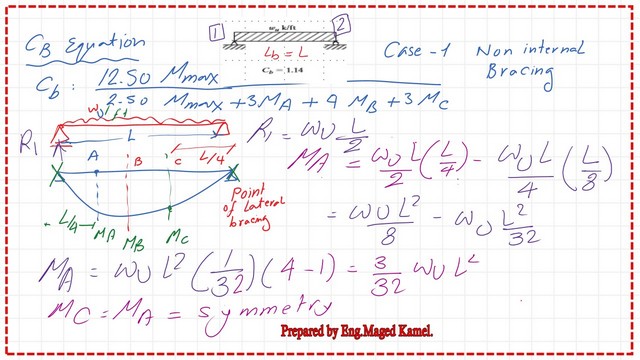
How do you estimate the moment gradient cb for a simply supported beam under a uniform loading?
Divide the distance between bracings into four equal parts, determine the moment at each part, and then use the Cb equation. Please refer to the next slide image.

The equation we use to evaluate the moment gradient factor Cb is cb=12.50*M-max/(2.50* Max+3*MA+4*Mb+3*Mc); the sum of the denominator values is 2.50+3+4+3=12.50.
A is the first quarter-point. B is the second quarter-point. C is the third quarter-point.
In the case of a simply supported beam under uniformly distributed W-ult k/Ft, for which we have two bracings, the first bracing is located at the left edge, while the second bracing is at the proper support.
As we are familiar with a simply supported beam under uniform loading, the M-max value is Mmax=W-Ult*L2/8, where L is the span distance.
This is the M-max for Ma value at the first-quarter point. First, estimate the reaction is W*L/2.
The moment caused by the reaction = Wult*L/2*L4, opposed by a moment for loading =Wul*(L/4)*1/2*(L/4)= the moment value Wul*l2/8-Wul*L2*1/32.
Finally, MA=3*Wult*L^2/32, due to symmetry Mc value is the same as the Ma, what is left is the Mb value.
For the Mb value, Wult*L^2/8, now we have all the information to estimate the Cb value,
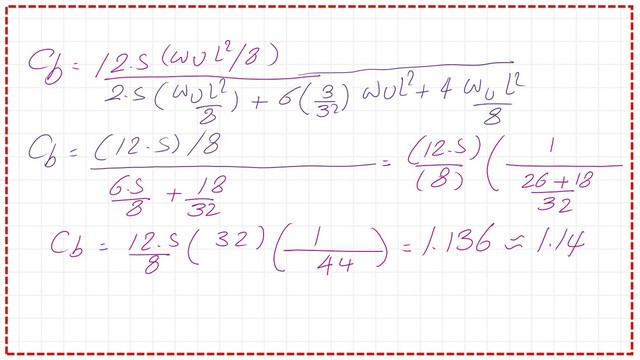
We have12.50*Wult*L^2/8/(2.5*Wult*L^2/8)+3* (3*Wult*L^2/32) +(4*3*Wult*L^2/8) +(3*Wul*L^2/32).
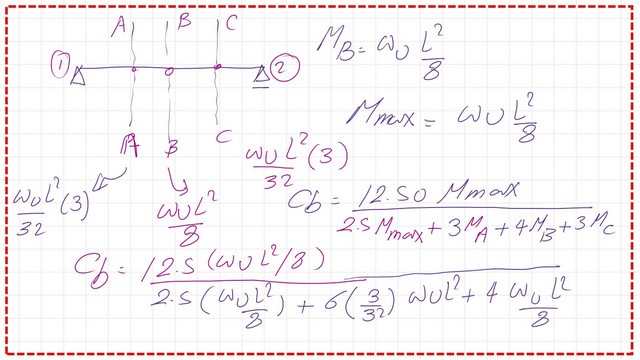
Take Wult*l^2 as a common factor; we have (2.50+ 18/32+4/8) for the denominator. The denominator sum=44/32. The CB equals (12.50*32)/(8×44)=1.14, the same result as the first case in Prof McCormac’s shape.
How do we estimate the moment gradient factor Cb for a fixed-end beam under a uniform loading?
Let us check another case. L is the span length under a uniform loading, with two bracings at the edges of a beam with two fixed supports. We know that the fixed-end beam moment equals Wu*L^2/12, while the positive moment equals Wul*L^2/24.
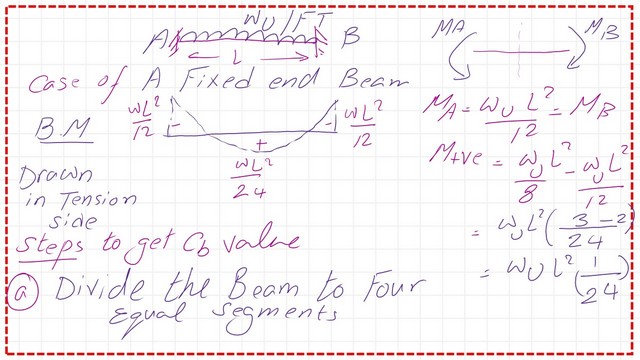
For the fixed beam, the Fixed end moment M-Fe=Wul*L2/12 if the uniform loading = W-ult k/Ft.
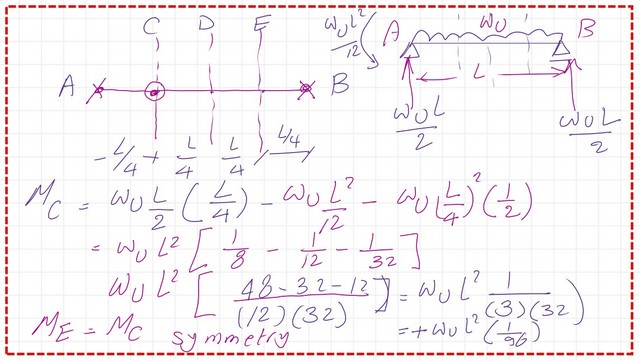
We will divide it into C, D, and E, where C is at a distance = L/4, D is in the middle, and E is L/4 from the right support.
We draw the bending moment diagram, but must estimate the reaction at the supports. We will perform the same exercise as before.
The reactions at supports A and B are equal, and the value of each is Wu*L/2.
The moment at point C=Wu*l/2*L/4-WUl^2/12-Wu*(L/4)*L/8=Wu*L^/96, due to symmetry at the middle of beam, MC=ME.
The Moment at Point D is equal to Wu*L^2/24 as expected. Please refer to the next slide image.
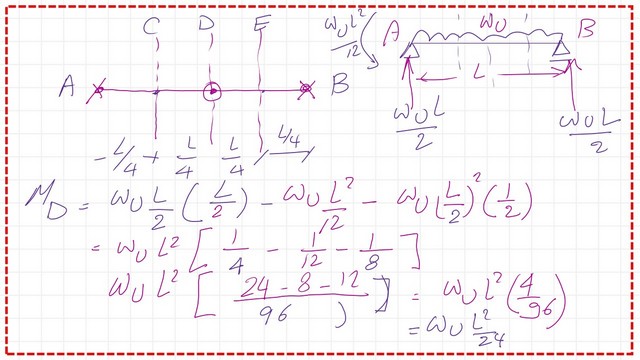
Apply the equation of Cb, we note that the maximum moment is Wu*L^2/12 is taken as a value without the negative sign.
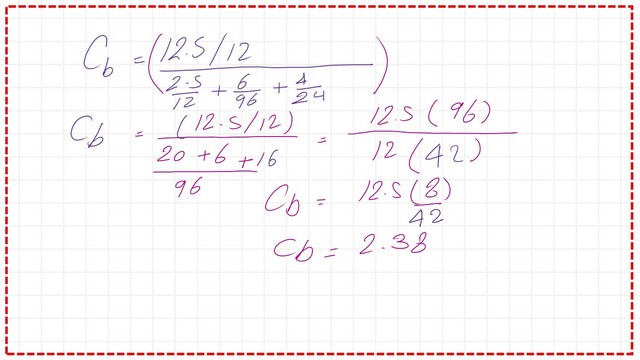
The numerator=12.50*Wul*L^2/12/(2.50*W*L^2/12+3*(1/96)*Wul*L^2+4*Wult*L^2/24+3*(Wul*L^2/96). So we have in the denominator: WulL^2(2.50/12)+(6/96)+(4/24).

Summing values (2.50/12)+(6/96)+(4/24) results in (12.50/12). The coefficient of moment (Cb) or moment gradient factor for the fixed-end beam is 2.38.
The moment gradient factor Cb, or the coefficient of moment, equals 2.38 for the fixed-end beam.
Thanks a lot, I hope you like the content of this Post.
For more detailed illustrations of the CB, please follow this Flexural Limit State Behavior.
A Beginner’s Guide to the Steel Construction Manual, 16th ed. Compression Flange Local Buckling Limit State
This is the complete list of all posts related to Cb:
1-Introduction to Cb-Bending coefficient part-1 for steel.-post 17-this post.
2- Cb-The coefficient of bending part 2 for steel beams-post 18-next post.
3-Cb-The coefficient of bending part-3 for steel beams-post 18a.
4-Cb value-bracing at the midpoint of a beam-uniform load-Post 18b.
5-Cb value bracing at third points of a beam, U load-Post18C.
List of published posts –2a for the previous posts.
