Last Updated on February 12, 2024 by Maged kamel

List of compression Member Posts-part 2.

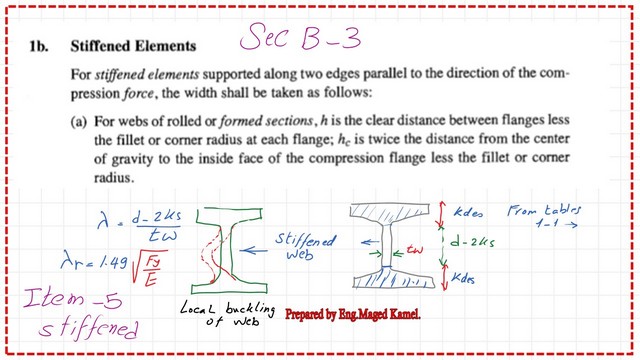
11- Local Buckling stiffened and unstiffened.

This is the 11th post of the compression Member Posts-part 2 , which includes the local buckling classification of sections for local buckling, for compression, sections are classified as a non-slender element or slender element.

For non-slender elements, We have two factors, one factor for flange and the other is for the web. if the b flange / t flange < certain ratio λr. The section is the non-slender. the ratio, which we have already talked about via tables. h web/ t web < λr .

For a certain factor then the section is non-slender, if bigger than λr shown in the table, then the section is slender, then a reduction of the coefficient.

There are two coefficients, the first one is Qs and the second coefficient is Qa, the Qs is for the flange, if the lambda λ, is > the ratio is given, While the Qa is for the effective area/ A gross. Non -slender not exceeding λr, from table B4.1a,

This is the link to post 11: Local Buckling stiffened and unstiffened.

12- Solved problem 5-2 for local buckling.

This is the 12th post of the compression Member Posts-part 2 which includes solved problem-  5-2 is quoted From Prof. Jack McCormac’s handbook.

a) Using the column critical stress values in Table 4-22 of the Manual, determine the LRFD design strength and the ASD allowable strength for the column shown in Fig. 5.8.

If 50-KSI steel is used. (b) Repeat the problem, using Table 4-1 of the Manual. (c) Calculate and, using the equations of AISC Section E3,

This is the link to post 12: Solved problem 5-2 for local buckling

13- A Solved problem 5-3 for local buckling.

This is the 13th post of the compression Member Posts-part 2 which includes Example 5-3. From Prof. Jack McCormac’s handbook. An HSS is used for an 18-ft-long column with simple end supports.

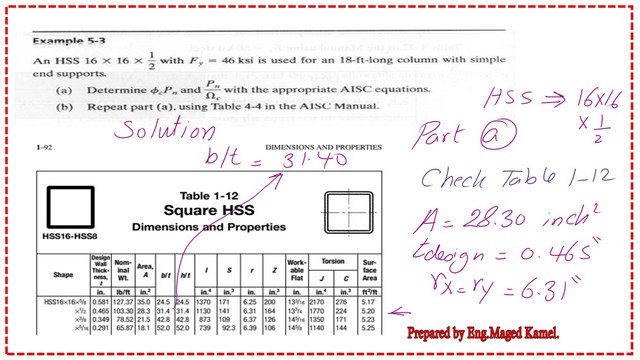
(a) Determine and with the appropriate AISC equations.
(b) Repeat part (a) using Table 4-4 in the AISC Manual.

This is the link to post 13: A Solved problem 5-3 for local buckling.

  14- Solved problem 6-8 for local buckling.

This is the 14th post of the compression Member Posts-part 2 which includes a Solved problem  6-8 From Prof. Jack McCormac’s handbook. Determine the axial compressive design strength and the allowable design strength of a 24-ft HSS column section.

The base of the column is considered to be fixed, while the upper end is assumed to be pinned.

This is the link to post 14: Solved problem, 6-8, for local buckling.

15- A solved problem 6-19-4 for local buckling.

This is the 15th post of the compression Member Posts-part 2 which includes a Solved problem  16-19-4 From Charles G salmon’s handbook. Example 6-19-4 Determine the nominal axial compressive strength φc*Pn for the nonstandard shape of Fig. 6.19.4 for an effective length KL equal to 8 ft. Use Fy = 100 ksi and the AISC LRFD Method.

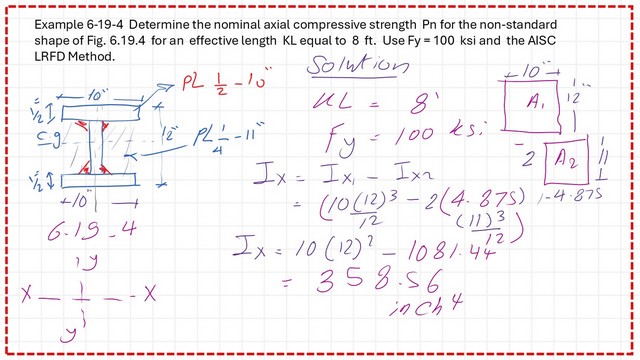
This is the link to post 15: A solved problem 6-19-4 for local buckling.

16-A solved problem 5-10 for local buckling.

This is the 16th post of the compression Member Posts-part 2 which includes a new example 5-10 from the unified design of steel structures by   Louis  F. Geshwendener.

In solved problem 5-10. It is required to determine the available strength of a compression member with a slender web, the section is W16x26 as a column with lcy =5.0 ft.

This is the link to post 16: A solved problem 5-10 for local buckling.

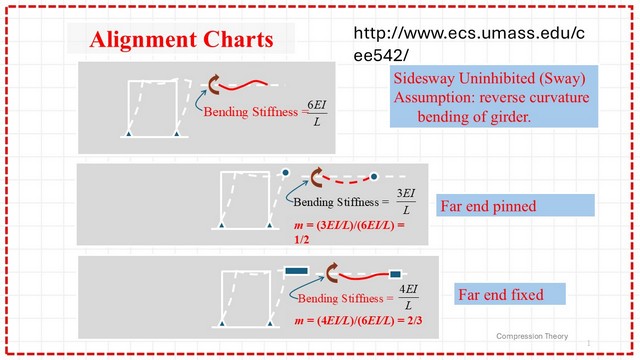
17-Alignment chart part 2.

This is the 17th post of the compression Member Posts-part 2 which includes the alignment chart, with conditions, of anti-symmetrical curvature for the unbraced frame.

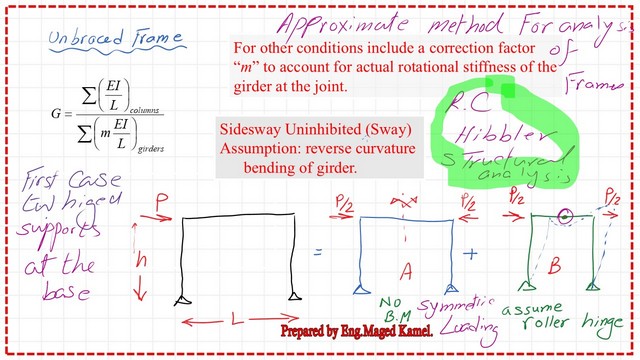
The Nomograph was based on the double curvature assumptions. we have a bending moment at one end, but at the other end, there is another moment, equal in magnitude, that will cause anti-symmetrical curvature.

This is the link to post 17: Alignment chart part 2. of the post.

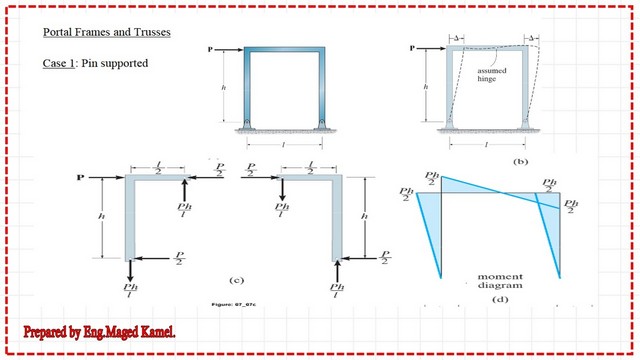
18-A Review of portal frames.

This is the 18th post of the compression Member Posts-part 2 which includes How to make a structural analysis, and we give an example, as we have a frame that has two hinged supports and is acted upon by a force at the left side.

The frame can be analyzed by dividing the frame into two parts, the first part has antisymmetrical loading and the second part has a symmetrical loading, and due to that loading the elastic curvature.

This is the link to post 18: the A Review of portal frames. of the post.

19-Modification to alignment chart unbraced frame.

This is the 19th post of the compression Member Posts-part 2 which includes discussing adjustments to be made for the G equation as the end condition for the girder does not match the requirement.

This is the link to post 19: Modification to alignment chart unbraced frame.

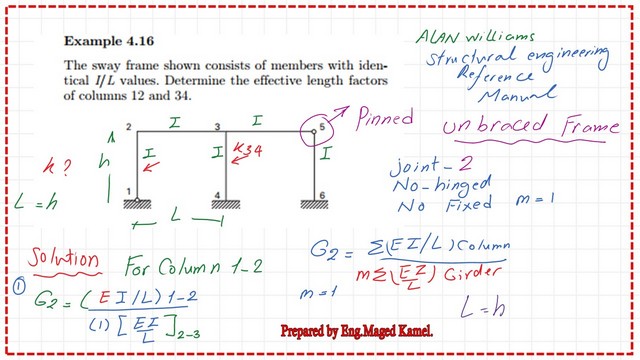
20-Solved problem 4-16 for k factor.

This is the 20th post of the compression Member Posts-part 2 which includes Our example is 4-16, from Prof. Alan Williams’s book, structural engineering reference manual. Given a sway frame, it means Unbraced frame, Unstiffened frame, sway frame shown consists of members with identical I/L values.

Determine the effective length factor for columns here are the columns. 12 and 34, he has not included any values for the length of girders or height of columns, we can consider that h and L are the same, it is required to get the K value for two columns,12 and 34,

This is the link to post 20: Solved problem 4-16 for- k factor.

This next list of compression member posts is part 3.

An external source for tension members from Prof. T. Bartlett Quimby’s site is the link.