- A Solved problem 5-2 for local buckling of columns.
- Brief content Content of the video.
- Detailed data for the Solved problem 5-2 for local buckling of columns.
- 1-Get the properties of section W12x72 from table 1-1 for the Solved problem 5-2 for local buckling
- 2-Check whether buckling is controlled by minor or major axis for the Solved problem 5-2 for local buckling
- 3-Check the bf/2tf value for the W-section-unstiffened section.
- 4-Check the hw/2tw value for the W-section-stiffened section.
- 5-Using table 4-22 for the available critical stress for compression members.
- If Using Table 4-1 for the solved problem 5-2 for local buckling.
- If Using the general provision for the available strength.
A Solved problem 5-2 for local buckling of columns.
Brief content Content of the video.
The first solved problem 5-2 for local buckling in which, the arrangements of the solution, the Qs, Qa will be considered as = 1 as a start, The solved problem 5-2 is From Prof. Jack McCormac’s handbook, Referring to the given section of W12x72, Height is 15 feet, fixed from the bottom and hinged from top, kx=ky=0.7, But, the recommended values for kx, Ky=0.80.
Using table 1-1 for the properties of the section of Wt, the section as shown in the sketch, bf=12 inches, the thickness of the flange is 0.67, the web thickness is 0.43, the overall height d=12.3 inch. First, we have to check, whether the column is short or long
We start by estimating the (Kl/ry) max (Kl/ry) at y, where ry is the minimum radius of gyration, we have k=0.8, l=15 x12=180 inch, That was a part of the video, which has a subtitle and closed caption in English.
You can click on any picture to enlarge, then press the small arrow at the right to review all the other images as a slide show.
Detailed data for the Solved problem 5-2 for local buckling of columns.
We are going to see, the first Solved problem for local buckling 5-2, in which, the arrangements of the solution. The Qs, Qa will be considered as = 1 as a start, solved problem 5-2 is From Prof. Jack McCormac’s handbook,
For the column shown in fig 5.8, if 50 ksi steel is used.
a) Using the column critical stress values in table 4-22 of the Manual, determine the LRFD design strength Φ Pn and the ASD allowable strength P/Ωc, for the column shown in fig 5.8.
b) Repeat the problem by using table 4-1 of the Manual.
c) Solve by using the AISC – equation E3.
1-Get the properties of section W12x72 from table 1-1 for the Solved problem 5-2 for local buckling
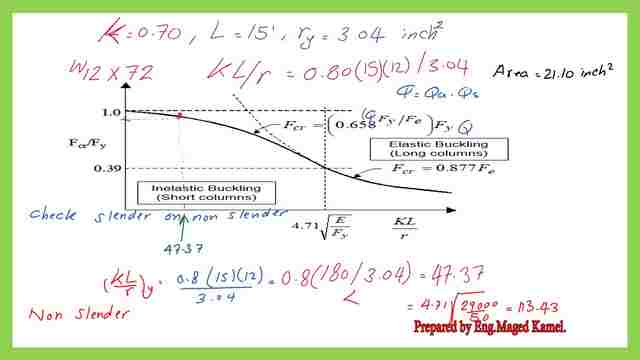
Referring to the given section of W12x72, Height is 15 feet, fixed from the bottom and hinged from top, kx=ky=0.7. But, the recommended values for kx, Ky are taken equal to 0.80.
Using table 1-1 for the properties of the section of Wt, the section as shown in the sketch, bf=12 inch, the thickness of the flange is 0.67, the web thickness is equal to 0.43, the overall height d=12.3 inch.
2-Check whether buckling is controlled by minor or major axis for the Solved problem 5-2 for local buckling
We have to check, whether the column is short or long, so, we start by estimating the (Kl/ry) max (Kl/ry) at y, where ry is the minimum radius of gyration, we have k=0.8, L=15 x12=180 inch, ry=3.04 inch 4, kl/r at the y is 47.37, for Fy =50 ksi.
Our equation 4.71*sqrt(E/Qfy), is known to be =113.43 for Fy=50 ksi, the kl/r at the y-direction is less than 113.43.
The column is short.
3-Check the bf/2tf value for the W-section-unstiffened section.
We proceed to the next slide, and check the ratio of the flange to the width, unstiffened section, as bf/2tf= 12/(2* 0.67)=8.96, Compare this value with the value in the table for the unstiffened section.
The controlling bf/2tf is =0.56*sqrt(E/Fy) . refer to equation E-7-4.
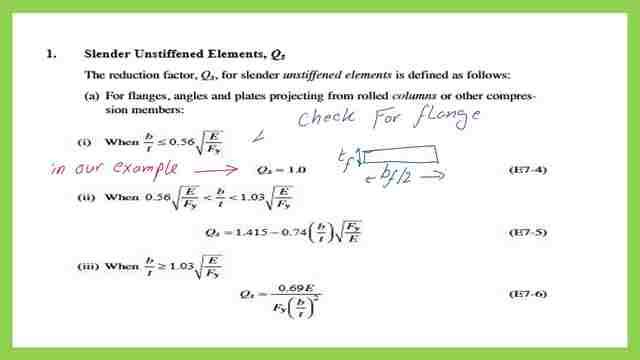
For hot rolled when b/t<= 0.56 *sqrt (E/fy), then the Qs is =1, 0.56 *sqrt (E/fy),E=29000 ksi, Fy =50 ksi.
The limiting Kl/r = 0.56 *sqrt (E/fy)=0.56 *sqrt (29000/50) = 13.49.
Since bf(2*tf) of our section = 8.96 which is less than 13.49, then the element is not slender, but a short column.
Please refer to the next slide image for given data for the solved problem 5-2 for local buckling of columns for a more detailed estimation.
4-Check the hw/2tw value for the W-section-stiffened section.
The next step is checking the web from the previous check of the flange, Qs=1, for the Qa relating to the stiffened element, the web from the next slide, the overall height d is 12.3 inch, k-des is obtained from the table, kdes=1.27 inch multiplied by 2, and subtract from 12.3 inches,d-2ks=12.3-(2*1.27), then divide by the web thickness, 0.43, the result is 22.7.
Refer to the coefficient λr for stiffened element case number 5.
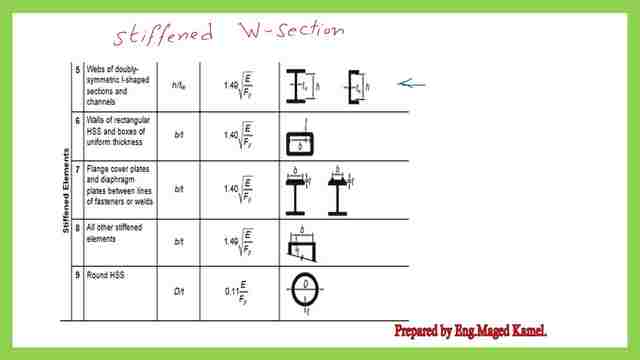
The detailed illustration when b/t is more than 1.49*Sqrt*(E/Fy).
The ratio (1.49 sqrt(E/fy)=1.49*sqrt(29000/50)=35.89, the W section stiffened part is case Number -5.
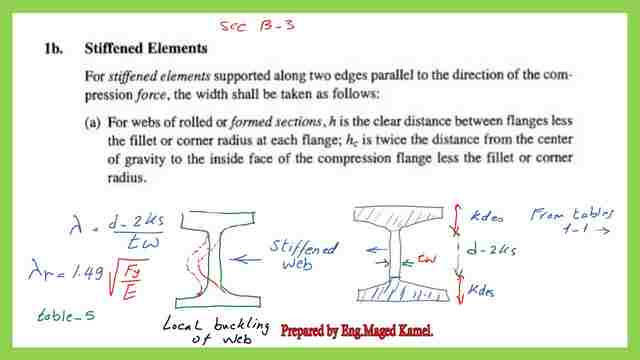
Our web ratio hw/tw= 22.7 < 35.89.
Then the section is non-slender, stiffened regarding the web, and non-slender unstiffened regarding the flange.
let us review all the findings, first, the column is short, secondly, the flange, is non-slender, and also the web is non-slender, then Q=1.
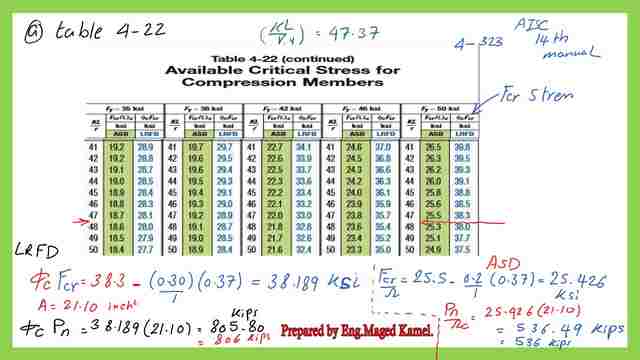
5-Using table 4-22 for the available critical stress for compression members.
Refer to the table for the kl/r and refer to the fy=50 ksi, our kl/r =47.37, this value is within the range of 47 and 48.
The table will give Fcr corresponding to both the LRFD and ASD.
For LRFD, the value is between 38.3 and 38.0, by interpolation.
We will get the value of 38.189 ksi For the LRFD of the solved problem 5-2 for local buckling.
While for ASD, the value is between 25.5 and 25.3. The final value is 25.426 for the ASD, to estimateΦc* Pn and Pn/Ω.
For the ASD, Fcr/ Ω=25.426 ksi as stress will be multiplied by the area, the value of Pn/Ω=536 kips approximately
. Finally, the Φpn =806 kips=806 kips, while for the ASD Pn/Ω=536 kips, thus we have completed part a, as for table 4-22. Φ*Fcr =38.189 ksi as stress will be * area which is 21.1, then Φc*Pn =805.80 kips approximated to 806 kips.
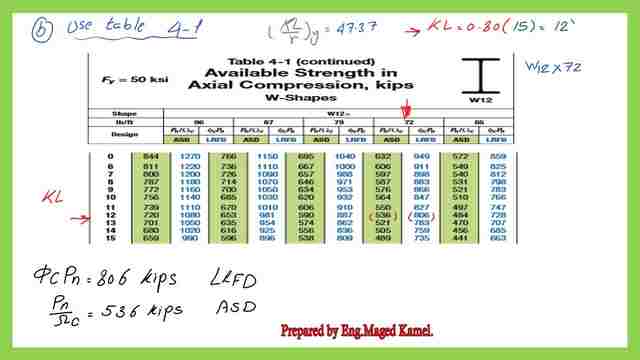
If Using Table 4-1 for the solved problem 5-2 for local buckling.
Then for part b, for the solved problem 5-2, using table 4-1, as part b for the solved problem the main difference between the two tables is that table 4-1 is using the coefficient Kl, not Kl/r as used by table 4-22, previously our K*l/r=47.37, while, our kl= 0.8* 15=12 feet as a reminder, our column is fixed-fixed and k recommended =0.8 and the height is 15 feet log in by 12 ft from the left side, and from the top select the W12x72, as a vertical line.
.The intersection between the two lines, the value of 806 and 536 kips and 536 kips, the same values obtained from table 4-22 item a, these values matching each other.
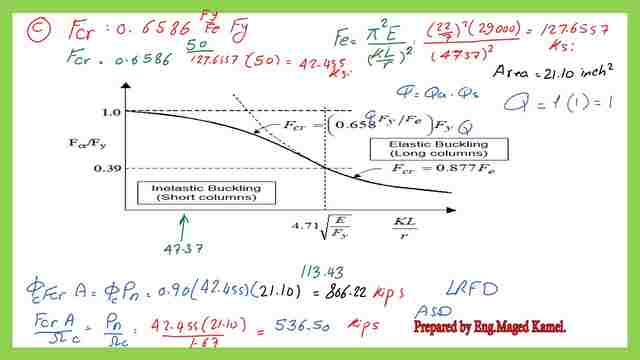
If Using the general provision for the available strength.
In the third step, it is required to estimate the values by the general equation, which is part c for the solved problem 5-2, this is the general equation, for the short column we are dealing with the curve at the left side of the 113.43 line.
The equation of the curve is given by Fcr= 0.658^(Q*fy/fe)*QFy, since Qs=Qa=1 then Q=1, First for Fe=Pi^2E/(kl/r)^2=(22/7)^2(29000)/(47.37)^2, fe=127.6557 ksi,.
Fcr=0.658^(50/127.6557), all multiplied by(1*50), fcr=42.455 ksi, to be multiplied by phi=0.9 by area=21.1.
We get Φ*Pn =806 kips for the LRFD.
In the case of ASD, for Ω=1.67, then Pn/Ω=42.455*(21.1)/1.67 Pn/Ω=536.5 kips, which is matching with the values obtained from table 4-22 and table 4-1.
This is the pdf file used in the illustration of this post.
The next post: solved problem 5-3 for local buckling.
This is a great external reference for limit state, here is the link: Limit State of Flexural Buckling for Slender Sections
