Last Updated on July 29, 2024 by Maged kamel

Cb value-bracing at the midpoint of a beam-uniform load.

In this post, we will estimate Cb value-bracing at the midpoint of a beam-uniform load. the case where we have three braces one at the midpoint and two at the supports.

In the previous post, number 17, we estimated the CB value or the coefficient for a moment for a simply supported beam with two braces at the supports under uniform load.

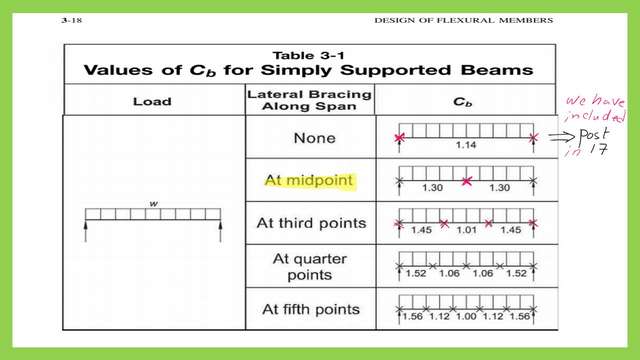
The next slide image shows the different cases for a simply supported beam with different CB values based on the bracing locations in the span.

Cb value-bracing at the midpoint of a beam-uniform load-simple beam case.

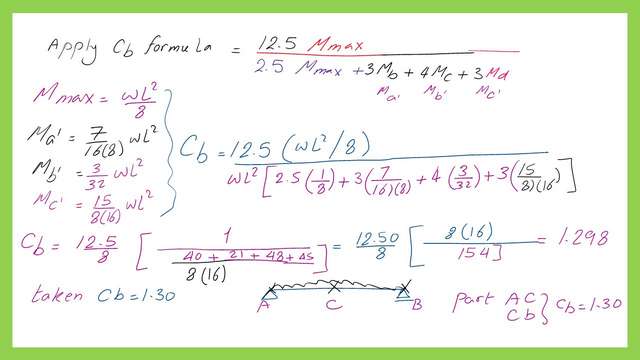
We can see that we have a brace in the mid-span of a simple beam under uniform load W. The midpoint is point C.

To estimate CB value-bracing at the midpoint of a beam-uniform load, we divide part Ac into four quarters and calculate the value of moments at the different points.

What is the maximum value for a moment?

The moment value at point A’ is estimated as equal to ((7/(16*8))*wL^2, where L is the span and w is the uniform load per kip or meter based on the used units.

The moment value at point B’ is estimated as equal to (3/32)*wL^2, and the moment value at point C’ is estimated as equal to ((15/(16*8))*wL^2. The moment at point c is equal to (1/8)*wL^2. Exploring the moment values for segment AC we find that the maximum value is WL^2/8.

Now, we will apply the equation to get Cb value-bracing at the midpoint of a beam-uniform load. The maximum moment is w*l2/8, which will be multiplied by 12.50 for the numerator. For the denominator, we will sum 2.5*Max +3Ma’, 4Mb’, and 3Mc’.

The Cb value for a simply supported beam will be found to be equal to 1.3, which matches the Cb value for the second case in Table 3-1.

The Cb value for segment Bc will be equal to 1.3 because of the symmetry.

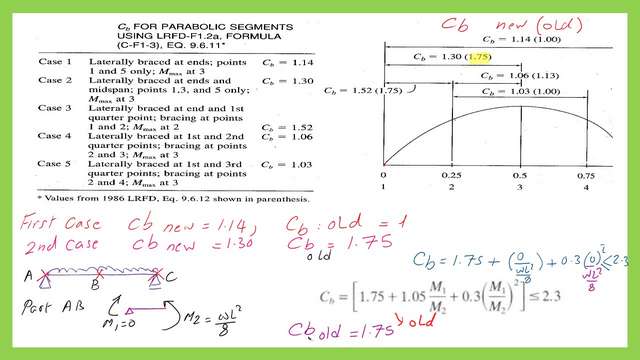
If you wish to compare the value of Cb based on the equation to the old value, we will find that the old value of Cb will be equal to 1.75 since m1/M2 will be equal to zero.

The next slide details how to find the old Cb value.

Cb value-bracing at the midpoint of a beam-uniform load-fixed end beam case.

The second case is a fixed-end beam with three braces, two at the support and one at the midpoint.

We will find the Cb value-bracing at the midpoint of a beam under uniform load. As we know from our structural analysis study for a fixed-end beam under uniform load, the fixed end moment of such a beam will be equal to W*L2/12, where w is the uniform load, and L is the span of the mean.

The middle moment can be found by superposing the positive WL2/8 and the fixed end moments of W*L2/12.

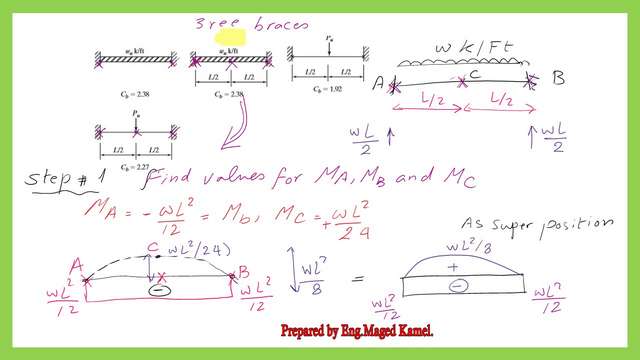
To estimate CB value-bracing at the midpoint of a beam-uniform load, we divide part AC into four quarters and assess the value of moments at the different points. The next slide image includes the steps to find M’a and M’b moment values.

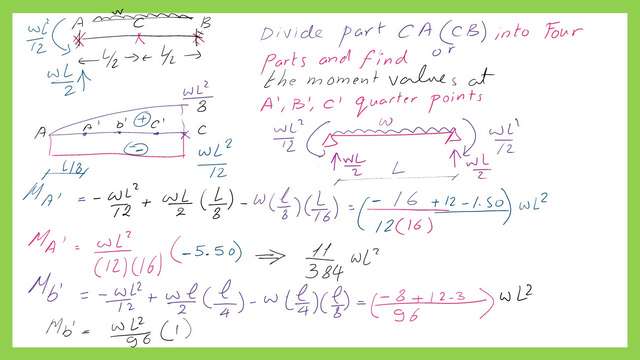
The next step is to estimate the moment for c’ in the third quarter for segment Ac. The value of the moment at c’ will be equal to (13/384)*w*L2. The value of mc is w*L2/24.

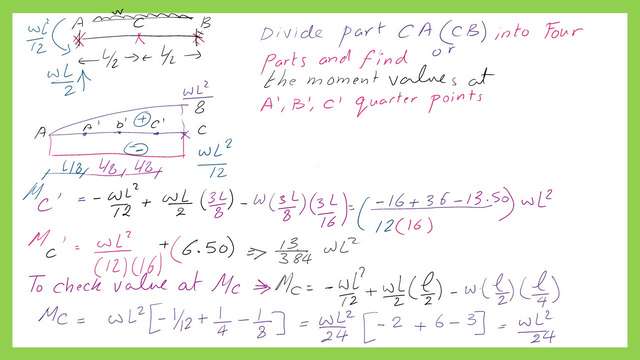
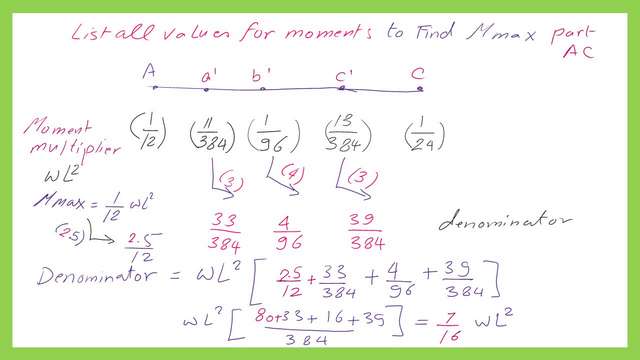
I have listed all the values for moments at points A, a’, b’, c’, and point c. Considering the maximum value of the estimated moment value, which will be found to be equal to Wl2/12, we consider the absolute value of moments.

What is the maximum value for a moment?

The denominator values are prepared using (2.5M max+3*Ma’+4*Mb’+3Mc’) as a multiplier of w*L2. The final value is (7/16)*w*L2.

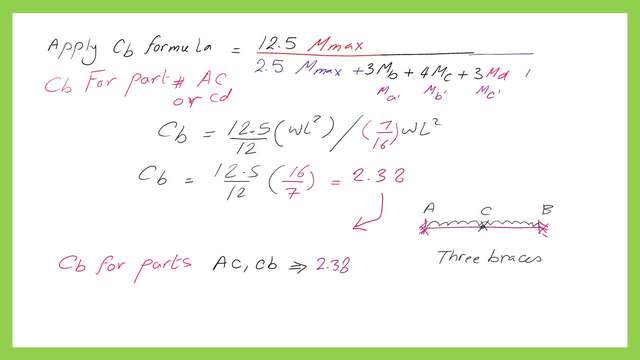
Apply the equation for Cb to get the coefficient of moment for the fixed end beam with three supports, two at the supports and one at the middle point. The value is 2.38, which matches the value shown. for segemnts AC and CB.

Please refer to table 8.2.1.1 Computation of Cb from A Beginner Guide to Structural Steel Manual 15th Edition.

This is the complete list of all posts related to Cb:

1-Introduction to Cb-Bending coefficient part-1 for steel.-post 17.
2- Cb-The coefficient of bending part 2 for steel beams-post 18.

3-Cb-The coefficient of bending part-3 for steel beams-post 18a -Previous post

4-Cb value-bracing at the midpoint of a beam-uniform load-Post 18b-This post.

5-Cb value bracing at third points of a beam-U load-Post-18C-Next post.