Last Updated on March 9, 2026 by Maged kamel
A solved problem for compound interest.
Detailed expression for compounded continuously.
This is the equation used to estimate the future value of continuously compounded payments, where (e) is Euler’s number. We will need to use the equation for the third option in the next solved problem, as we will see later on.
A Solved problem, the reference is the Time value of money part-1.
It is required to select the correct option from the four given options, which is the least amount to be deposited today to get $10000 after the given number of years from today? The interest rate options vary by interest rate and by interest frequency.
Option-1 for the solved problem for compound interest.
This is the first option. How do we find the present value P0 to get $10,000 after 4 years at 8 interest compounded quarterly?
The relation of P0=F*(P/F,i%,n) will be used, provided that the new interest rate is adjusted to be (0.08/4). The term (1+i/n)^nt=(1+(0.08/4))^(4*4), since the number of years=4, the present value=F/(1+i/n)^nt=10,000/(1+0.02)^(4*4)=$7284.45.
Option-2 for the solved problem for compound interest.
This is the second option: how to find the present value P0 to get $10,000 after 5 years at an interest rate of 7% compounded yearly?
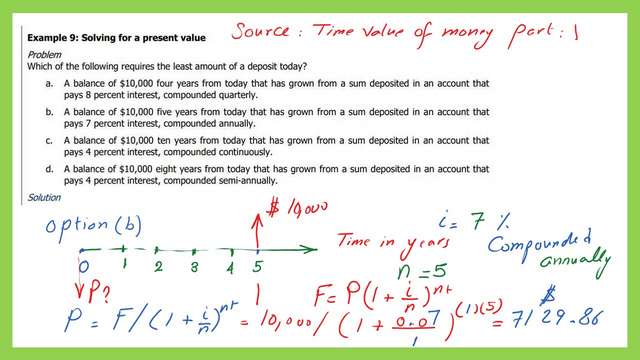
The relation of P0=F*(P/F,i%,n) will be used provided that the new interest rate is. The term (1+i/n)^nt=(1+(0.07/1))^(1*5), since the number of years=5, the present value=F/(1+i/n)^nt=10,000/(1+0.07)^(5)=$7192.86.
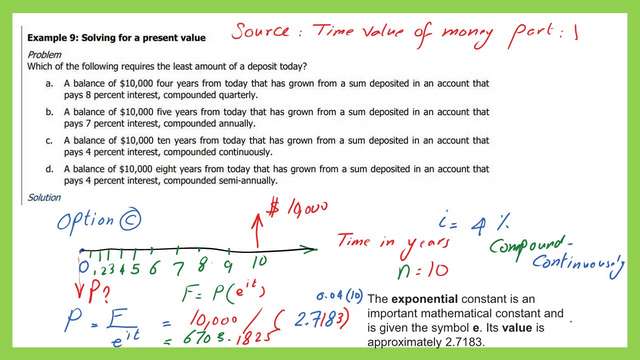
Option-3 for the solved problem for compound interest.
This is the third option, how to get the present value P0 to get $10,000 after 10 years, with an interest of 4 % compounded continuously?
The relation of P0=F*(P/F,i%,n) will be used provided that the new interest rate is, and the Euler value e is taken as 2.7183; is used for the case of compounded continuously.The term (e)^it=(2.718))^(0.04*10), since the number of years=10, the present value=F/(e^)it=10,000/(2.718))^(0.04*10)=$6703.1825.
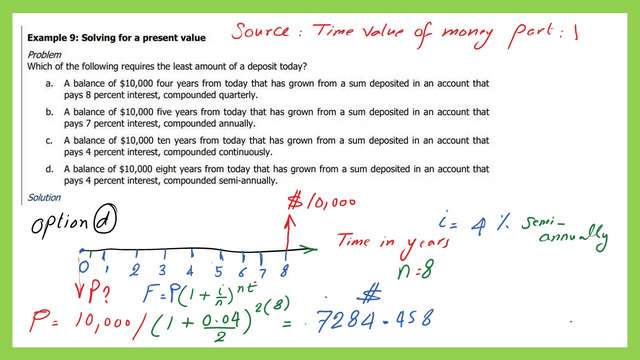
Option 4 for the solved problem for compound interest.
This is the fourth option: how to find the present value P0 to get $10,000 after 8 years at 4 interest compounded semi-annually?
The relation of P0=F*(P/F,i%,n) will be used, provided that the new interest rate is 4%, compounded semi-annually.The term (1+i/n)^nt=(1+(0.04/2))^(2*8), since the number of years=8, the present value=F/(1+i/n)^nt=10,000/(1+0.02)^(16)=$7284.458.
Among the above options, the lowest P0 value is $6703.2, which is option C.
The PDF file for this post can be viewed or downloaded from the following link.
For a valuable external resource, Engineering Economy is a good reference.
The next post is post 7– Approach to Uniform series of compound interest-1/2.