Last Updated on July 14, 2025 by Maged kamel
Moment of inertia Iy for the Trapezium.
For the moment of inertia Iy for the trapezium, we will start estimating Iy about Cg and later find Iy in the left corner.
First, we will consider a symmetric trapezium about a vertical axis passing by the Cg, which we call y’.
The Trapezium with base b and the upper top side a and height h is divided into the following shapes:
1-Two rectangles of base a/2 and a height of h, Areas are A1, A2 each area =1/2*(a/2) h, it is CG is apart from the y’-axis by a distance x1= (a/4).
2-A left triangle of base C and a height of h, its area A3=1/2*C*h, it is CG is apart from the y’-axis by a distance x2=-(a/2+1/3C).
3-A right triangle of base C and a height of h, its area A4=1/2*C*h, it is CG is apart from the y-axis by a distance x3=(a/2+1/3C).
The next slide image shows the division of the trapezium into four areas, where Y is the axis of symmetry.
We will estimate the inertia Iy’ for only one rectangle and one triangle and later multiply the value by 2. The value of Iy’ for the rectangle is, as shown in the next slide, equal to (h*a3/24). For the triangle, we will estimate the inertia about its Cg as equal to h*c3/36 as C is the base. We will add the product of the triangle area by the square of the Cg distance to the Y’ axis.
The Cg distance is equal to (1/6*(3a+2c). The calculation of both of the inertias for rectangle and triangle are shown in the previous slide image.
Moment of inertia Iy’ for the Trapezium about the Cg vertical axis.
Adding the two values of inertia and then multiplying by 2 will give us the final value of inertia Iy for the trapezium. We have used c as the base distance; later, we will equate it to (b-a)/2.
For the terms inside the bracket, we will get their values in terms of b and a. We have the first term as 6h*c^3, and the second term is 9h*(a^2*c). The third term is 12*hC^2*a—the last term 3h*a^3. Iy for the trapezium is obtained after getting the values of the four terms, which are shown in detail in the next slide image.
Please refer to the following slides for more details about simplifying the terms.
Moment of inertia Iy for the Trapezium in terms of b &a and h.
Finally, the Iy’ for the trapezium can be obtained. This is the final value for Iy for the trapezium, where the y’-axis passes by the CG. The inertia for a trapezium about the vertical axis y’ that passes by the Cg will be equal to h/48*(a+b)*(a^2+b^2), where a is the upper part length and b is the lower part length for the trapezium.
The square value of the radius of gyration about y’ axis passing by the Cg equals (a^2+b^2)/24.
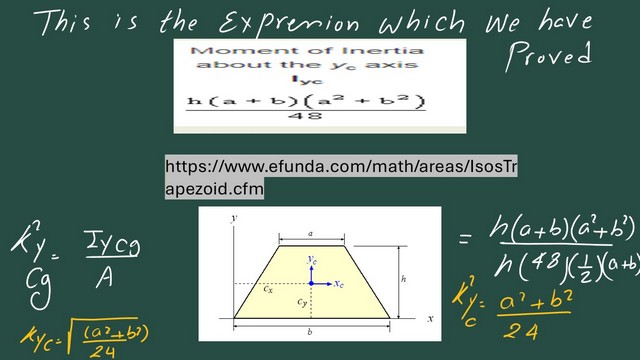
Moment of inertia Iy for the Trapezium at an external axis.
The inertia Iy for the trapezium about the Y-axis that passes by the left point can be obtained by adding the value of Iy’ to the product of the trapezium area by the square value of the Cg distance to the y-axis. The final value of Iy is shown in the next slide image.
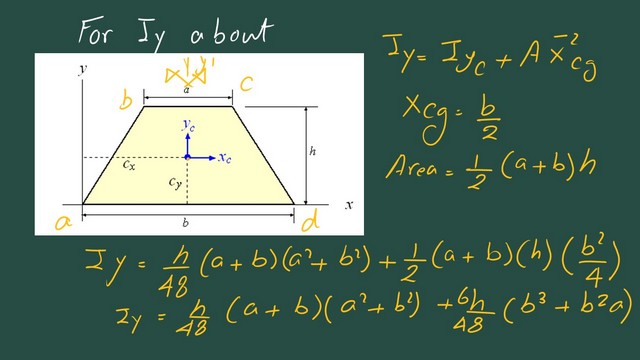
The radius of gyration value for inertia Iy for the trapezium.
The radius of the gyration value is shown in detail in the next slide image.
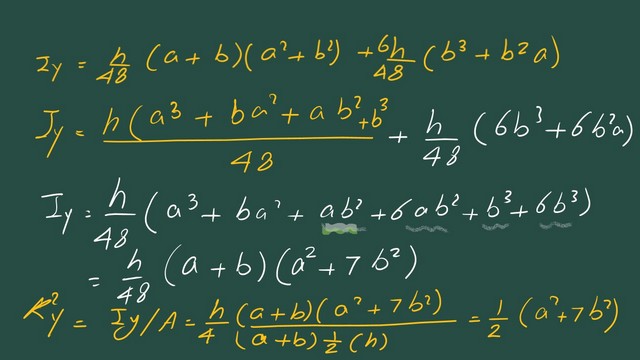
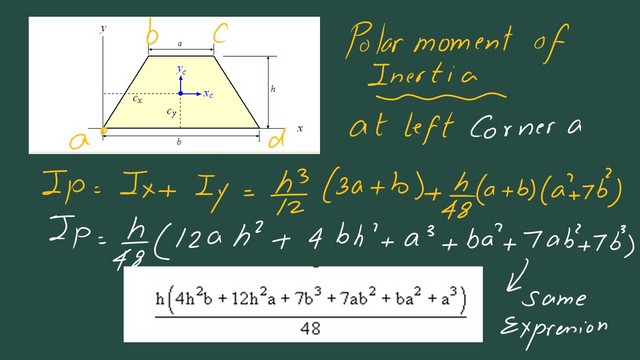
The Polar of inertia value IP for the trapezium.
This is the polar inertia value for the trapezium shown in detail in the next slide image.
For an external resource for the inertia values, please find this link to the e-funda.
This is a link to the complete details of how to get the x-bar and y-bar for a Trapezium.
This is a link to the post for the Ix value for the trapezium.
The next post will be about getting the product of inertia for a parallelogram.