Last Updated on April 7, 2023 by Maged kamel

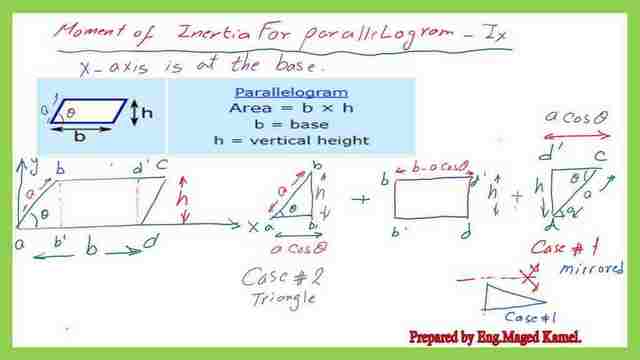
Moment of inertia Ix for parallelogram.

Divide into areas and estimate inertia for each individual area about the x-axis.

The post includes how to estimate the moment of inertia Ix for a parallelogram. The axis x is located at the base of the Parallelogram. A parallelogram is a skewed rectangle with an angle=θ, between the base and the left side. When θ=90, the shape becomes a rectangle.

The dimension of the parallelogram is b*a, whereas the side length and the height is=h, h can be considered as=a*sinθ.

To get the expression for Ix, we will divide the parallelogram into two triangles and a rectangle, and we will use the previous data for the moment of inertia about the x-axis that was obtained for inertia for the right-angle triangle and rectangle.

Here are the relevant data for Ix for the right-angle-case 1, Ix for the right-angle triangle case-2, and Ix for the rectangle.

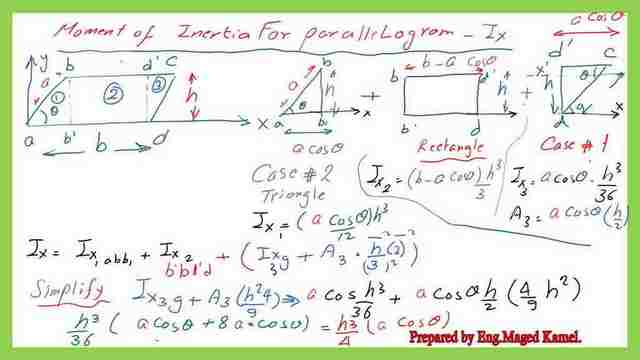
The left triangle has a base of (a* cos θ) and height h, the inertia for the x-axis will be estimated about an axis passing by the left corner point of the base of the Parallelogram. Ix for that triangle=base*height^3/12, or (a* cos θ)*(h^3/12).

As for the rectangle about x-axis, Ix=base*height^3/3=(b-(a* cos θ)*(h^3/3).

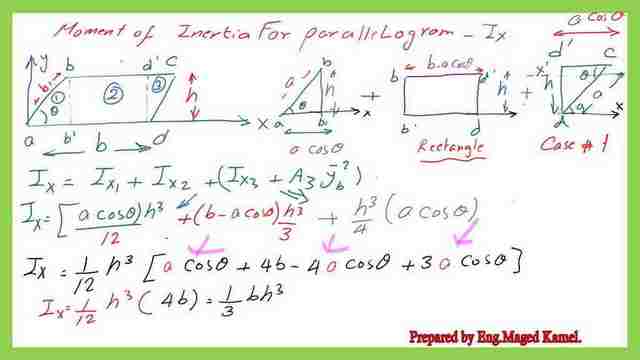
For the last triangle inertia, Ix will be deducted, it is case-1 of the right-angle triangle, but this angle is inverted, we will get Ix about the Cg and add the multiplication of area by the y_cg ^2 which is ((2/3)*h)^2=(4/9)) h^2.

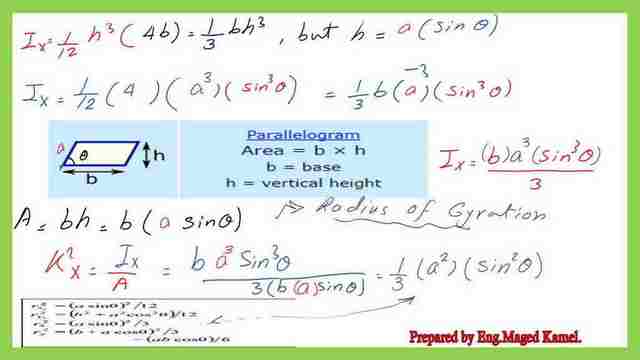
The common items will be grouped and adjusted. The final expression for inertia Ix for parallelogram can be estimated as Ix=(1/3)bh^3. To get an expression in terms of a, the value of inertia Ix for parallelogram will be=ba^3(sin θ)^3(1/3).

The radius of gyration (r_x)^2 for the parallelogram about the x-axis.

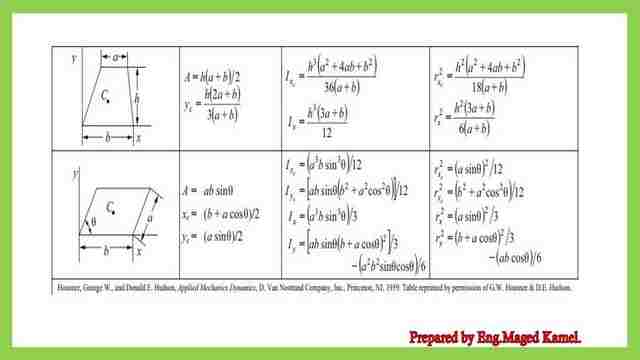
Since the expression for rx^2=Ix/Area, we will divide the inertia Ix for a parallelogram by the value of the area, the final expression is shown in the next slide image and compared by the value obtained from the FE Reference Handbook.

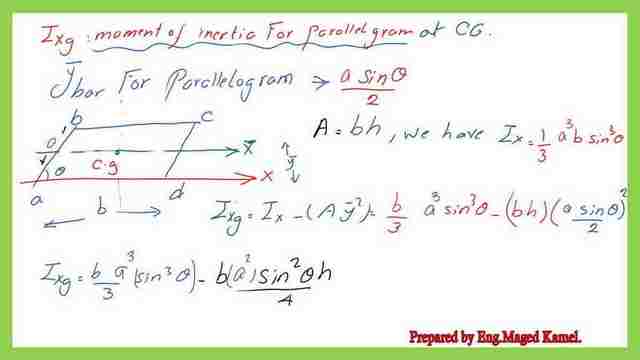
The radius of gyration (r_x)^2 for the parallelogram about CG.

The expression for the moment of inertia about Cg can be derived by using the parallel Axes theorem, we consider Ix for parallelogram about the X-axis and subtract the product of the area*ycg^2 from the Ix value. We have the Cg distance y cg=1/2(a*sin θ) from the X-axis that passes by the base, Area is =b*h. The final value and the relevant calculation can be derived from the next slide image.

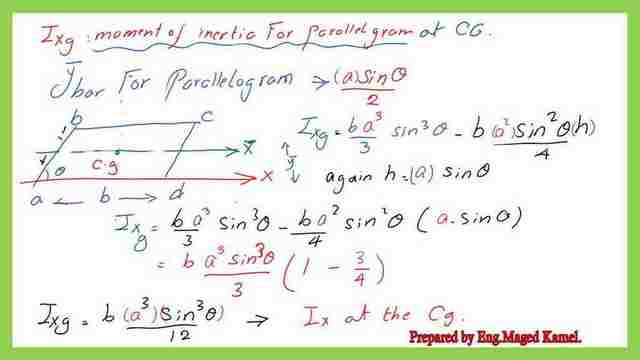
A modification of similar terms is to be conducted, h value is to be replaced by the equation h=aasin θ. the final term of Ix cg=(1/12)ba^3*(sin^3 θ).

The square value of the radius of gyration for the parallelogram about the Cg in the x-direction can be estimated by dividing the inertia Ix for the parallelogram at the CG over the area, Ixg/A, we have Ixg=(1/12)*b*a^3*(sin^3 θ), while the area of the parallelogram=b*h, rg^2=(1/12)*b*a^3*(sin^3 θ)/(b*h).

The square value of the radius of gyration about the Cg in the x-direction can be found to be =(1/12)*a^2*(sin^2 θ).

The rectangle inertia Ix is similar to the inertia Ix for a parallelogram, for which the angle θ=90 degrees, then the square value of the radius of gyration about the Cg in the x-direction for the rectangle is =(1/12)a^2(1), a is the height h, finally =(1/12)*h^2, please refer to the next slide image for more details.

The slide image contains the values of the moment of inertia for the parallelogram, and the radius of gyration and matches with the previous calculation as shown in this post.

This is the pdf file used in the illustration of this post.

For a link to a calculator for various shapes, please find Moments of Inertia – Reference Table.

This is the next post: Moment of Inertia Iy for Parallelogram.