Last Updated on April 8, 2025 by Maged kamel
Parallel Axis theorem for Iy, Polar Moment of Inertia.
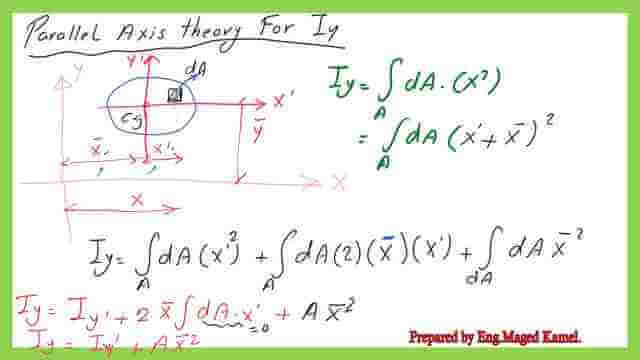
Parallel axes theorem proof for Iy.
We are going to talk about the parallel-axis theorem for Iy. We have again two external axes. x and y. we are going to choose a small Infinitesimal area. We call it, dA, and x ‘ and y are two axes passing by the CG of the area. The distance from the CG to the y-axis is what we call x̅. while the horizontal distance between the CG of the dA, to y’.
The horizontal axis passing by the CG is called x’, and y̅ is the vertical distance from the axis x’ to x.
If we are going to estimate the moment of inertia about this y-axis. The ∫ dA*x^2 distance x distance can be estimated as the sum of two components.
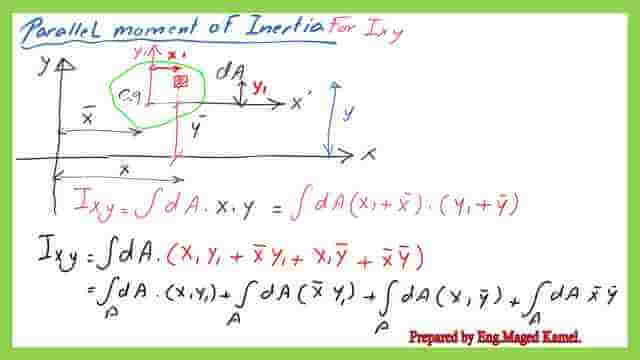
Parallel axes theorem proof for Ixy.
For Ixy, the product of moment of area, again, the same area. We are going to introduce x’ and y’. These are two axes passing by the CG, and the external two axes, as usual, are x and y. The distance from the CG is y̅.
To estimate the product-moment of area, we need to make integration by multiplying dA by x, the horizontal distance * and y, the vertical distance, for both x and y external axes.
Similarly, as before, this x = x̅ + x’ and the y overall distance y̅ + y ‘. We put both items inside brackets, and then Ixy = ∫dA.
Then we multiply by x1* y1+ X1* y̅ + x̅ *y1 + x̅ * y̅.
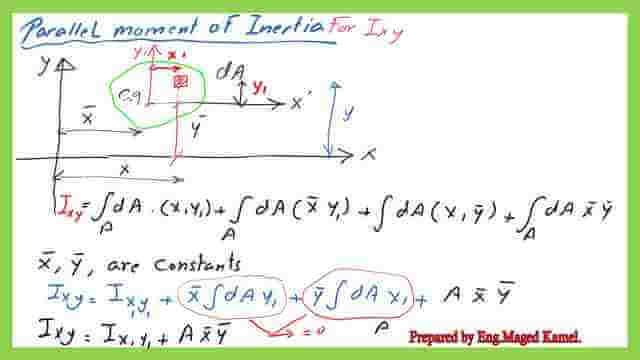
We are going to investigate each item and see what it resembles The ∫ dA*x1*y1=+ ∫ dA* x̅ *y1+ ∫ dA*x1* y̅ + ∫ dA* x̅ *y bar. Since x̅ and y̅ are constant distances, they will come out. dA*x1*y1, this is the product-moment of inertia about the CG., and we are going to call them Ix1y1.
The second term x bar will come out, we are left with dAy1+ y̅ will come out by the ∫ of dAx1, both these two terms, since the first moment of the area about x and y, are equal 0
These axes are passing by the CG. Then, these two terms will be canceled. The last term, which is the ∫ dA* x̅ * y̅, will =A * x̅ * y̅ at the end Ixy=Ix1y1, and the product of inertia about two axes passing by the CG+Area x̅ * y̅.
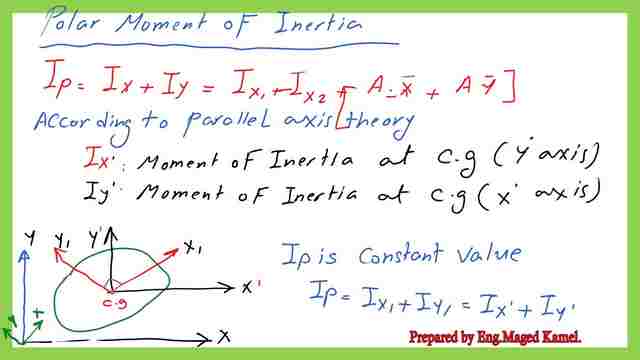
What is the polar moment of inertia Ip?
For the Polar moment of inertia, this is new a new expression. I polar, the moment of inertia is the summation of the moment of inertia about x moment of inertia about y and this will be considered as a constant value.
What does it mean? it means that for any two arbitrary orthogonal, this is supposed to be 90 degrees, the angle should be 90 degrees, at first we x’ and y axes pass by the CG, the I polar=Ix +Iy =Ix’+Iy’.
The moment of inertia, if you are going to discuss the CG, Ix+Iy= Ix1 +Iy1.
We are going to say that I polar =Ix +Iy, which means that again, we are going to write Ix1+A* x̅+Iy2+A* y̅, and if we are going to select any two orthogonal axes passing by the (0,0). We can call them whatever names x & y, X and Y, then the I polar, again, will be = the summation of Ix+Iy.
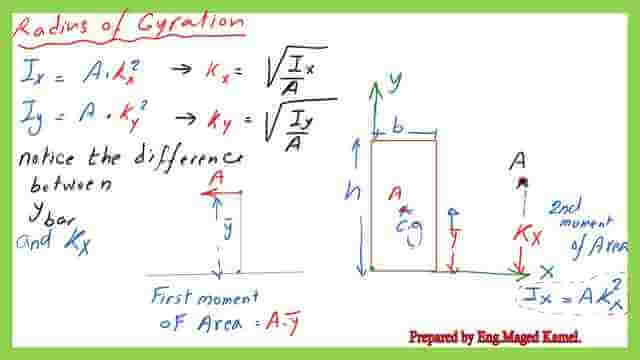
What is the radius of gyration for x and y axes?
There is another term, the radius of gyration for Ix = A* kx^2, so we can get an expression, that kx =sqrt of Ix/A. Similarly, Iy=A*ky^2, which means that ky=sqrt of Iy/A, we will consider that the area, for example, for a rectangle, that area is concentrated at the CG.
We will find out that when the area is concentrated at the center of gravity, then the first moment can be estimated as the multiplication of area by y̅, which is the vertical distance to the x-axis.
The difference between y̅ and k x lies in that the location of the point will be considered changed and the multiplication of the area *kx^2, will produce the second moment of area, that is why the Ix =A *kx^2 after considering that the concentration of area.
This is the PDF used to illustrate this post.
For an external resource, Engineering core courses, the moment of inertia.
This is a link to my playlist on YouTube.
For more details on the radius of gyration, see the wiki.
Next post: Moment of inertia Ix- what are the methods to estimate for the rectangular section?