Last Updated on June 15, 2025 by Maged kamel
Derive the P and F relation as cash flow moment.
How do we derive the present value and future value of a deposit?
We have a timeline from t=0 to t=n, the Timeline in a year. We have a deposit at time t=0 with the present value P value acting downwards. Using the similarity of the Cash flow and moment diagram, we expect to receive future value inflow at t=n, which is represented by an upward arrow (+F) as income.
The present value does not numerically equal the future value; the presence of an exponential function makes the F value greater than P.
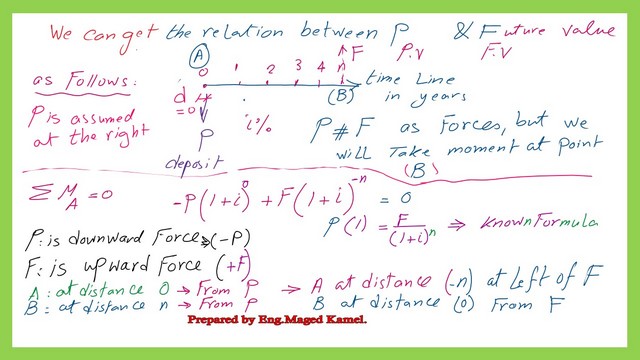
Let us estimate the moment at the pivot point at T=0; we have a moment due to -P, ith arm=(1+i)^0. The pivot point is exactly the same point where the force applies.
The sum of moments at the pivot point at t=0 should equal zero. We have a moment from the present value = (-p)*(1+i) 0, while the moment due to the Future value =+F(1+i)- n since the pivot point is at the left of +F, and the time elapsed is (-n) measured to the left from F.
Considering the sum of moments=0, we have P*(1) because the exponent is set equal to zero, equal to F*(1+i) —n. This can be modified as P=F/(1+i) n, the known relation.
The writing indicates the following points: P is a downward force considered (-P), while F is upward. The pivot point A is at t=0, at a distance =0 from the force (-P). That point is located at the left of F and has a distance =-n.
The pivot point B is at t=n, at a distance =n, from the force (P). The sum of forces in the case of cash flow in the Y- Y-direction does not equal zero, as we will see in the next slide. The P and F relation as cash flow moments is used to get the expression for P.
The pivot point B is at t=n, at a distance =-n from the force (F). We will proceed to the next slide.
How to derive the expression for the Future value of a deposit?
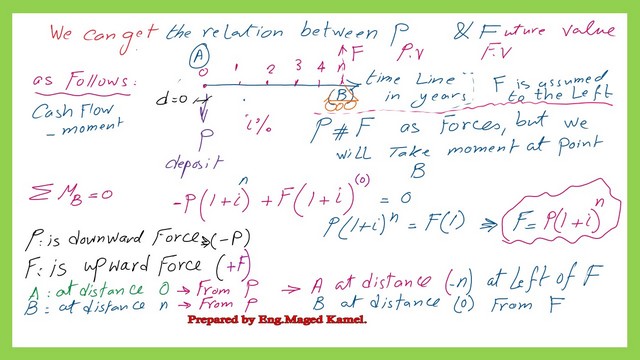
We want to get the same expression between P and F, which is F=P*(1+i)^n, but this time we will apply another approach by
We are considering the Timeline as expressed in years starting fromt=0 to t=n. We will consider our pivot point at b, where t=n.
Let us check the moment due to (-P), which will be the product of (-P)*(1+i)^n, since point B is at the right of (-P). For the moment, due to F, it is +F(1+i)^0; the point coincides with the force (+F). The sum of moments is zero. we will rearrange the terms , -P(1+i)^n +F(1+i)^0=0.
The P and F relation as cash flow moments are used to get the expression for F. The final value of the future value is F=P(1+i)^n. Please refer to the next slide image.
The first solved problem uses cash flow moment.
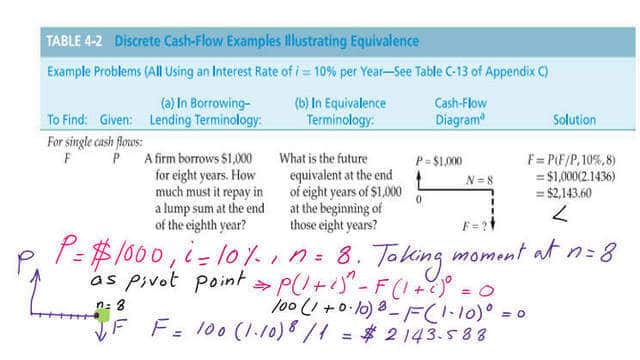
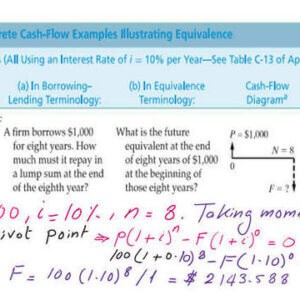
Table 4-2 for the discrete cash flow examples illustrating Equivalence from chapter 4 of William G. Sullivan’s book Engineering Economy. We have an example for a single cash flow diagram F, with the present value P.
The subject can be dealt with in two aspects; the first one is in borrowing ending terminology:
A firm borrows $1000 for eight years. How much must it Repay in a lump sum at the end of the eighth year? But in equivalence terminology, it can be written as What is the future equivalent at the end of eight years of $1000 at the beginning of those eight years?
The borrowed money at t=0 is P of $1000 with an upward arrow. The unknown is the future value, which is F. The given data is the number of years for the loan, n=8 years, and the interest rate i=10%. To get the value of F in terms of P, F=P(F/P, i,n), in that expression P goes with P, so F is equal to F on the right-hand side. Comma i=10% and comma n=8 where n is the number of years.
We will use Table c-13 of interest based on i=10% and get the related F/P, which must be bigger than one. It will be equal to 2.1436. We will multiply F/P by P, and we will get $1000*2.1436=$2143.6.
If we want to get the same result but use the similarity between the cash flow and moment, P=$1000, i=10%,n=8, take the pivot point at t=8 at the right of the upward P, we can write the summation of the moments as 1000*(1+0.10)^8-F(1+0.10)^0=0.
F will move to the right-hand side, with a positive sign, 1000*1.10^8=F*1. This method will give the same value of F by using the calculator, F/P=2.1436. The future value is equal to $2143.588. The slide image shows the four cases for forces and the distance to pivot points.
The second problem was solved using the cash flow moment relation.
A firm wishes to have $2143.6 eight years from now. What amount should be deposited now to provide for it? In this case, if the future value is inflows, the present value is a deposit and will be with -P, downward arrow.
If we consider this example from the equivalence point of view, what is the present Equivalent of $2143.6 received eight years from now?
The interest rate is 10%. We will consider the pivot point at point t=0. Given F=$2143.6, using the known formula P=F(P/F,i,n), i=10%, and n=8. Where F goes with F and P will equal P, comma 10%, comma 8). Using the table of interest for i=10%, we can get the value of P/F.
P/F must be less than 1, and it is equal to 0.4665, then P=$1000. So the man should deposit $100 to get $2143.6 after eight years by an interest of 10%. For the moment estimation, we will take the summation of all the moments about the pivot point t=0, where P is acting downwards (-P) and is unknown.
-P*(1+0.10)^0+F*(1+0.10)^-8=0, can be written as -P*1.0+$2143.6*(1.10)^-8=0. P will move to the other side, equaling 2143.6/1.1^8=$1000. The result matches the estimation that was made using the formula.

Please click on any image, and you will have a slide show. Each photo will appear more transparent.


The Following Links to a helpful reference: Engineering Economy-Applying Theory to Practice
Please refer to this post, Introduction to Economic Equivalence, to review the subject of equivalence.
The next post is 12-Solved problem-Future value for uniform series deposits.