Last Updated on June 7, 2024 by Maged kamel
Solved problem 8-32 for plastic nominal Uniform load.
Solved problem 8-32 for plastic Nominal Uniform load by the lower bound theorem.
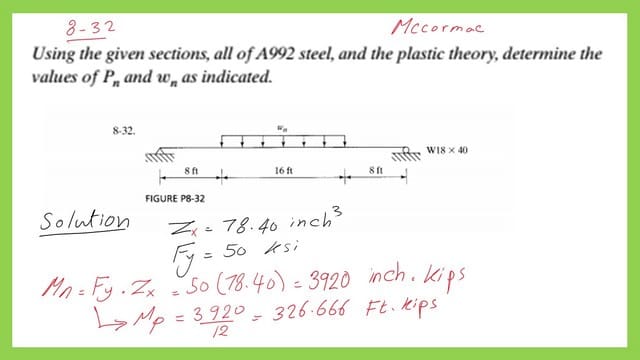
We have a Statically determinate system, a beam that is hinged at one support and has rolled in the other support. It is loaded under a partially uniform load. The uniform load occupies a length of 16′ out of the total span, which isย 32′.
The section of the beam is W18x40 with Fy=50 Ksi. The beam is a statically determinate system.
We have a given section of W18x40. Use a table to get Zx, the plastic section modulus for that section. The Zx value is 78.40 inch3.
The Nominal Mn = Mp = FyZx = 5078.40 inch. Kips, then divide the value of Mp by 12 to convert it into Ft.kips.Mn=Mp=50*78.40/12)=326.66 Ft.kips.
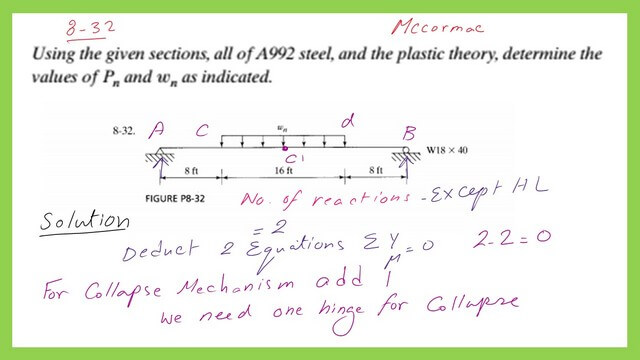
What are the number of hinges required for collapse?
The given beams have two vertical reactions. If we disregard the horizontal load, we will deduct the system of equilibrium equations for โ Y=0 and โM=0. The system is statically determined. We add one hinge to create a mechanism in the middle of the beam.
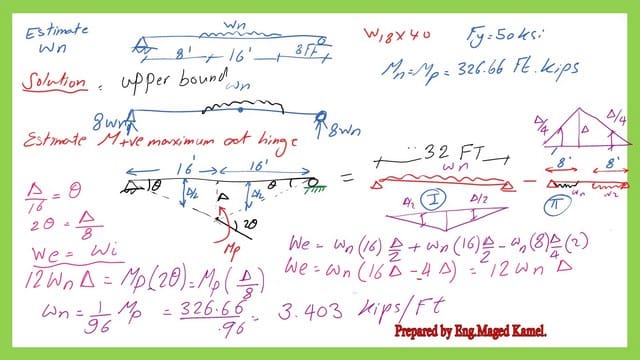
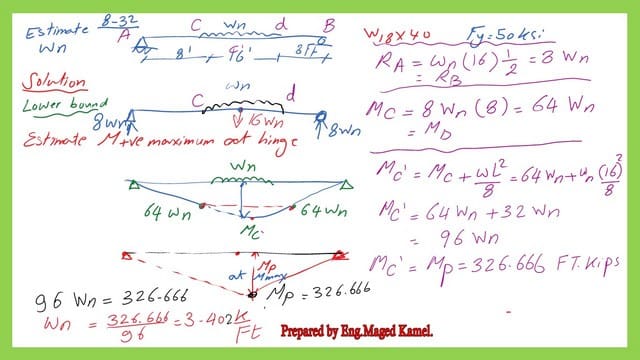
We need to estimate the beam’s positive moment at the plastic hinge at C. To get the value of reactions to estimate the plastic moment, the load weight = Wn*16.
The Reaction at A takes half of this value, RA=0.50*16W=8Wn,ย and the same reaction value RB for support at B.
To estimate the moment point C, we will multiply RA*8. the moment at c=Moment at D=64*Wn.
A parabola of w*(16)^2/8 will be added to get the value of the moment at point C, which is =64*Wn+32*Wn=96*Wn, the maximum positive moment value.
The Mp, the plastic moment at the plastic hingeย =96*Wn, equals 326.66 Ft.kips. The plastic nominal uniform load Wn=Mp/96=326.66/96=3.40 Kips/ft is the value Wn estimated by the lower bound theorem or the statical method.
Solving the example by the upper bound theorem.
Another method of estimating the plastic nominal uniform load is the upper bound theorem or the mechanism method.
Since we have a partially uniform load,ย the external work of the uniform load is the value of this load multiplied by the average deflection underneath the uniform load.
This rule applies if the uniform load occupies the full length of the span; then, the external work will be Wn*0.50*ฮ. However, in this case, a different treatment will be used. At support A, there is a slope due to deflection at point C; due to collapse, the angle at A=ฮธ.
The same angle will be present about the roller support; its value is =ฮธ. The positive Plastic moment at the mid-span will have an angle=2*ฮธ, which is internal work.
The external work can be estimated as the sum of case 1 for the fully loaded span, Wi=0.50*Wn*32*ฮ, minus the external work for the two parts for the uniform loading acting upwards.
The deflection at the edge=ฮ/2, since the distance to the edge/mid span=0.50. The external work for that case =2*Wn*8*(0.50)*ฮ/2.
The total external work for the system=0.50*Wn*32*ฮ-2*Wn*8*(0.50)*ฮ/2=16*Wn*ฮ-4*Wn*ฮ=12*Wn*ฮ.
This is the external work for the system, while for the internal work=Mp*2*ฮธ, where ฮธ=ฮ/16.(12*Wn*ฮ)=Mp*2*(ฮ/16)= Wn, ฮ goes with ฮ, Wn=2*Mp/(16*12)= Mp/96. Wn=Mp/96, since Mp=Mn=326.66 Ft. kips, then wn=326.66/96=3.40 kips/ft.
The value of plastic’s nominal uniform load is 3.40 Kips/ft, which is the same value obtained by using the lower bound method or the static method.
Solving problem 8-32 by MASTAN 2.
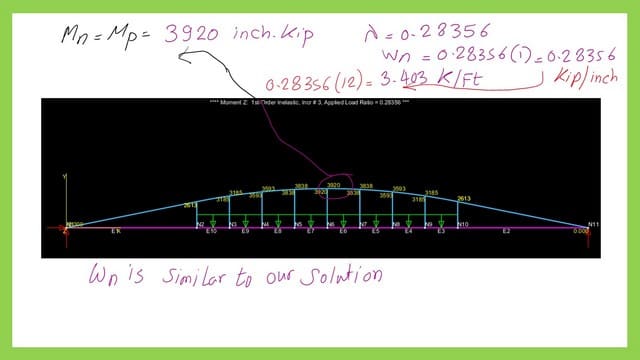
The beam was modeled using MASTAN 2, by the first inelastic analysis, using a uniform load of 1 kip/inch, the node distances are all in inches.
A plastic hinge is located at the mid-span due to a Wn load of 0.284 kip/inch. The Wn value given by the program MASTAN 2 matches our estimated Wn value of 3.402 Kip/ ft.
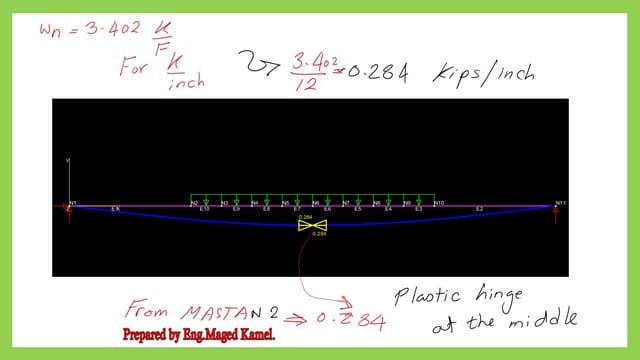
The next slide image shows the moment distribution done by MASTAN 2 for the partially loaded beam. The plastic moment obtained is 3920 inch. kips, which is the same as estimated earlier. The Wn value is 3.403 K/ft.
Have more information about the structural analysis –III.
The next post is Plastic Nominal Uniform load for partially loaded beam.