Last Updated on July 1, 2025 by Maged kamel
Introduction to Roster notation.
Discrete math symbols.
For discrete math, if we look at the NCEES handbook for the FE Exam version. 5.5, we will find Discrete Math subjects. On page 21, the symbols are included. Small x is part of X or a member of X for the subject. The second symbol is for the empty cell or phi. S is a subset of T. The following symbol for S is a proper subset of T. The next symbol is for the empty set, followed by a subset of T.

Roster notation.
We start with Roster notation. From Merriam-Webster, a roster is a list of people or things that belong to a particular group. There are symbols with different shapes.
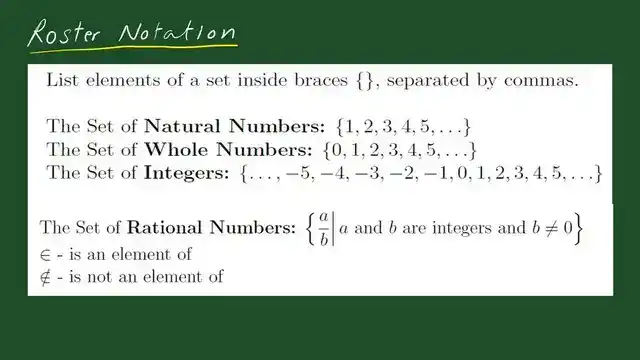
List elements of a set inside braces, separated by a comma. As discussed for the natural numbers, according to Roster notation, it starts with braces at the left and right, enclosed is 1, included in the counting numbers 1,2,3,4,5,…
The previous list is enclosed for the whole number, but we add the 0. We have a continuation or infinity for both the natural and whole numbers.
We have already discussed the expression of Integers: integers are zero, positive, and negative values.
As for the Rational numbers, we have one brace { at the left and at the right, }, these braces include a/b, where a and b belong to Integers. The expression deals with positive or negative.
That is why the definition states that fractions are integers. It is possible to have a zero in the numerator and express it as a/b, where a and b are Integers. Still, the denominators should not be = 0; it is written that b does not = 0 to avoid the expression of infinity.
∈ is an element that belongs to ∉ is not an element or does not belong to it is the same previous symbol ∈ but with an inclined line.
Some samples for Roster notation.
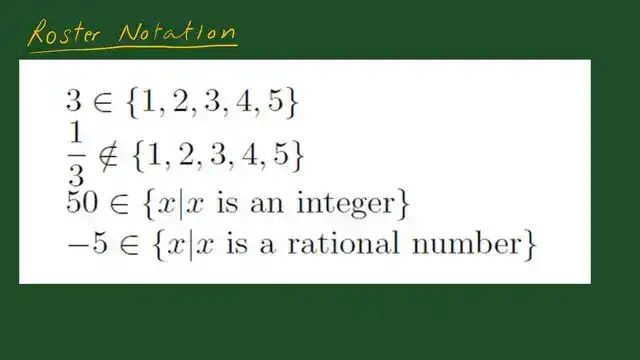
For example, we have 3, which belongs to or is an element of the group between two braces: 1, 2, 3, 4.
It is true that 3 is an element within that group, but 1/3 does not belong to the previous group, 1,2,3,4,5.
Then it is written as ⊄. The number 50 is a part of X, such that, expressed by the symbol |. x is an Integer
The statement is true if x, expressed by the symbol |, which starts at 0 and extends to 50, is a positive non-rational number. It is said that 50 is an element of x, provided that x is an integer.
For -5. Does -5 belong to the family of rational numbers? It is possible, since a rational number may contain -5/1. Any number can be considered rational if the denominator is 1, so -5 belongs to all x values such that x is a rational number.
Solved Examples 1 and 2- for Roster notation.
Let us check example #1. Let G be the set of whole numbers <10. We have explained that a whole number includes 0, and all positive numbers. To write that expression in Notation, we start with the left brace {, we begin by writing 0, then followed by 1,2,3,4,5, and end with 9, followed by the right brace }.
For example #2. Let X is the set of all odd numbers that are <12. The odd numbers are 1,3,5,7,9,11,13. We consider these odd numbers. 0 is not an odd number; it is not included accordingly. We start with the left brace {, we start by writing 1, then followed by 1,3,5, 7, 9,11, followed by the right brace }.

Example 3 for Roster notation.
In example # 3, which of the following set of whole numbers < 10? There are three options, the first one is the Expression of capital c.
It is required to check the set of whole numbers, as discussed, the whole numbers that include,0,1,2,3,4, without 0, without fractions. It is required only for the odd numbers; this is a true selection if it is required for the integers which are<10, but this is ok without writing only odd numbers.
The first choice is not correct. We move to the second selection which is, {0,2,4,6,8}, this list includes all the even numbers< 10, this option is not {1,3,5,7,9}.
This collection has all odd numbers less than 10 and positive Whole numbers.
The fourth choice is not a true selection. The third option is the correct one.

Equality of sets.
The next item is if we have two sets and we want to check whether they are equal or not.
The first set is A= {1,3,5,7}. The first set is B= {3,7,1,5}.
The two sets have the same number of elements; the same numbers are there. Since the arrangement is not important, then A=B. The selection is correct, why?
since all elements of A are the same as the elements in b. Let us check example # 4, with God’s will. Let R all Set of whole numbers <5.
Let S= {4,0,2,3,1}, same numbers of elements, and the next question will be what are the all set of whole numbers<5. The whole numbers start with 0 and continue with 1,2,3,4 and can be represented by R={0,1,2,3,4}.
While for S {4,0,2,3,1}, the same number of elements and the same figures, thus both R and S are equal sets.
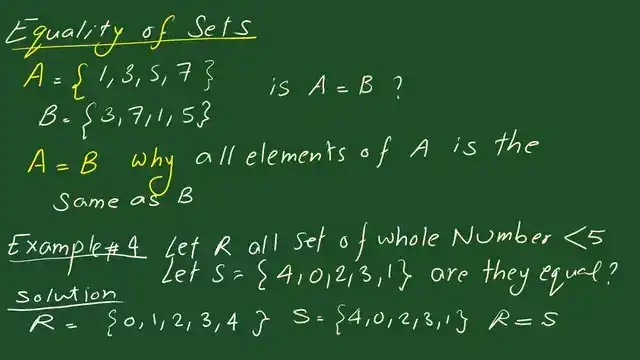
Examine the set, whether finite or infinite.
The following item examines whether the set is finite or infinite. Finite is limited, while infinite is not defined. At the same time, write the set of all Integers.
We write the set as= {…,-2,-1,0,1,2,3,4,….}, the set of integers is infinite since it is continuous from both ends. While for item b), the set of all natural numbers between (0,5), {,1,2,3,4} is an example of a finite set.

Press on any slide to start slide show.






For an external link, please find this link: Venn Diagram.
For the next post, here are the subsets of sets and the Venn diagram.
