Last Updated on November 18, 2023 by Maged kamel

Practice problem 5-8. Find the centroid for the shaded area.

Find the x and Y coordinates of Points A and b in the curved portion.

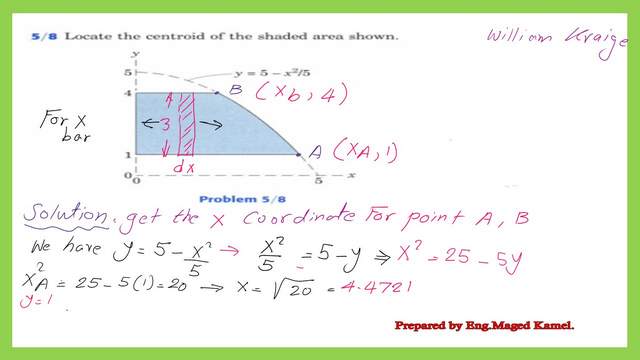
In this post, I will introduce practice problem 5-8. Find the centroid for the shaded area, the practice problem is quoted from Prof.William Kraige‘s book. The shaded area bottom side is at 1 unit from the X-axis and coincides with the Y-axis on the left side.

The shaded area is a composite section which is a rectangular portion with a height of 3 units and a curved portion which has the equation of y=5-(x^2/5).

In order to locate the coordinates of the points on the curve, point A and point B, we have the y value of these points we will substitute by the value of y to get the corresponding X value.

Xa will be found to be equal to the sqrt(20)=4.4721. Please refer to the next slide image for more details.

Xb will be found to be equal to the sqrt(5)=2.23691.

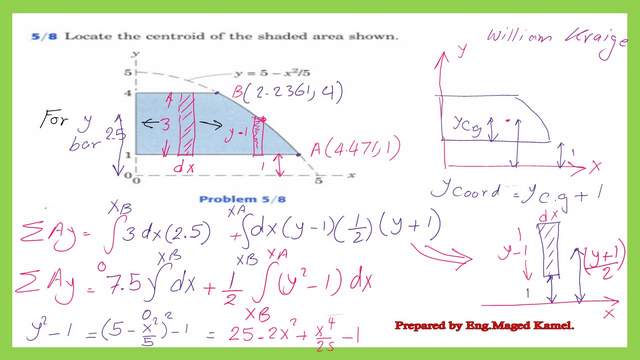
Find the first moment of area about the y-axis for the shaded area.

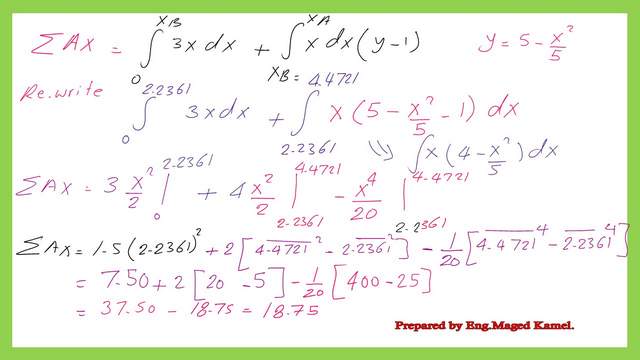
For the first moment of area about the y-axis it is estimated as the sum of the integration of the rectangular strip of width (dx) and height equals 3 from zero to xb multiplied by x and the integration of a strip of width (dx) and height (y-1) from xb to xa multiplied by x.

The height of the second strip is (y-1) since the base of the shaded area is shifted one unit up from the X-axis. For the estimate of the area of the shaded shape, we will integrate a strip of (dx)*3 from zero to xb and add to the integration of a strip of width (dx) and height (y-1). Please refer to the next slide image for more details.

We will perform the integration for the first moment of Area about the Y-axis and substitute by the values of Xa and Xb. The final value of the first moment of area about the Y-axis will be equal to 18.75.

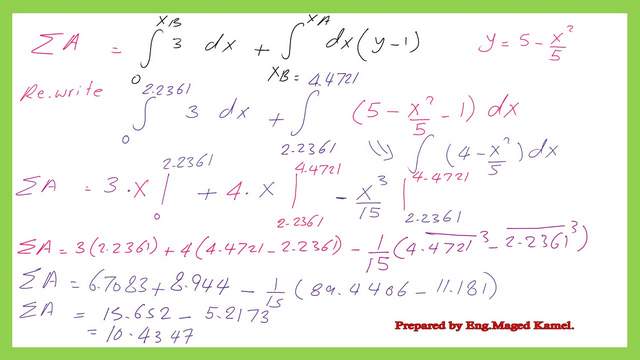
Find the area for the shaded area.

We will proceed to estimate the area of the shaded portion for the two parts, again we will substitute for the values of XA and Xb. For the details of these calculations please refer to the next slide image, the area is equal to 10.4347.

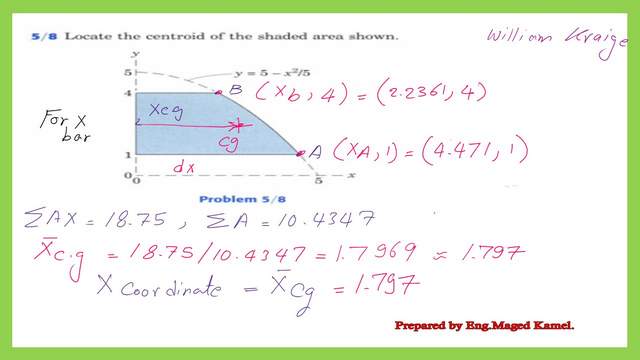
Find the X bar for the shaded area and the x coordinate.

It is easy to find the x bar which is the distance from the Cg of the shaded area to the Y-axis by dividing the value of the first moment of area about the Y-axis to the area. The final value of the x bar is equal to 1.797 which is the value of the x coordinate of the Cg point.

Find the first moment of area about the X-axis for the shaded area.

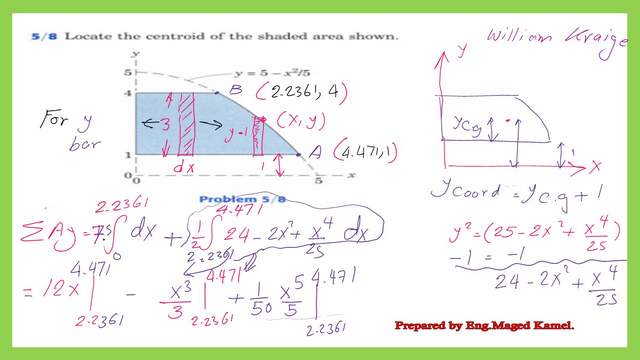
d the integration of a strip of width (dx) and height (y-1) from xb to xa multiplied by (1+y)/2. There is a detailed estimate sketch that shows how to derive the y-bar for the second strip from the X-axis. The details of the estimate of the first moment of area about the x-axis are given in the next three slides in detail.

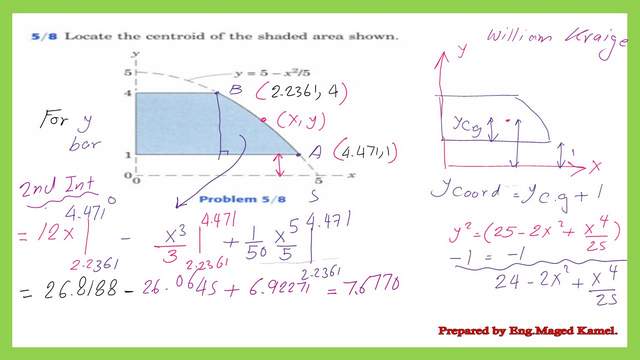
I have considered the second integration to simplify the calculation.

This is the value of the second integration for the first moment of area about the X-axis which is equal to 7.6770.

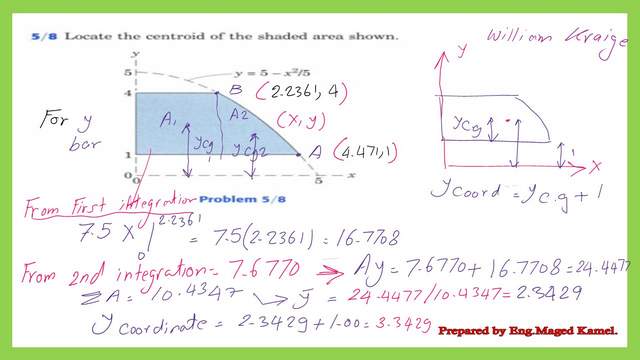
Find the Y-bar for the shaded area and the Y-coordinate.

We add the value of the first moment of area about the X-axis for the first integration for the rectangular portion to the second integration, the final first moment of area about the X-axis is 24.4472.

It is easy to find the Y- bar which is the distance from the Cg of the shaded area to the X-axis by dividing the value of the first moment of area about the X-axis to the area. The final value of the Y-bar is equal to 2.3429. for the y coordinate of the cg add one unit, so the value of the y coordinate will be equal to 3.3429. Thus I have completed the second requirement for Practice problem 5-8. Find the centroid for the shaded area.

This is the previous post. How to find the area and Cg for a circular segment?

This is a link to a very useful site: Engineering Statics open and interactive.