16-A Solved Problem 5- 10: Available Strength for a Slender Column.

Last Updated on September 19, 2026 by Maged kamel

Solved Problem 5- 10: Available Strength for slender column-slender W section.

We will review solved Problem 5-10. From the unified design of the steel structure, for local Buckling, our column section is a W16x26. Kl in the y-direction = 5.00 ft. The solution is based on CM#14, AISC-360-10.

Estimate the available Strength of the column. The column is slender, as shown in the following picture, after verifying the Flange and Web local Buckling parameters.

This Table shows the shapes and corresponding Fy and Fult values.

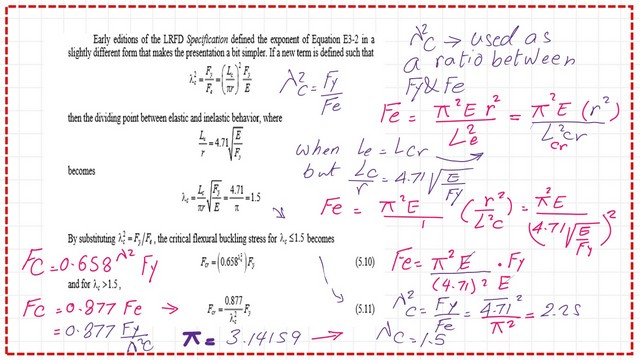
This is the λ2 Expression used in earlier versions, where λ2 = Fy/Fe; Fy is the section yield stress, Fe is the Euler stress, and the Fcr equations are adjusted accordingly for the general Buckling equation.

The following slide image shows the lambda^2 equation.

Solved example 5-10 for available Strength.

This is a newly solved Problem 5-10 from Unified Design of Steel Structures by Louis F. Geshwendener. It requires determining the available Strength of a compression member with a slender Web; the section is a W16x26 column with lcy = 5.0 ft.

Step 1- Check whether the column is long or short using the limiting (kL/r) Value for the solved problem 5-10, estimated from the formula (KL/r)=4.71sqrt(e/FY)=4.70SQRT(29000/50)=113.43.

Step 2: Get the Area of the section, r,y, and the radius of Gyration about y for solved problem 5-10.

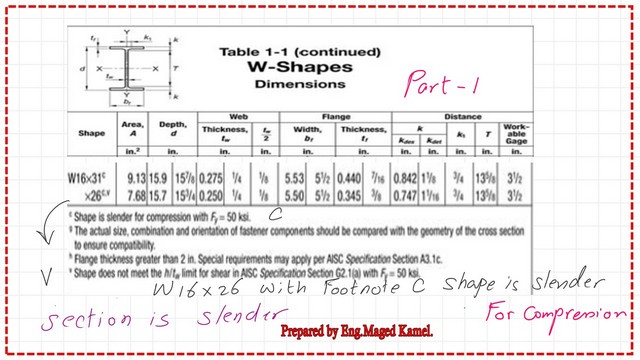
From the relevant Table for W16x26, we get the data for A, ry, d, h, t-web, and (K*l/r) in the y-direction: (1*5*12/1.12)=53.57, which is < 113.43; therefore, the column is short.

The data are in Table 1-1 for the corresponding section. Bf, tf, and tweb for section W16x26 have footnote c, which means it does not meet the h/tw requirement.

Step 3: Check the column, whether elastic or inelastic, for both Flange and Web. From the following image, we get Bf/2tf = 7.97 and h/tw = 56.80.

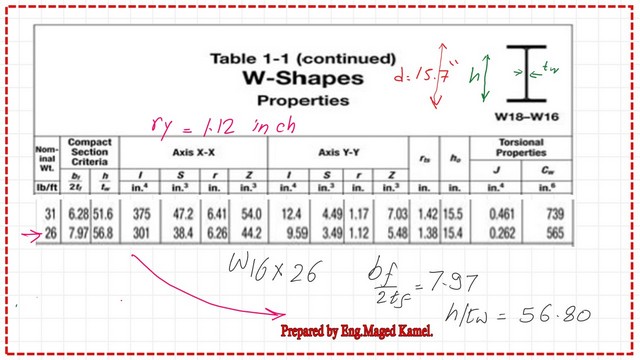
Is a column elastic or inelastic?

We will check against 0.56*sqrt(E/fy), which yields 13.49 > bf/2tf for the Flange in problem 5-10.

While the Value for W16x26(hw/tw) = 56.80 is given in the Table, the limiting Value is 1.49*sqrt(E/fy), which yields 33.72. This Value is less than 56.80, so the section is a slender-web section.

For the Flange, the column section is non-slender since bf/2tf is less than 13.49.

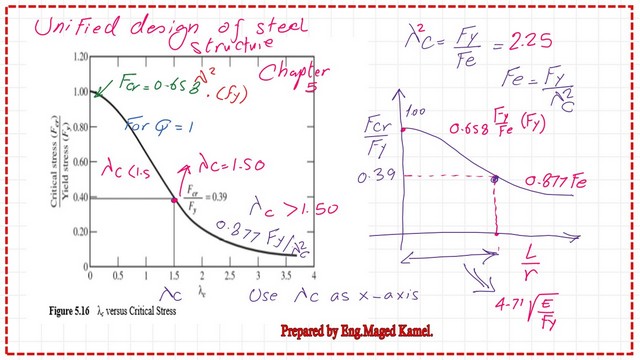
F_cr Value for the column.

Step 4: Estimate λ2, which is Fy/FE, and obtain the relevant Fcr. Consider Q = 1, where Q is the reduction factor; Fe = 99.73 ksi and Fy = 50 ksi.

λ2=(50/99.73)=0.50, we will evaluate Fcr by using the equation fcr=0.658^(λ2Q)(Q*Fy).

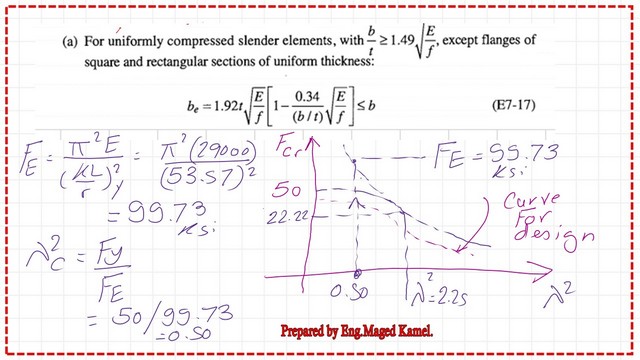
We will evaluate the critical stress, Fcr, using the equation Fcr = 0.658^ (λ^2*Q)(Q*Fy) = 0.658^(0.50)*50 = 40.56 ksi.

The Value of the effective Area.

Step 5: We will estimate the Value of he fromE7-177. According to the AISC equation E7-17, we have Fcr = f = 40.50 ksi. the valu of b/t=h/tw=56.80. We check that he is <h Web; the Value obtained = 10.80″<14.206″.

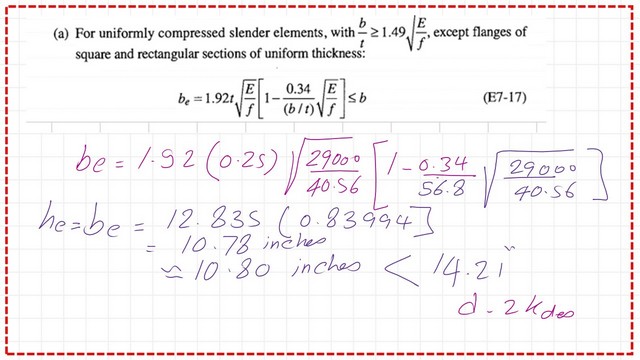
Step 6: We will estimate the Value of A eff after deducting the ineffective Area. The effective Area A eff is obtained from Agross- Aw+(he*tw) = 7.68- 3.55 + 2.7 = 6.83 in2.

The following slide shows the nominal Load equation E7-1; the Area of the column is the gross Area multiplied by the critical Strength.

Estimate the available Strength for LRFD and ASD designs.

Step 7: Use the Value of Aeff and the Fcr to get the LRFD and ASD values. We have an Area of 6.83 in², and the stress is 40.50 ksi.

Estimate the  Strength Value for the column as LRFD Value = Φc*Pn = Φc*For*Ag = 0.90*40.50*7.68

=280.0 kips. For the D Value, Ag*Fcr/Ω = 7.68*40.50/1.67 = 187.0 kips, which was the final result for Problem 5-10 without additional iterations, assuming Q = 1.0.

You can view and download the PDF of this Post from the next document.

This is the Link to the next Post, 16A, Solved Problem 5-10 with more iterations.

For a good A Beginner’s Guide to the Steel Construction Manual, 14^th ed. Chapter – Concentrically Loaded Compression Members.

For a good A Beginner’s Guide to the Steel Construction Manual, 15^th ed. Chapter – Concentrically Loaded Compression Members.

For a good A Beginner’s Guide to the Steel Construction Manual, 16^th ed. Chapter – Concentrically Loaded Compression Members.