Last Updated on June 7, 2024 by Maged kamel
Review Of Shear Stresses for steel beams.
Introduction to shear stress for beams and proof that both vertical and horizontal shear stresses are equal.
If we take two sections at a beam apart by a distance, at elevation =y, there will be two compression forces due to M and M+dM due to the moment difference.
The stress developed from the known formula f=M*y/I will be multiplied by dA, creating two compressive forces. These forces are not equal due to the current difference, which is=dM. To create a balance, a shear force acting horizontally will be created.
As the sum of Fx =0, the shear force is developed to create a balance; this force=shear stress* area=τ*dx*b=σ’*dA-σ*dA, but (σ’-σ)*dA=(dM)*y/Ix, then τ*dx*t=dM*y/I.
How do we derive the expression for shear stress?
Making integration, we get an expression for the stress for shear τ=∫dMydA/(dx*t)=V*Q/I*T, where V is the shear.
Q is the first moment of the area at the point of interest, I is the moment of inertia for the section, and finally,t is the breadth of the section.
Horizontal shear stress for beams.
For the balance of a beam element, the horizontal stress of shear should be accompanied by vertical stress due to shear, as shown in the next slide.
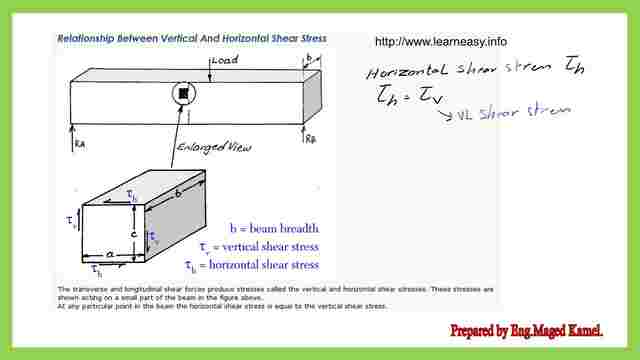
It is proven that the value of τh=τv, where τv is the vertical stress due to shear, while τh is the horizontal stress due to shear by multiplying by area and taking a moment at the edge.
The next post will contain a solved problem for stress estimation for different shapes.
For more detailed shear in bending, please read link-Shear in Bending
This is a link for the next post, A solved problem for shear stress.

