Last Updated on August 14, 2024 by Maged kamel
Solutions for Block Shear-Coped Beam Problem.
This post will introduce solutions for the Block Shear-Coped Beam Problem. A solved problem is quoted from solved problem #5.13 from Prof. Alan Williams‘s handbook.
The solved problem shows how to estimate the block shear-coped beam value when the factor for block shear UBS equals 1.00, which is the case where we have a uniform stress distribution for tension force.
A solved problem for Block Shear-Coped Beam-case-1-UBS=1.00.
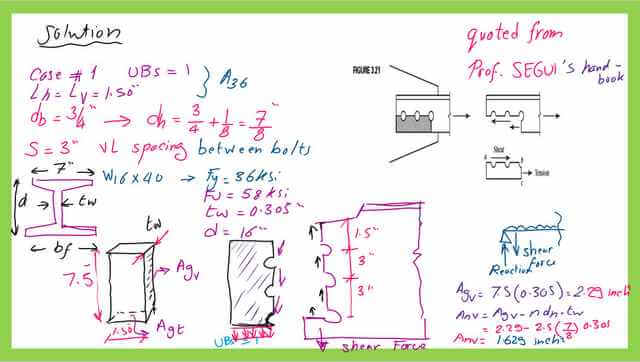
It is required to determine the resistance to block shear of the coped beam W16x40 grade A36.
The horizontal distance between the beam edge and the center line of the first line of bolts is 1.50 inches, and the vertical distance between the edge of the beam to the first line of bolts is 1.5 inches.
The vertical spacing between bolts is 3- inches, as shown in the next slide image. The bolt diameter is 3/4 inches.

Gross and net area for shear-UBs=1.
- For W16x40, the web width is 0.305 inches, the flange breadth is 7 inches, and the flange width is 0.505 inches. The yield stress for A36 is 36 ksi, while the ultimate stress is 58 ksi.
For the hole diameter, we add 1/8 of inches to the bolt diameter, and the hole diameter will be equal to 7/8 inches.
2-One section is subjected to shear, the vertical section through bolts, and one section is subjected to tension, the lower section.
3-We estimate the gross area for the vertical section subjected to shear, which is equal to (7.50×0.305)=2.29 inch2. To get the net area for shear or Anv, we deduct the area for two holes and a half. The net area Anv is equal to 1.625 inch2.
Case of shear yielding and tension rupture.
The slide shows the case of shear yielding, where we have Agv equals 2.288 inch2. The acting shear stress is equal to 0.6*Fy, Since Fy=36 ksi. The shear stress accordingly will be equal to (0.6*36)=21.6 ksi.
Multiply the shear stress by the gross area for shear to get the shear yielding, equal to 49.42 kips.
For the case of tension rupture, first estimate the gross tension area equal to width by web thickness or (1.5*0.305)=0.458 inch2. To get the value of the net area for tension, which we call Ant, we will deduct one hole diameter area. As we can see from the slide, the Ant will be equal to 0.3241 inch2. The Ubs value equals 1. The ultimate stress Fult=58 ksi.

Case of shear rupture and tension rupture.
For the case of shear rupture and tension rupture-LRFD, the block shear-coped beam Phi value equals 0.75. We will multiply (0.60*Fult*Anv) plus (Ubs*Ant*Ful) by the Phi value. The result is equal to 56.46 kips, as shown in the next slide image.
We will select the min value of (51.20,56.42), which can be found to be equal to 51.20 kips, which is the LRFD value for the block shear-coped beam for UBS=1.
For the ASD value, we can find that it is equal to 34.13 kips for shear yielding and tension rupture and 37.64 kips for shear rupture and tension rupture. We will select the minimum value, which is kips, for UBS=1.

Estimate the final LRFD value for block shear.
Now, we are ready to proceed to our estimation for block shear. We have a yield stress of 36 ksi and an ultimate stress of 58 ksi. The gross area for shear, Agv, equals 2.228 inch2. The net area for shear is equal to 0.3241 inch2.
The first case is the case of shear yielding and tension rupture. For that case, the shear stress equals (0.60*Fy), while the tension stress is fult acting on the net area of tension Ant. Please refer to the left side of the slide.
Shear yielding is estimated as equal to 49.42 kips. The tension rupture equals UBs by Fult by Ant; the UBS value equals 1, Ant equals 0.3241 inch2, and the Fult=58 kips. The total value of tension rupture will be equal to 18.798 kips. Adding the value of shear yielding and tension rupture will give a total value of 68.218 kips.
The second case is the case of shear rupture and tension rupture.
Please refer to the right side of the slide. In that case, the shear stress is equal to (0.60*Fult), while the tensile stress is Fult acting on the net area of tension Ant. The shear rupture value is 56.411 kips, and the tensile rupture value is 187.798 kips.
Adding the value of shear rupture and tension rupture will give a total value of 75.028 kips.
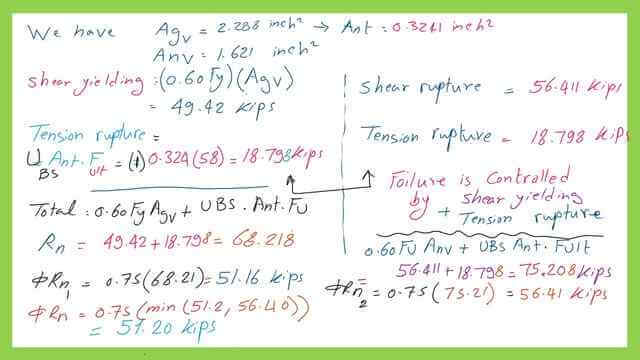
If we want to find the LRFD value for block shear for the first case, we multiply phi, which is equal to 0.75. Please refer to the left side of the slide. Phi will be multiplied by opening a bracket (0.60Fy*Agv plus ( 0.60*fult)*ubs*Ant). The final LRFD value for case 1 equals 51.16 kips.
We will select the minimum values of the two (51.20 and 56.46). The final LRFD value for Block Shear-Coped Beam will equal 51.20 kips.
That means shear yielding and tension rupture controls.
Estimate the final ASD value for block shear.
For the ASD value, consider (1/omega)=(1/2).
We can find that the final ASD value for the block shear*-coped beam will equal 34.13 kips. We have come to the end of the solved problem.

For more details regarding block shear, please refer to the post -*Quickstart to the introduction to block shear resistance.
Our next post will discuss using AISC tables to get the block shear, yielding, block shear rupture, and tension rupture values and how to develop these expressions. Please find the detailed post.
A new post: Post 21-Case 2 for the block Shear-Coped Beam Problem.
For an introduction to coped beams, please refer to the previous post, post 13-The relation between Block shear and coped beams.
For a more detailed illustration of block shear, there is a very useful external link-Tensile yielding and tensile rupture. A Beginner’s Guide to the Steel Construction Manual, 14th ed.
