Last Updated on July 28, 2025 by Maged kamel
Part-2-4 for the Solved Problem 9-9-6, How To Find LL?
The following slide image contains a summary of the content of post 26-steel beam.

Sx for the built-up section.
This is part-2-4 of the solved problem 9.9.6 from Prof. Charles Salmon’s book. We will estimate the elastic section modulus Sx for the built-up section.
The Ix for this section can be estimated for the whole rectangular section. For the Ix, where the x-axis is the major axis, the easiest method to evaluate the Ix for the section is to consider the whole rectangle section (16″x27.25″) and then deduct the two void rectangles of dimensions (7.843″x26″).
The moment of inertia can then be estimated as Ix=(16*27.25^3/12)-(2*7.843*(26)^3/12))=4002.8116 inch4.
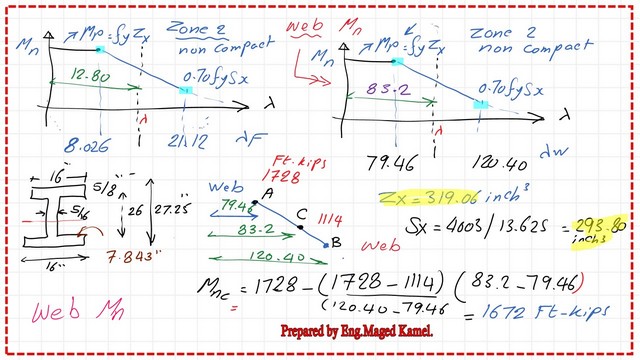
The distance from the N>A to the top of the upper flange Y value =27.25/2=13.625″. the section modulus Ix/y=Sx=(4002.8116/13.625)=293.80 inch3.
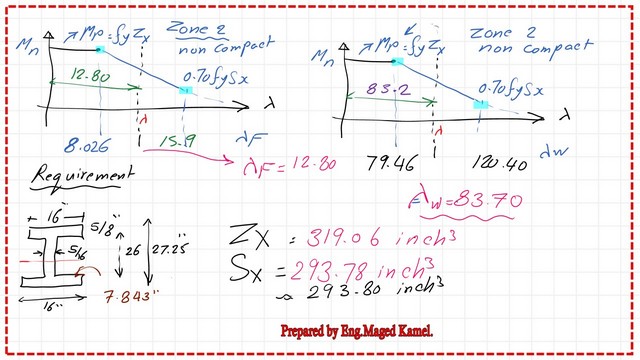
Zx should be > Sx. We have estimated Zx=319.06 inch3, which is > 293.80 inch3. We can proceed to estimate the Nominal moments.
Mn-based on the local buckling of the flange.
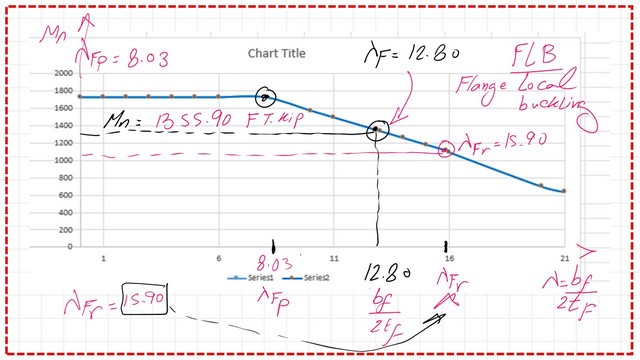
In part-2-4 of the solved problem 9.9.6. Two graphs are drawn: the left-side graph is for the local buckling of the flange, and the values are as follows: the x-axis represents lambda, while the vertical axis represents the nominal moment value.
Both flange and web are non-compact sections; The Mn value will be >0.70 Fy*Sx but less than Fy*Zx.
λp=8.026 For which the Mn=Fy*Zx=65*319.06/12=1728 Ft- kips. λr=15.90 For which the nominal moment Mn=0.70FySx=0.765*293.806/12=1114 Ft- kips. The nominal moment of the flange will have a value between 1728 and 1114 ft-kips.
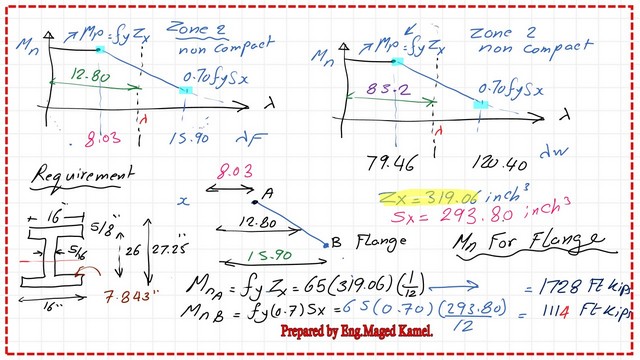
When the factor λflange is equal to 12.80, for which the nominal moment value Mn=1728-(1728-1114)*(12.80-8.026)/(15.90-8.026)=1355.70 Ft-kips, this represents the Mn based on the limit state for the local buckling of the flange.
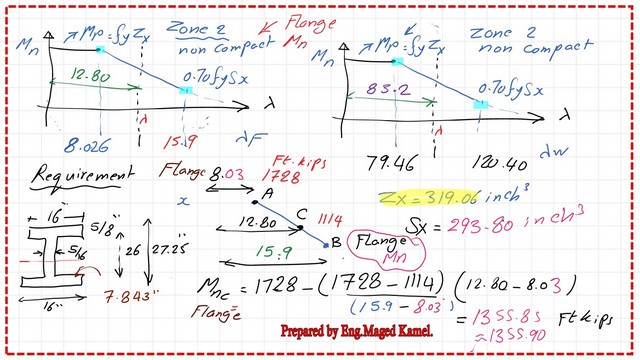
The following slide image contains a table for Mn for the flange using an Excel sheet.
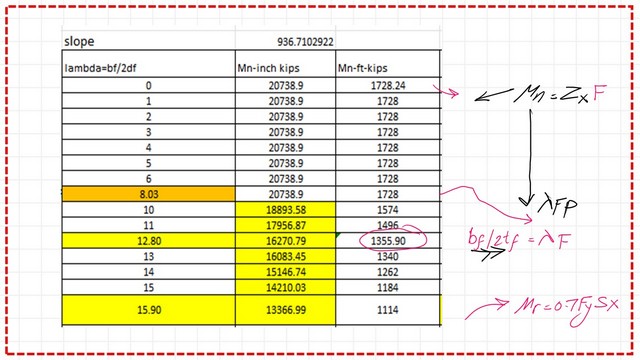
The following slide image contains a graph for Mn for the flange using an Excel sheet.
The nominal moment Mn is based on the local buckling of the Web.
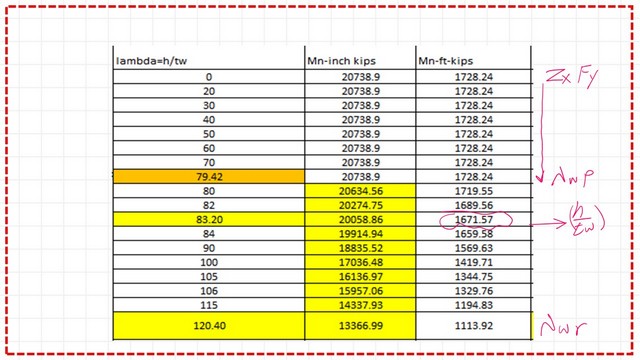
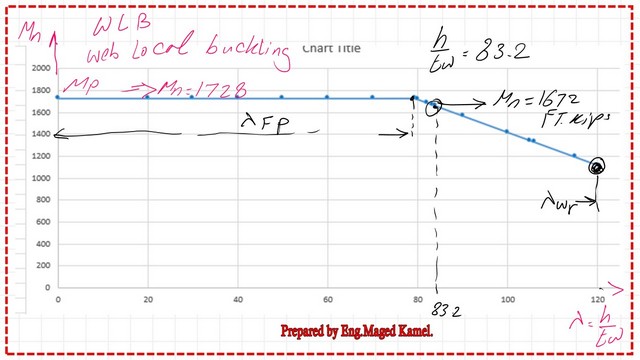
In part-2-4 of the solved problem 9.9.6. Two graphs are drawn from the right-side graph that represents the local buckling for the web, the values are as follows, The plastic lambda value λp=79.46 for which the nominal moment The value of the nominal moment Mn=Fy*Zx=65*319.06/12=1728 Ft- kips.
λr=120.40 For which the Mn=0.70Fy*Sx=0.7*65*293.806/12=1114 Ft- kips.
When the factor λweb=83.20 for which the nominal moment can be estimated as Mn=1728-(1728-1114)*(183.20-79.46)/(120.40-79.46)=1627.0 Ft-kips, this is the Mn based on the limit state for the local buckling of the web. The next slide image shows the detailed calculations for the nominal moment.
The following slide image contains a table for Mn for the web using an Excel sheet.
The following slide image contains a graph for Mn for the web using an Excel sheet.
The next post will be Part 3/4 for Solved Problem 9-9-6. How do you find LL?
This is a very useful external resource for steel beams. Chapter 8 – Bending Member’s Structural Engineering
