Last Updated on April 10, 2023 by Maged kamel
Analysis of portal frame-fixed supports at base.
The Portal frame is analyzed into one frame and two cantilevers.
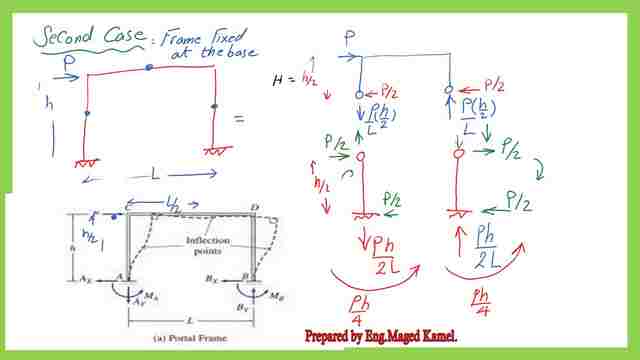
The second case is for the portal Frame when it has a fixed at the base, the inflection points are located at the mid-height of the columns in addition to the hinge at the midpoint of the girder.
The upper part above the hinge is represented separately, there are three hinges. This part is the same as the part which we have already solved, which is a portal frame hinged at the supports, but the only difference lies that the height in this case=h/2.
While the forces at joint derived earlier was= P*h/L at the support at the left and at right also, the same value but opposite direction.
At the left joint P*h/L acts downwards, while at the right support acts upwards, so the joint reaction =P* height/L.
Estimation of the portal frame reactions.
The height from the upper floor to the lower hinge is equal to h/2.
To estimate the left joint Reaction it will be found that is equal to P*h/(2*L) acting downwards, and at the right support, Reaction=P*h/(2*l) acting upwards due to joint balance, there will be P*h/(2*L) force acting downwards for the lower part at the hinge at the left column at height=h/2.
How to draw a moment diagram of portal frame-fixed supports at the base?
We have a horizontal force =P/2 acting to the right and, a vertical force=P*h/L acting upwards. Regarding the bending moment, the moment due to a couple will rotate in this manner, then the support moment to create stability will act in this direction as anti-clockwise, the moment will be=(P/2)*(h/2)=P*h/4.
Let us have a look at the right portion of the joint. We have P/2 at the right hinge acting from right to left, as opposed to a force P/2 from left to right. We have vertical force Ph/2L acting upwards, and opposite force Ph/2L acting downwards.
The couple at the right side =(P/2*h/2), opposed by the moment at the support.
The moment at the left support=Ph/4 acting anti-clockwise which is also the same moment value at the right support of the portal frame-fixed supports at the base.
For the Moment values due to superposition, we have a negative moment, zero at the mid-column height then reversed at joint C, The slope is =0, then the slope starts to increase as the moment increases. Let us have a look at the bending moment diagram and the other forces at joints, but the moment is drawn differently than what we have drawn.
The moment value is P*h/4 and the reaction =P*h/2L as a vertical reaction same as we have evaluated, as we draw the moment as at the tail of the arrow but the same values are identical.
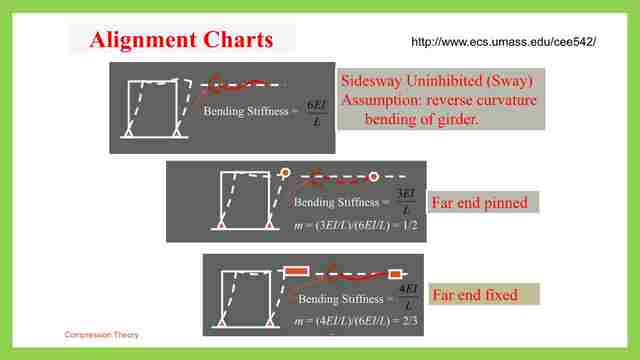
Introduction to m value for alignment chart for the unbraced frame.
The new subject is the modified factor m value From this link UMass, there is useful information for structural steel design, from which I have copied these shapes. I have put the link from the AISC code teaching aids. please download the data and check the compression members
. There is a modification to the alignment chart to be applied for the shown three cases in the next slide image.
We will continue our discussion about m values for the side-sway uninhibited in the next post.
This is the pdf file used in the illustration of this post.
The Core Teaching Aids for Structural Steel Design Courses very use full data that can be downloaded from the AISC link.
This is a link to A very useful external link Concentrically Loaded Compression Members.
This is the next post, a modification to the alignment chart.