Last Updated on September 4, 2024 by Maged kamel

Practice problem-longitudinal welds of a plate section.

This is a Practice problem for the longitudinal weld of a plate section. This is case 4 for the shear lag factor table D3.1-CM-14. It can also be considered case 4a based on CM-15 and related specification -2016.

In this post, we will solve two solutions: the first is based on CM-14, and the second is based on CM-15.
A given 3/8″x5 ” plate section Of A36 steel with two longitudinal welds, as shown in Figure P3-34, is required to find The LRFD and ASD values neglecting the block shear.

The welded area equals 3.75 inch2; please refer to the next slide image for more details.

Estimate the shear lag factor U based on CM-14.

As we can see from the next picture, table D3.1 shows shear lag factors for connections to tension members. For longitudinal welds, it is case 4. Here, it is only for plates where the tension load is transmitted by longitudinal welds. The shear lag factor depends on the width of the joint w and establishes a relation between the length of connection L and width W.

1-From the given Data for the ASTMA36, we can get the yield stress Fy=36 ksi and the ultimate stress Fult equal to 58 ksi.

From the given section of PL3/8″x5″, the width of plate w=5 inches, we cannot estimate the plate’s minimum shear lag factor by diving the area of the connected area of the whole area since it is not allowed by the code for plates.

2- The connection length is given by the given section as equal to 5 inches.

3- From the given section of PL3/8×5, the width of plate w=5 inches .

4- There are three cases based on the relation between L and W for the value of shear lag factor U. For this practice problem, we have L=W, and the U value will equal 0.75.

The equations that we use for the LRFD and ASD designs.

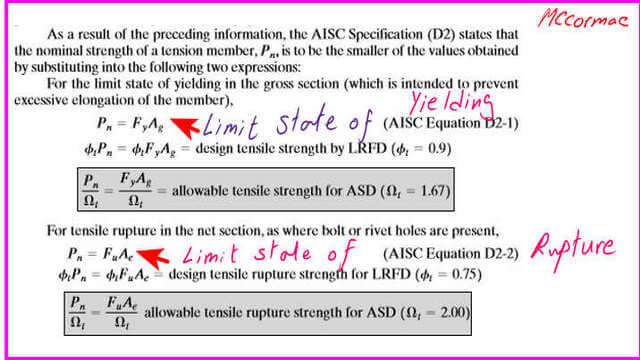
Effective area based on CM-14.

The net area of the -longitudinal weld of a plate section is equal to the gross area of the plate, which is (width by thickness)=5×3/8=1.875 inch2.

The effective area can be found by multiplying the U*net area, which equals 1.406 inch2.

Estimating the Nominal strength for Practice problem-longitudinal weld of a plate section.

We have two cases for the nominal strength, the first case is the case of tensile yielding for which we consider the area as the full area and the tensile strength is equal to yield stress multiplied by the area and can be found as (1.875*36)=73.125 kips.

The second case for tensile strength is rupture strength, in which the value is found to be equal to the effective area multiplied by the ultimate stress And can be found as (1.406*58)=81.55 kips.

LRFD strength for Practice problem-longitudinal weld of a plate section.

Multiply the first value of the strength due to yield by the reduction value of φ equal to 0 .90; we can get the LRFD strength due to yielding as equals 60.75 kips.

When we multiply the second value of the strength due to rupture by the reduction value of φ, which is equal to 0.75, we can get the LRFD strength due to rupture, which is equal to 61.16 kips.

We will select the lesser value as our final LRFD strength, which equals 60.75 kips, indicating that the LRFD strength is governed by yielding.

ASD strength for Practice problem-longitudinal weld of a plate section.

Multiply the first value of the strength due to yield by the reduction value (1/Ω) equal to (1/1.67); we can get the ASD strength due to yielding as equals 40.42 kips.

Multiply the second value of the strength due to rupture by the reduction value (1/Ω) equal to (1/2), and we can get the ASD strength due to yielding as equal to 40.78 kips.

We will select the lesser value as our final ASD strength, which equals 40.42 kips. This indicates that the ASD strength is governed by yielding.

Estimate the shear lag factor U based on CM-15.

Referring to Table D3.1 for shear lag factor based on CM-15. The item number for shear lag factor U for the longitudinal weld to a plate is termed 4a. It includes other structural items that also have longitudinal weld unlink, the previous version of table D3.1 for Cm-14.

The U value is estimated by the product of (3L^2/3l^2*w2) multiplied by (1-1- x̅/ L). The second term is set equal to 1 for plates.

1-From the given Data for the ASTMA36, we can get the yield stress Fy=36 ksi and the ultimate stress Fult equal to 58 ksi.

From the given section of PL3/8×5, the width of plate w=5 inches.

1- The connection length is given by the given section as equal to 5 inches.

2- From the given section of PL3/8×5, the width of plate w=5 inches .

3- The shear lag factor U equals(3*5^2/(3*5^2+5^2)=0.75 which is the same value as estimated by CM-15.

The estimation of the effective area-CM-15.

The net area of the -longitudinal weld of a plate section is equal to the gross area of the plate, which is (width by thickness)=5×3/8=1.875 inch2.

The effective area can be found by multiplying the U*net area, which is equal to 1.406 inch2. The gross area is equal to 1.875 inch2.

LRFD strength for Practice problem-longitudinal weld of a plate section.

Multiply (0.90*36*1.875), the LRFD strength due to yielding equals 65.75 kips.

Multiply (0.75*58*1.406), the LRFD strength due to due to rupture equals 61.16 kips.

We will select the lesser value as our final LRFD strength, which equals 60.75 kips. This indicates that the LRFD strength is governed by rupture.

ASD strength for Practice problem-longitudinal weld of a plate section.

From the estimated calculation the selected ASD value is 40.42 kips, the full data is shown in the next slide image.

The next post is 9c- Practice problem-longitudinal weld of a c-section.

Chapter 3 – Tension Members– A Beginner’s Guide to Structural Engineering is a great external resource.