Last Updated on July 4, 2025 by Maged kamel
Cash flow in and out diagram.
The difference between the cash flow in and out diagram.
The cash inflows are the sources of cash, including receipts, revenues, Incomes, and savings generated by project and business activities.
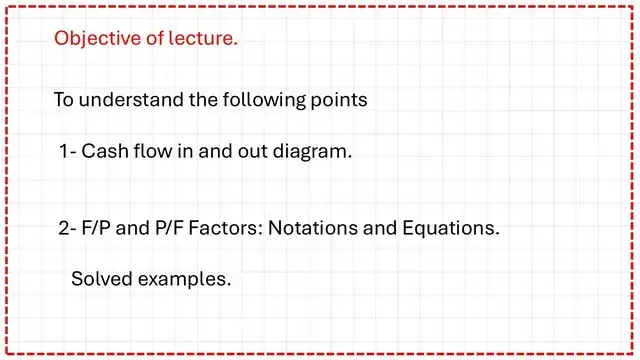
The inflows are represented by a plus or positive sign(+), which is why it is called cash inflows.
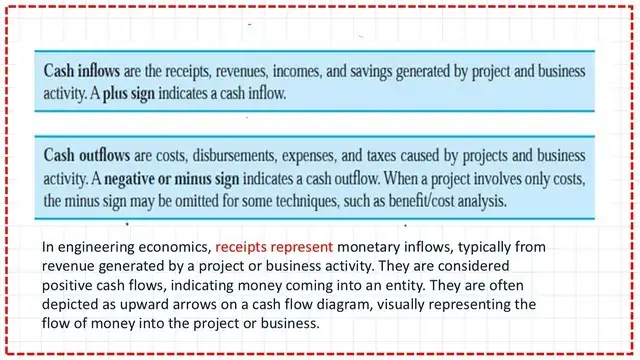
While cash outflows are the sources for cash out, such as costs. An amount that must be paid or spent to buy or obtain something. While disbursements refer to payments made from a loan, expenses denote the costs incurred for a particular purpose.
A compulsory contribution to state revenue.
The cash outflows are the sources of cash out, such as costs, which are amounts that have to be paid or spent to buy or obtain something. While disbursements are the payments from a loan, expenses are the costs required for something or taxes, which is a compulsory contribution to state revenue.
Projects and business activities cause cash outflows. The outflows are represented by a negative sign (-), indicating cash outflows.
When a project involves only costs, the minus sign may be omitted for specific techniques, such as benefit-cost analysis.

An example of a cash flow in and out diagram.
This is an example of a cash flow in and out diagram, for 5 years, the X-axis represents the time scale. The axis is divided into five equal spaces from 0 to 5.
The interval from 0 to 1 represents the first year period, from 1 to 2 represents the second year, from 2 to 3 represents the third year, from 3 to 4 represents the fourth year, and from 4 to 5 represents the fifth year.
What matters is that the cash flow is the plus sign (+) for cash in and the minus sign (-) for money out.
This is shown on the vertical axis. At time t = 0, the present is considered, and t = 1 represents the end of the period, one year later. We assume that the periods are in years for now.
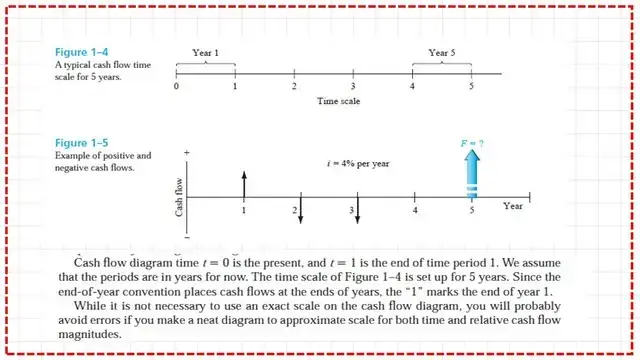
The time scale is set for 5 years, since the end of the year, the convention places cash flows at the end of a year, the “1” marks the end of year 1, as shown in this cash flow at the end of the first year there is money received represented by an upward arrow.
At the end of the second year, payment is made, or cash-out or outflows or expenses, and there is an arrow pointing downwards.
At the end of the third year, there are expenses as well represented by an arrow pointing downwards, it is expected to have cash-in at the end of the fifth year for which the income is unknown.
There is an example: you borrowed $8500 at time=0, first, we draw the scale, but check the scale units for cash flow in and out of the diagram and the total time duration, we have time in weeks, we have time 0 and two times intervals 1 and 2, the x-axis title is written weeks.
The income is represented by the(+) sign, and the expenses or outflows by the minus(-) sign.
You borrowed $8500 at time t=0, the borrowed money is considered as cash-in, and an upward arrow is drawn at t=0 or now, after one week or t=1, he bought a carby $8000, which is expressed as -$8000, since it is cash-out and is drawn with a downward arrow.
A paint job is done at t=2, with expenses of $ 500. A negative sign is placed since this represents an expense, and a downward arrow is drawn at t=2. The arrow is drawn with a small scale to represent the $500. This is the sketch from the borrower’s point of view.
For the different perspectives, the value and time are shown as follows: In the case of the bank, a loan of $ 8,500 was given, which will be represented by the (-) sign in the bank’s cash-in and cash-out diagram.
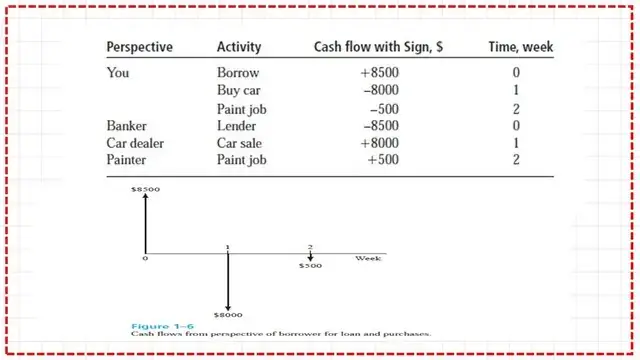
The car dealer, cash-in and cash-out, has an income of $8000; after selling a car, which is cash-in at t=1, this income will have a (+) sign.
For the painter, the cash-in and cash-out transactions resulted in a $500 receipt, as cash-in represents an income or inflow, and has a (+) sign at t=2.
A solved example 1.10 for Cash flow in and out diagram.
This is an example from Prof. Anthony Tarquin’s book, Engineering Economy, how to draw a cash flow in and out diagram.
Example 1.10 – An electrical engineer wants to deposit an amount P now such that she can withdraw an equal amount of A1, $2000 per year, for the first 5 years, starting 1 year after the deposit, and a different annual withdrawal of A2, $3000 per year, for the following 3 years. How would the cash-flow diagram appear if i =8.5% per year?
The solution can be done through these steps:
1- Draw a timeline and divide it into eight equal intervals.
2- She deposited at t=0 is represented by a(-) sign since she withdraws money from the other resource. For the cash flow in and out diagram, the Present value of P is to be estimated and is represented by a downward arrow; it is called P. 3.
She can withdraw an equal annual A1=$2000 for the next 5 years; we draw an equal five arrows at t=1, t=2, t=3, t=4, and at t=5 at the x-axis for time intervals in years

The arrows are drawn as upward arrows.
4-She can withdraw A2=$3000, which are drawn as an equal arrow at t=6, t=7, and t=8.
Each arrow is an income and is drawn in the (+) direction in the cash flow in and out diagram at the x-axis for time intervals in years. The arrows are drawn as upward arrows, and the arrow height for the last three years is longer as compared to the arrows for A1 since the A2 value is larger. The cash flow in and out diagram is completed.
Terminology and symbols.
The equations and procedure of the engineering economy utilize the following terms and symbols to make these symbols unique and universal; sample units are:
P: The value of the amount of money at the time designated at the present or time 0.
F: the value of money or amount of money in the future.
Also, F is called the future worth, or future value(FV) in dollars.
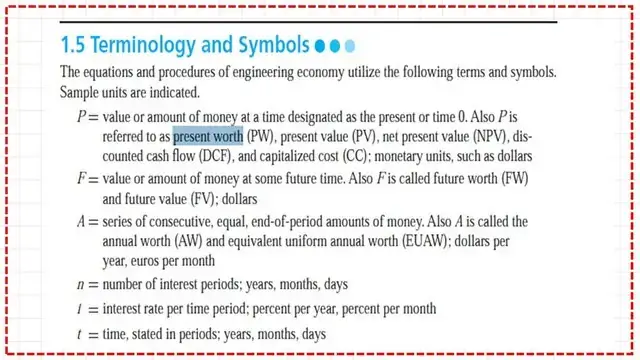
A is a series of consecutive, equal, and end-of-period amounts of money. It is also called annual worth (AW) or equivalent uniform annual worth (EUAW). n : number of interest periods, months, days.
I : interest rate per period; per year, assumed per year unless otherwise mentioned else, or percent per month. t: time, stated in periods: years, months, days.
Economic factors.
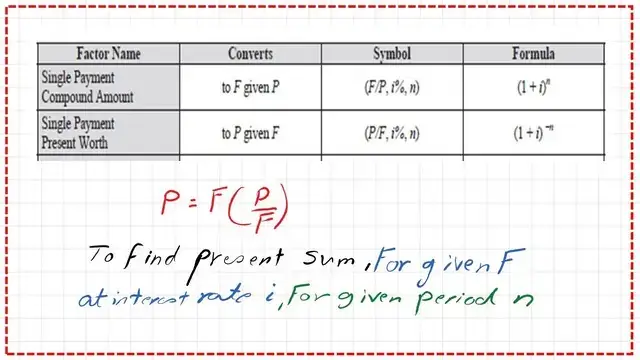
As if creating an equation, if we need F, then we equate it to P(F/P), where P is given in the numerator and the denominator P cancels each other out.
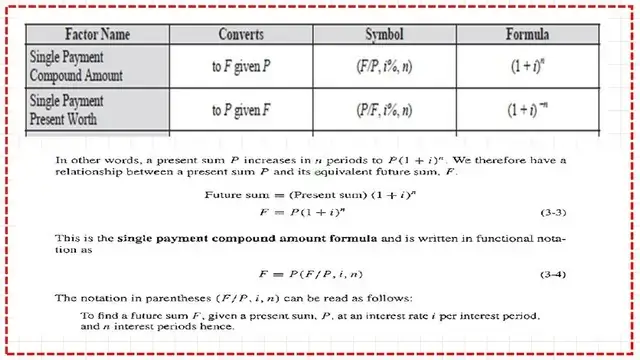
Then, F on the left-hand side and F on the right-hand side.
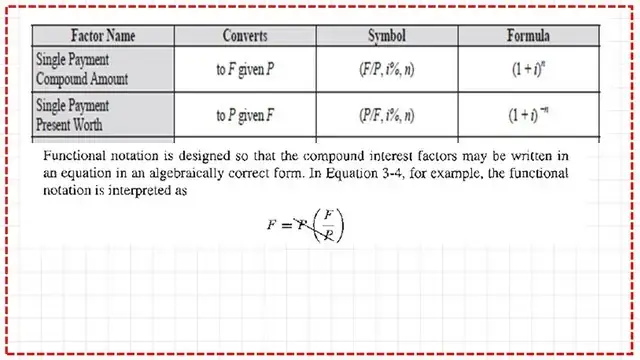
However, if we want the P, the present value as a function of the given F, then this can be expressed as P = F (P/F). If the F value is given, i% and n are also provided.
For a valuable external resource, Engineering Economy, click the link: A good reference.
This is a link to the next post, What is MARR?
