Introduction to design of continuous beam, solved problem 4-15.
This is the summary of the content of this lecture.
This is a table to remind us of the corresponding Fy and Fult for the different ASTM categories.
Detailed illustration for the design of continuous beam based on LRFD.
The following steps will be followed to Design a continuous beam.
1-Estimate The Ultimate load for which we have the maximum value of 1.20 Wd+1.60WL or 1.40 Wd for the given two-span continuous beam.
a-For Wult=1.20*1+1.60*3=1.20+4.80=6.00 kips/ ft, the other possibility of 1.40Wd=1.40*1=1.30 kips/ ft, The maximum value for Wult selected will beWult=6.00 kips/ft.
2-Estimate the maximum M+ve and M-ve values, We have from statics that M-ve= Wult*L^2/8 where L is the span length. While for M+ve value =0.07*w*L^2/and the ultimate positive and negative moments as M+ve =wul*L^2*0.07for the LRFD design.
3-Then M-ve=6*20^2/8=300 ft.kips. As for M+ve=0.07*6*20^2=168 ft.kips.
4-Since the given beam is a continuous beam, perform a reduction of negative moment Mult-ve=0.90*Mult, this value will be the final value of Mult -ve, then add the average of this value to Mult +ve, to get the final Mult+ve.
The M-ve for design=0.90300=270.0 Ft-kips is based on the LRFD design. As for M+ve final=168+0.100.5*300=(168+15)=183.0 ft.kips
5-Select the maximum value of Mult+ve and the Mult -ve, consider this maximum for the solved problem 4-15, Mult=0.90*Zx*fy, hence the value of Zx can be estimated as Zx=Mult/(0.90*fy), we have Fy=50.0 KSI and Mult=270.0 ft. kips.
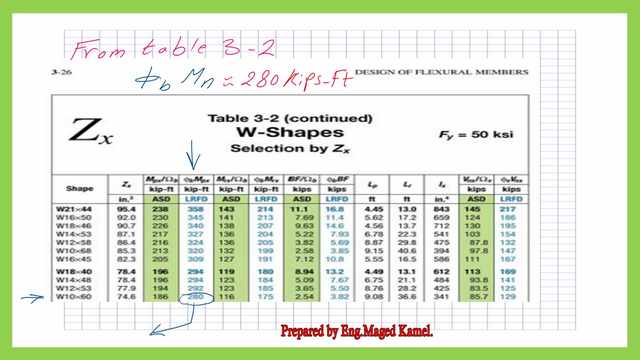
The value of Zx is 270*12/(0.90*50)=72.0 inch3. 6-From table 3-2 where the W sections are arranged and sorted based on Zx, based on the author’s requirements of selecting the lightest W10 section.
We can select the w10x60 which will give Zx value as =74.60 inch3>72.0 inch3 as required by the estimation.
7- Check the compactness of the selected section as shown in the next slide image if we wish to calculate manually, then check whether the section is compact by estimating λf and λweb is less than the criteria given by the AISC specifications.
We need to go to table 1-1 to find the data for bf and d and he and tw, or we can get the compactness ratios directly from the table.
We can get the same result for the factored moment from Table 1-1 for section W10x 60, please refer to the next slide image.
8-Estimate the final (φ)Mn, when having φ=0.90, while Mn=FyZx=5074.60=310.83 ft.kips, then finally/12=(φ)Mn=(0.90)*310.83=279.75. 18ft. kips approximated to 280.0 ft.kips.
We can use Table 3-2 to verify our previous calculations for the LRFD design.

Detailed illustration for the design of continuous steel beam-based on ASD.
1-Estimate The total load for which we have the maximum value of Wd+WL for the given two-span continuous beam.
a-For Wt=1+3=4.0 kips/ ft .
2-Estimate the maximum M+ve and M-ve values, We have from statics that M-ve= Wt*L^2/8 where L is the span length. While for M+ve value =0.07*wt*L^2/and the ultimate positive and negative moments as M+ve =wt*L^2*0.07for the ASD design.
3-Then M-ve=4*20^2/8=200 ft.kips. As for M+ve=0.07*4*20^2=112 ft.kips.
4-Since the given beam is continuous, perform a reduction of negative moment M-ve=0.90*Mt, this value will be the final value of Mt -ve, then add the average of this value to Mt +ve, to get the final Mt+ve.
The M-ve for design=0.90200=180.0 Ft-kips based on the ASD design. As for M+ve final=112+0.100.5*200=(112+10=122.0 ft.kips.
5-Select the maximum value of Mt+ve and the Mt -ve, consider this maximum for the solved problem 4-15, Mt=(1/1.67)*Zx*fy, hence the value of Zx can be estimated as Zx=1.67 Mt/(Fy), we have Fy=50.0 KSI and Mt=200.0 ft. kips, then Zx value=(1.67*200)*12/(50)=72.0 inch3.
6-From table 3-2 where the W sections are arranged and sorted based on Zx, based on the author’s requirements of selecting the lightest W10 section. We can select the w10x60 which will give Zx value as =74.60 inch3>72.0 inch3 as required by the estimation.
8-Estimate the final (1/Ω)*Mn, when having Ω=1.67, while Mn=Fy*Zx=50*74.60=310.83 ft.kips, then finally/12=(1/Ω)*Mn=(1/1.67)*310.83=186.18 ft.kips. This is the last step for the design of a continuous steel beam based on The ASD design.
We can get the same result for the factored moment from table 3-2 for section W10 x 60,, Based on the ASD. please refer to the next slide image.
This is the pdf file used in the illustration of this post.
This is the next post, Collapse Load for A Simply Supported Beam.
A Beginner’s Guide to Structural Engineering.

