Last Updated on July 29, 2025 by Maged kamel
Part 4/4 of the Solved Problem 9-9-6, How To Find LL?
The following slide image illustrates the content of post 28.

How to estimate the Cb for the beam-Part 4/4 of the Solved Problem 9-9-6.
The BC value for part AB and CD.
Cb for a simply supported beam under uniformly distributed, this is included in part 4/4 of the Solved Problem 9-9-6. This is a reminder of the Cb value for parts AB and CD—the value of Cb=1.46.

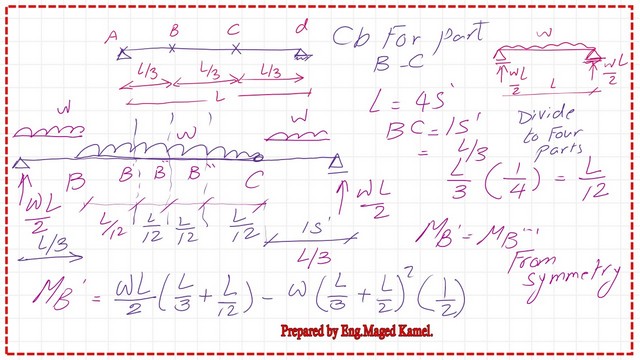
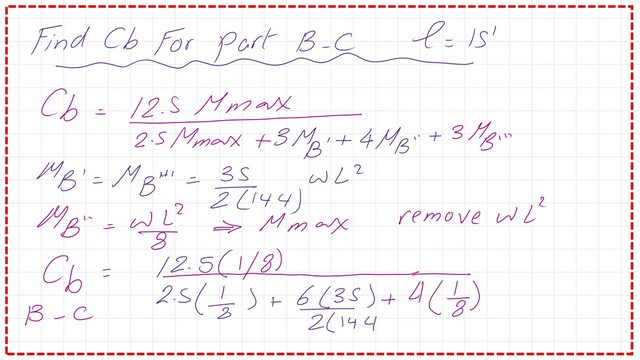
Find the BC value for part BC.
We have one roller support at one end and a hinged one at the other. The bracing spacing is every L/3 distance, where L is the span length. We will take the first part of L/3 and magnify it. This is part BC.
The reaction for the beam at the left hinge = W*L/2=Reaction at the right support.
We need to estimate the moment values at B’, B”, b”‘, and C. The L/3 length is to be divided into four sections. So we have L/12 intervals between these points. Then, we can estimate the CB equation. CB=(12.50*M-max)/(2.50 M-max+3*Mb’+4*MB”+3*Mc’)
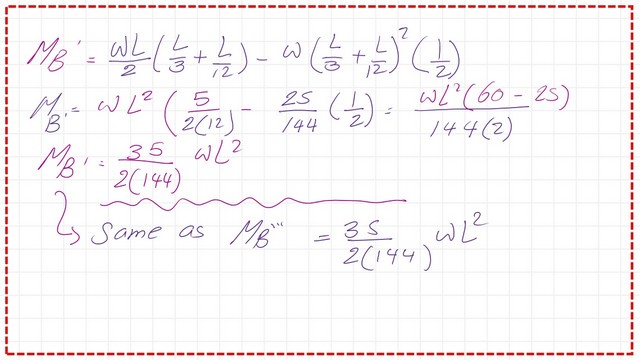
The next slide image shows the value of the moment at point B’, which has a length of 5L/12 from the left support.
This is the final value of the moment at Point B’, which is the same value for point B”‘.
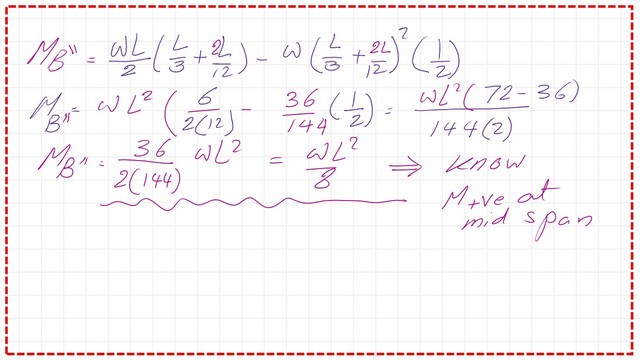
The following slide shows a detailed estimate for the moment value at point B”, which has a distance of L/2 from left support A.

This is the final value of the moment at Point B”, which is equal to W*L^2/8.
The next slide shows the detailed calculation for the Cb value for part Bc.
From the next slide. Use the equation of CB is equal to (12.50*M-max)/(2.50 M-max+3*Mb’+4*MB”+3*MB”‘). The maximum value is W*L^2/8.
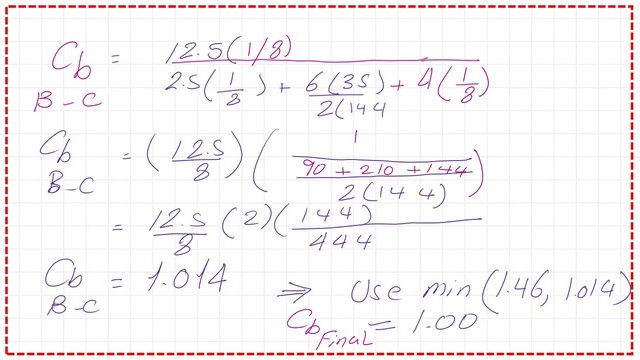
The value of Cb=12.50/8*(90+210+144)/288=1.01, the beam has three values of Cb as (1.46,1.01,1.46), the CB can be used to increase the Mn, the selected value is 1.00.

How to estimate the final Mn for the beam?
The next step is to estimate the final value of the Mn value based on the limit state of the lateral-torsional buckling.
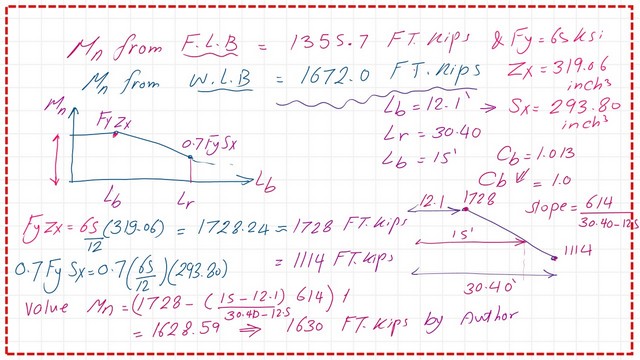
We have Lp =12.10′ while Lr = 30.41′, and the actual bracing length is 15′, as given in the solved problem. We have Mp=1728 at Lb=12.10 and Mr=0.70*Fy*Sx=1114 at Lb=Lr.
At the 15′ distance Mn=Cb* (1728-1728* (1728-1114)/(30.41-12.10)*(15-12.10), if we so we can use cb as=1.01, taken as =1.0 by prof. Salmon.
The next slide shows a graph of Lb and Mn values—at Lp=12′, Mn=1728 ft, while for Lr=30.41′, Mn=1114 ft. kips.
The Mn value for cb=1.00 will equal 1630 ft. kips for lateral torsional buckling. The slide shows the three values. This is a reminder about the Mn in the case of local flange buckling.
This is a reminder of the Mn from the flange and web local buckling case.
We have three Mn values: one from the local buckling of the flange, which is equal to 1355.7 ft-kips; the second from the local buckling of the web, which is 1672 Ft-kips; and the third, 1630 ft. kips.
The minimum selected value of Mn is 1355.70 ft-kips.
The LRFD value multiplied by φb will be φb*Mn=0.90*1355.70=1220 ft-kips.

How can the LL for the beam- part 4/4 of the Solved problem 9-9-6 be estimated?
The ultimate load for the simple beam Mult must be =< 1220 ft.kips. The span is equal to 45 FEET.
From the next slide, for the LRFD design, use 1.2D+1.6L=(0.15*1.2+1.60*LL)*(45^2)/8<=1220 ft-kips. Then (0.18+1.60*LL)*253.125<=1220.
The value of 1.60*LL=(1220/253.125)-0.18=4.639. The service Live Load LL=4.639/1.60=2.899 Kips/ft. Taken as LL=2.90 kip/ft, which matches the final solution by Prof. Salmon. Thanks a lot.

This is the next post, Moment Redistribution For Continuous Steel Beams. The post introduces the method for moment redistribution, setting the AISc code to reduce negative moments and adding 0.10 to the average value between supports and positive moments.
For useful external resources for steel beams. Chapter 8 – Bending Members