27- Part 3/4 of the Solved problem 9-9-6, how to find LL?

Last Updated on July 29, 2025 by Maged kamel

Part 3/4 for the Solved Problem 9-9-6, How To Find LL? 

The following slide image illustrates the content of post 27.

The limiting coefficient Lp/ry for the built-up section.

As we can see from the beginning of our solved problem that the bracing distance=15′, we want to get the Nominal moment due to lateral-torsional buckling and compare the value obtained against Mn due to Local buckling.

How to get the ry? it is obtained from the relation of r^2y= Iy/A, then we estimate Lp/ry, for the limiting ratio Lp/ry=300/ sqrt(Fy).
 In our part 3/4 for the solved problem where Fy=65 ksi, Lp/ry limiting ration =300/sqrt(65)=37.21, then the value is to be multiplied by ry.

After estimating its value, but we have a rather tough equation for Lr. First, we will estimate the ry value, for our built-up section where, r^2y=Iy/A, for Iy we estimate as we have a two flange and one web, then Iy can be estimated by the relation, Iy =parallel*perpendicular^3/12, for the flange=(5/8)*(16^3/12).

Multiply by 2 to include the lower flange as well. While for the web, Iy web=(26)*(5/16)^3/12. then Iy for the section=426.74 inch4.

Check lateral torsional buckling, the value of  Lp for the built-up section.

The total area At, we have estimated earlier as At/2=14.0625 inch2. Then At=2*14.0625= At=28.125 inch2.
ry =sqrt(Iy/At)=sqrt(426.74/28.125)=3.895 inch, that is the value of the ry. The radius of gyration for the Y-axis, then the plastic distance between bracings Lp limiting value=37.21*3.895=145.00 inch.

To convert to ft, divide /12=145/12=12.077 feet, Lp=12.10′. In our solved problem, part 3/4, the span is 45 feet, and the distance between bracings Lb=(L/3)=15″. L bracing=15′, the author aims to discuss the section in the second zone where Lb>Lp and <Lr.

Part 3/4 Of The Solved Problem 9-9-6, the equation of Lr.

Let us estimate each item Cw= Iy*h^2/4c^2, Iy= 426.74 inch4. The Mn value is between Mnp=1728 ft-kips and Mr=1114 ft-kips, but for Lr relation, Lr=1.95*rts* (E/0.70Fy)*sqrt(J*c/Sx*ho*sqrt(1+sqrt(1+6.76Fy*(0.7*Fy*Sx*h0/(E*j*c)^2.

Let us estimate each item Cw= Iy*h^2/4c^2, Iy=426.74 inch4, ok, to estimate then ho=(27.25-5/8)=26.625 inch.
ho^2=(26.625)^2 inch2., taken as ho^2, to review the relation, ho is the distance between the CG of flanges.
The overall distance=total height-(tf/2)),Cw=426.74*(26.625)^2/ 4* c^2, where c=1 for doubly symmetrical sections.cw=75628 inch6.For r^2ts =sqrt(Iy*Cw/ry).

The equation rts=(sqrt(Iy*Cw)/Sx), where Iy=426.74 inch4 and Cw warping coefficient Cw=75628, I have estimated all these values since we are not able to use the tables given by The AISC since our section is a built-up section.
r^2ts= sqrt(426.74*7.5628)/Sx, where Sx=294 inch3. then r^2ts=sqrt(426.74*7.5628)/294=19.32, then rts=4.395 inch

 We will estimate Lr=1.95*rts*(E/0.70*Fy), ts=4.395, E=29000ksi, Sx=294 inch3, Fy=65 ksi. This bracket value(1.95*4.395*29000/0.70*65)*sqrt(J*C)/sqrt(Sx*ho) where J is the torsional constant first, C=1, Sx =294, ho=26.625″. The big square root, but first I have estimated the value of (1.954.39529000/0.7065)1/sqrt(29426.625), was considered as 61.7395, then multiplied by sqrt(J1)sqrt(1+sqrt(1+6.67(0.765)(29426.625^2/(29000J*1).

I have computed most of the items for the square roots until we estimate the J value. The square roots are re-written as sqrt(1+sqrt(1+6.76*12.815/ j^2).

Review of J polar for non-round shapes.

From Prof. Beer’s handbook, chapter 3: If we have a fixed circular at one end, the longitudinal axis will be wrapped due to the torsion, and point A will move up to become point A’.

The torsion causes an angle φ, and the torsion=R*φ causes the rounded portion movement.
The shear strain γ is the change of the length /original length = radial angle = tan γ = γ=R*φ/L.
As we remember, the bending moment stress, f=M*Y/I, causes compression stress at the top and tension stress at the bottom. However, that equation is for the bending moment.

Now that we have a torsion, τ, the shear stress will be used instead of the bending stress f, τ max.

The torsion moment T will replace M, while R will replace y-max in the case of bending moment, since the section is round, where R is the radius. Instead of I, the polar moment of inertia  I-polar will be used.

I polar is considered as Jp, then the equation will be τmax=T*R/Jp, the strain gamma γ =R*φ/L, but as strain=τmax/G.
G instead of I to be used, but τmax=T*R/J. γmax= R*φ/L=T*R/Jp. Finally, we get the expression of φ=T*L/(Jp*G).

Review of J polar for the non-round shapes.

For the non-round shapes, there is torsional fixation on one side.
If we look at an element of length L, due to opposite Torsional moments, one at the fixed part, the other at the free end, both act against each other.

Check the τmax and φ in this case. Using the mathematical theory of elasticity for a straight bar with a uniform rectangular cross-section, then τmax =T/c1*ab^2, where a is the longer side length. At the same time, b is the shorter distance.

While φ=TL/Jpg will be replaced by TL/(c2ab^3)Jp as if Jp=c2ab^3, where c1 and c2 depend on a/b. The c1=c2=1/3(1-0.63b/a) for case of a/b>=5 only.

  J- value for the built-up section.

The built-up section, where the web height is > its width,  can be considered as a group of rectangles.
For c1 =c2 to be used at infinity, c1=c2=1/3 and  Jp =1/3*a*b^3. Now refer to the next slide, where J =1/3ab^3, then φ= TL/((1/3)ab^3G) for the case of a rectangle. J will be the sum of 1/3ab^3 for the I beam, where a is the longer side while b is the shorter side.

Now refer back to Lr for the value of J, which is the sum of(1/3)*a*b^3 or 1/3*sum of (longer side)*(shorter side)^3.
For the flange at top and bottom, a=16″, b=5/8″, for the web a=26″ and b=5/16″, J=1/3(16*5/8^3*2+ 26*(5/16)^3). J=2.868 inch3.

For the Lr=61.7392*sqrt(2.868)*sqrt(1+sqrt(1+123.25/(2.868)^2.

This is a detailed calculation to find the final value: Lr=104.575* (3.4897) divided by 12, Lr=30.41. Lb is>Lp and <Lr.

Estimation of the coefficient of bending, cb.

The next step is to estimate the Coefficient of bending, Cb, to continue calculating the Mn due to lateral-torsional buckling. I have started with parts AB and CD, which are similar. Estimate the moment values for four portions. Please refer to the first part of the next slide.

The following slide image shows the moment values for the 2nd and third quarter points.

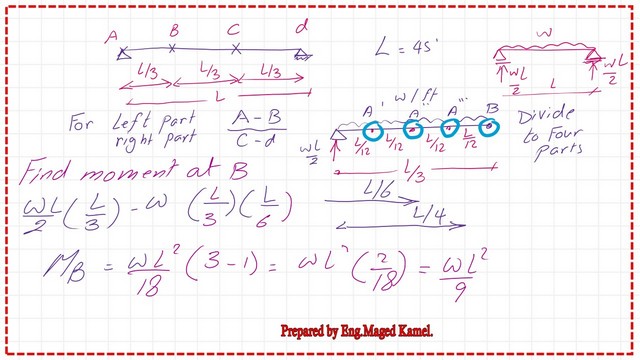
The following slide shows the detailed calculation for the Cb value for Part AB.

These are the CB values for These are the moment values for parts AB and CD.

The next post will include the required Final Live Load value.

This is the next post, Part 4/4, on Solved Problem 9-9-6. How To Find LL?
This is a very useful external resource for steel beams. Chapter 8 – Bending Members.