24b- Easy approach to solved problem-5-1-part 2-2.

Last Updated on May 11, 2025 by Maged kamel

Solved problem-5-1-part 2-2.

Solved problem-5-1-part 2-2. is the title of this post, in which we continue solving problem 5.1; we have obtained the required value of the moment of inertia, Ix, as equal to 440 inch4. We have selected W14x30 based on a Zx value of 44.40 inch3; we need to check its Ix value from Table 1-1. The Ix value equals 291.0 inch4, which is smaller than 440.0 inch4. So, we need to revise the selection.

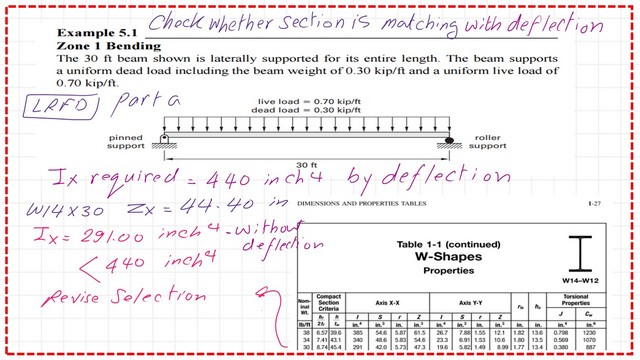
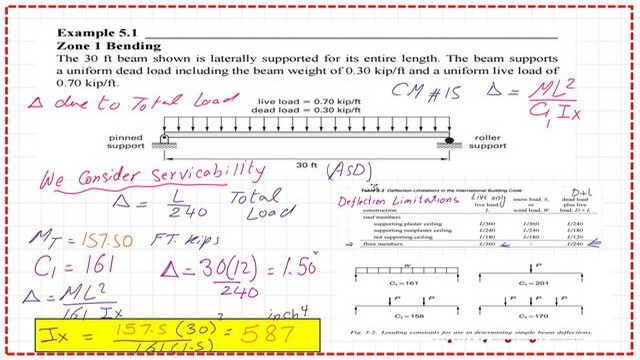
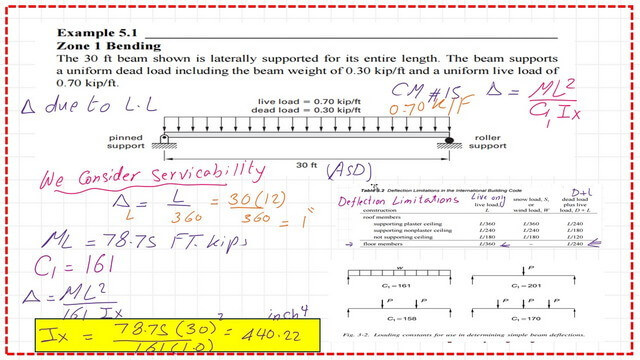
This is a reminder of the Moment of inertia of the required W section based on deflection due to live load.

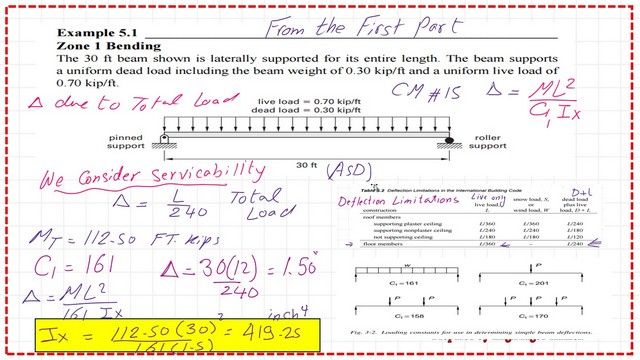
This is a reminder of the Moment of inertia of the required W section based on deflection due to total load.

Use Table 3-3 to select a new W section with Ix>or equal to 440 inch4.

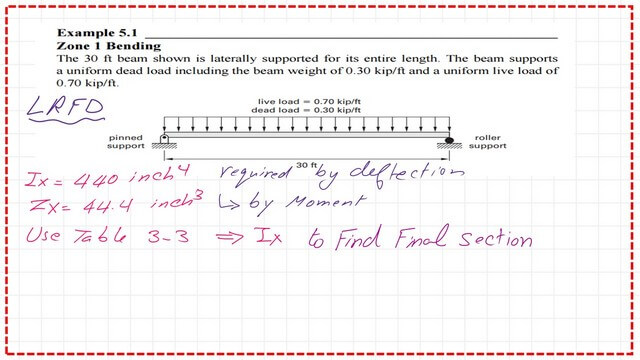
Use Table 3-3 for the solved problem-5-1-LRFD design.

Use Table 3-3 to select a new W and use the bold W18x35. This gives Ix equal to 510 inch4, greater than 440 inch4.

We have selected the first four W sections with Ix bigger than 440 inch4. We need to get the Zx values for these sections and select the final W section.

The next slide images show the data of the Four sections and why we have selected W18x35.

We will estimate the design strength for W18x35 based on the LRFD design. It will equal 249 ft. Kips, bigger than the Ultimate moment, equals 166.50 ft. Kips. The section W18x35 is adequate for design.

Find the required Zx based on the ASD design.

We need to estimate the required Zx for the beam based on the ASD design. The total moment equals 112.50 ft, kips, and the required Zx value equals 45.09 inch3

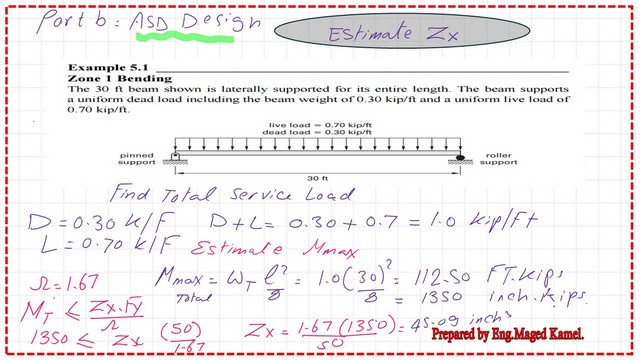
Due to the inertia requirement, the Ix value is 440 inches4, and the required Zx is 45.09 inches3; we need to use Table 3-3 based on Ix to find the proper section.

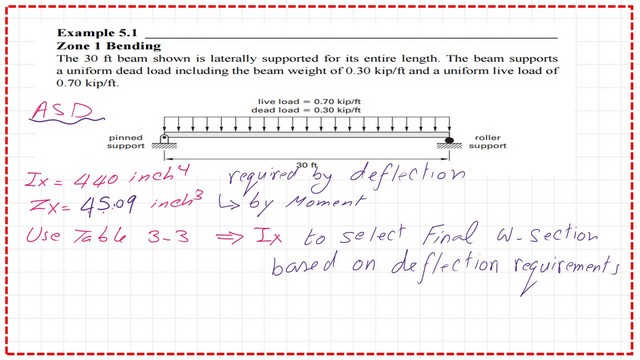
Use Table 3-3 for solved problem-5-1-ASD design.

Use Table 3-3 to select a new W and use the bold W18x35. This gives Ix equal to 510 inch4, greater than 440 inch4.

We have a total moment equal to 112.50 kips. Check the allowable strength for W18x35, which is equal to (1/omega)*Mt/Zx*Fy, equal to 166.0 Ft. kips. This value is bigger than the Total moment. The section is adequate for design based on the ASD design. Thanks a lot.

This a new quiz to check your understanding. There are Two questions.

As an external resource for the deflection Chapter 8 – Bending Members
For the next post, 25-part-1-4-solved-problem-9-9-6-1-4, how to find LL for a given slender section?