Last Updated on June 7, 2024 by Maged kamel

A Solved Problem 2-22 For Shear Stress Part-A.

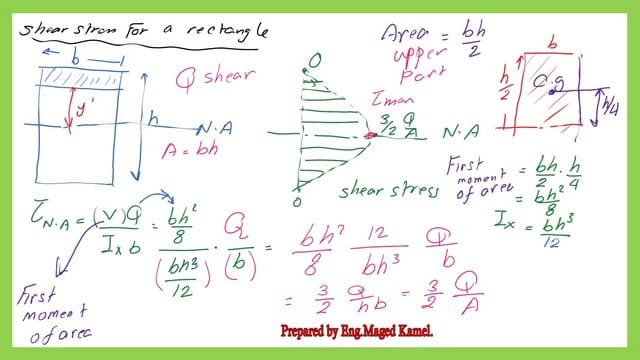
The next slide shows the shear stress distribution for a rectangular section. The shear stress formula can be written as τ max =V*Q/Ixx*b, where V is the statical first moment of area about the neutral axis for the portion enclosed by the upper edge and the neutral axis. Q is the acting force.

The Ix is the moment of inertia of the section at the x-axis, and b is the breadth of the section.

Derive the expression for the shear stress for a rectangular section.

We know the expression of the moment of inertia about the x-axis can be written as Ix= b*h^3/12, where b is the breadth of the rectangle while h is the height of the section.

The first moment of area is the area enclosed between the NA and the upper edge multiplied by the distance from Cg to the N.A. The enclosed area is (b*h/2), and the distance from CG is h/4.

The statical first moment of area =1*0.50(b*h)*(h/4)=b*h^2/8. The maximum shear stress at the neutral axis of the rectangular section can be estimated as the applied force multiplied by Q divided by the product of ix by b; the expression can be simplified to be τ _max =Q*(b*h^2/8)/(b*h^3/12)*b)=(3/2)*Q/A. A is the area of the rectangle.

How do you get the value for the first moment of area for the I section?

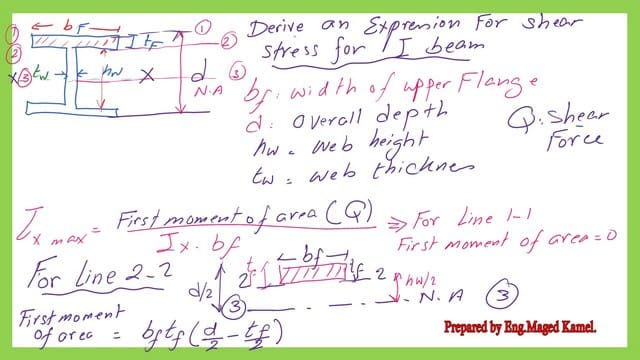
If we use the expression V as an indication for the first moment, the portion included between the upper edge and the neutral axis is the T-section or half of the complete I section.

We can find that the first moment of the area at the top of the flange=0; we can derive the expression for the first moment of the area at the bottom of the flange as shown in the next slide image.

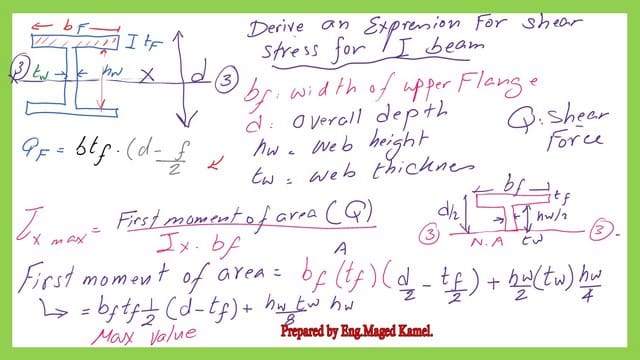
Then we have two items. The first moment of area for the I section consists of the first moment of area for Flange, and we will add the first moment of the web at the N. A.

The final first moment of area value equals the area of flange*y bar=bf*tf* (d/2-tf/2). The term d is the overall height of the I section, while the bf is the flange breadth.

The first moment of area for the V web at the N>A will be (1/2*hw*tw)*hw/4=1/8*(b*h2w). By adding these values together, we can get the final first moment of area for the I beam. Ix for the same section is shown on the next slide.

Two methods can be used to find the moment of inertia Ix for the I section. Please refer to the next slide image for more details.

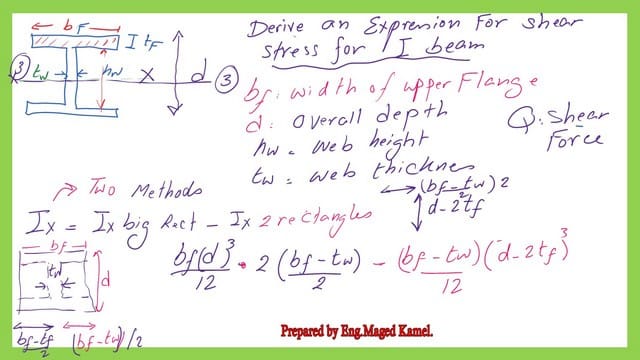
This is the second method for expressing the moment of inertia for Ix.

The next image will show the shear stress shape for an I section.

Part A of the solved problem 2.22 for shear stress.

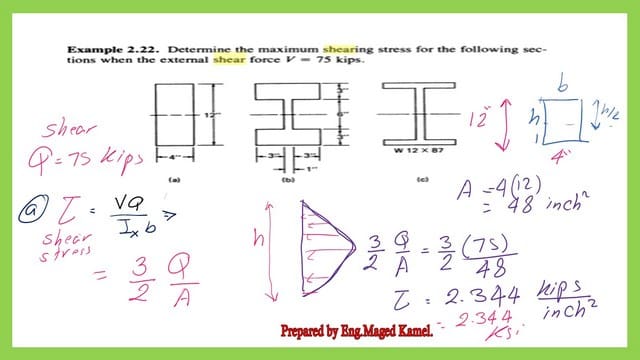
We have solved problem 2.22, for which the maximum shearing stress for the following sections when the external shear force V=75.0 kips must be estimated.

For part A, when the shear force is given as V=75 kips for a rectangular section, the expression for the maximum shear stress can be written as τ max =3/2*(Q/A).

Q is the applied force, and A is the area of the rectangle. The rectangle has a dimension of (4 inches) by (12 inches). The area will be equal to (4*12)=48 inch2.

We will substitute as τ _max =3/2*(75/(48)=2.344 ksi

In the next post, we will solve the same problem for the other shapes.

This is the next post, A-solved problem- 2-22 for shear stress.
For a good external site to estimate the shear stress for various sections.