Last Updated on September 29, 2024 by Maged kamel

Moment of inertia for an isosceles Triangle-Ix,Iy.

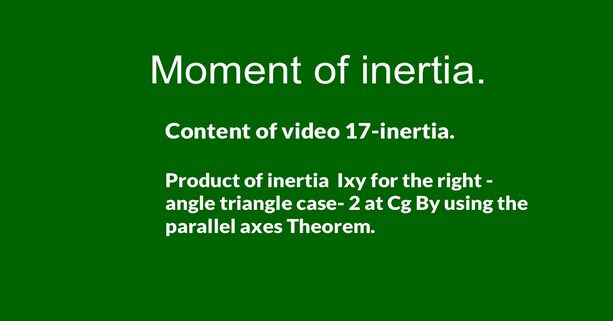
Moment of inertia for an isosceles triangle-Ix, Ixg.

1-The moment of inertia for an isosceles triangle Ix is obtained by considering the moment of inertia Ix for a Triangle, which we have obtained earlier an Ix= bh^3/12 and radius of gyration Kx^2 as Ix/area:b*h^3/12/(0.50*b*h)=h^2/6.

2-To get the moment of inertia at the Cg of the isosceles, which is termed Ix CG at the CG of the isosceles triangle we will deduct the product of area by the square of the distance from the Cg, which equals (A*y^2-bar) from the value of Ix, which equals bh^3/12, so we get the value of IxCg=b*h^3/36.

3-The square of the radius of Gyration, k^2cg can be estimated by dividing the moment of inertia about the Cg by the area, the value will be equal to Ixcg/A=h^2/18. For full data on the steps used, please refer to the next image.

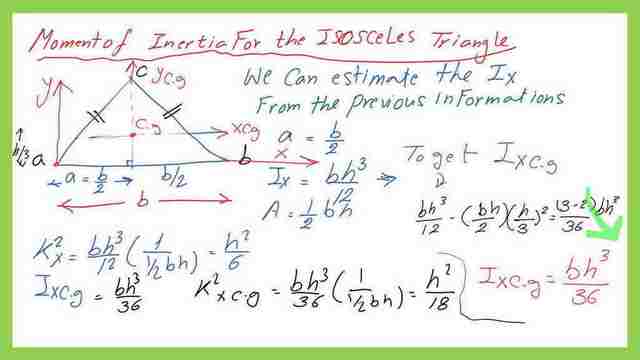
Moment of inertia for an Isosceles Triangle Iy & Iyg.

A- The moment of inertia for an isosceles Iy can be obtained after adjusting the terms of the Iy of the triangle, where the y-axis is an external axis passing by point a.

The moment of inertia Iy for the triangle that we have obtained earlier will substitute the value of (a )in the relation as 1/2*b, where b is the base length, after adjustment.

We will get the value for the moment of inertia Iy for an isosceles triangle as Iy=(7/48)*h*b^3, where b is the base while h is the height of the isosceles triangle.

B- for the radius of gyration Ky for a triangle expressed as k^2y=Iy/A=(7/48)*h*b^3/(0.50*b*h)= (7/24)b^2.

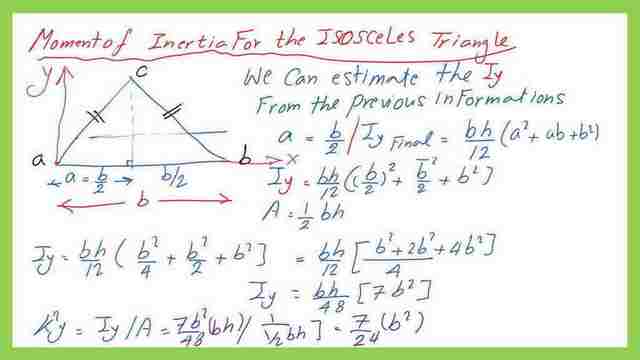
C-The moment of inertia Iy at the Cg of the isosceles triangle can be obtained by subtracting (A*x-bar ^2) from the estimated value of the moment of inertia Iy for a triangle as Iy=(7/48)*h*b^3, The distance from the Cg to the vertical y-axis which is x bar value =b/2.

Iy Cg=(h*b^3)/48, which expresses the moment of inertia about the Cg.
D- for the radius of gyration Ky for an isosceles triangle as k^2y at the Cg =Iy cg/A=(1/48)*h*b^3/(0.50*b*h)= (13/24)b^2.

For more information, please refer to the next slide image.

This is the PDF file used in the illustration of this post.

For an external resource, the definition of the moment of inertia with solved problems is 2nd moment of inertia.

The next post is Product of inertia Ixy for an isosceles triangle.