Last Updated on February 2, 2023 by Maged kamel

Area and Cg for a circle.

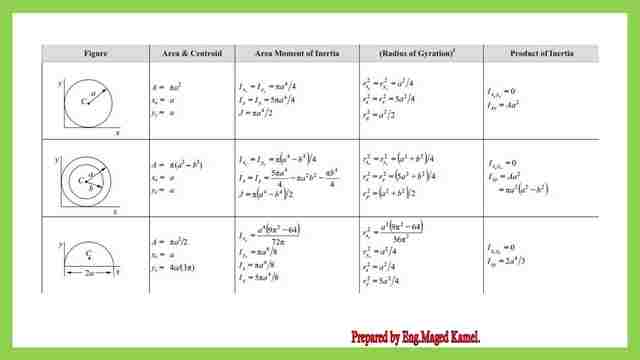
Reference handbook 10.00 value for the area and Cg for a circle.

There is a list of the common round shapes area and CG value. Our first case is the case of a circle.

The external axes X&Y are tangents to the circle which has a radius value of R.

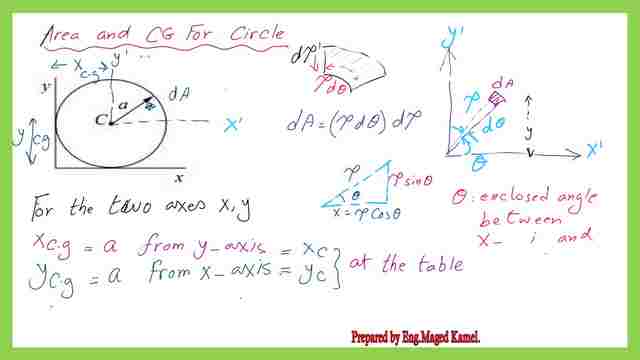
Area and Cg for a circle- select an area dA.

We have a circle with radius a, for which it is required to get the area and CG. We can find that there are two axes X’ and Y’ dividing the circle into four similar parts. Due to that symmetry, we expect that the Cg or the center of gravity will be at the point of intersections of these two axes.

We have two axes X&Y are tangents to the circle and apart from the X’s axis by a value of a, which is the circle radius. We will select a small area dA that has a radius of ρ from the intersection of the two axes X’ and Y’.

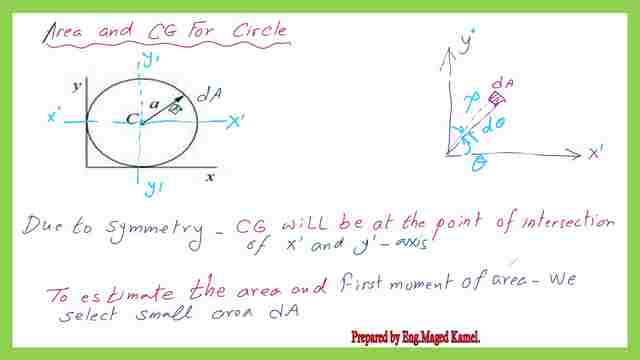
The small area dA has an angle from the X’ axis that is equal to θ and is enclosed by an angle dθ and a width equal to dρ. The area of the strip dA=( ρ*dρ* dθ).

Area and Cg for a circle- first moment of area about the x’-axis.

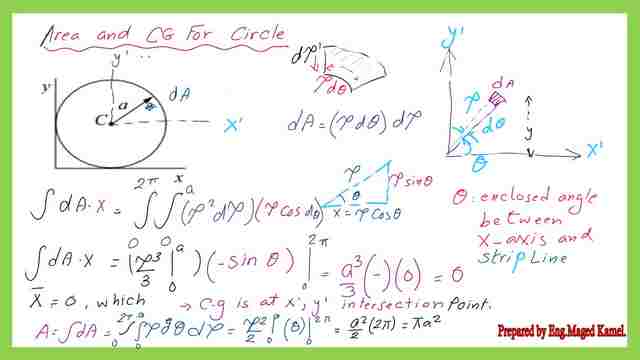
The first moment of area for the small area dA about the X’-axis is the product of that area by the vertical distance to the X’- axis. The vertical distance is y which is equal to ρ*sin θ. The moment dMx=dA*(y)=(ρ*dρ*d θ)*(ρ*sin θ). it will be simplified to (ρ^2*dρ*sin θ*dθ).

The expression of the first moment of area about X’ axis is shown in the next slide image.

For the first moment of area for the whole circle, we will use double integration since we have to integrate for ρ from ρ=0 to ρ=a. the second integration os for the angle dθ from θ equal to zero to dθ equal to 2*π or 360 degrees. dA*y=∬(ρ^2*dρ*sin θ*dθ).

This is the value of the first integration will be ∫ρ^2*dρ from zero to a=1/3*ρ^3 after substitution will lead to 1/3*(a^3-0)=a^3/3. While for the second integration ∫(sin θ*dθ)=-cos(θ), after substituting from zero to 2*π.
The value will be (- (cos(2*π)- cos(0))=zero.

But the circle area can be found from the integration of dA= ∬(ρ*dρ*dθ) from ρ=0 to ρ=a and for dθ from θ equals zero to dθ=2*π, the final expression for the area is dA=ρ^2*0.5*(θ), substitute to get A=0.50*(a^2-0)*(2*π-0)=π*a^2.

Divide A*y/A to get Y bar will lead to ybar=0/π*a^2=0. This includes that the Cg lies on the X’ axis.

The details of the calculations for the first moment of area for the circle about the X’ axis and the value of the area are shown in the next slide image.

Area and Cg for a circle- first moment of area about the Y’-axis.

The first moment of area dA about the y’-axis is dMy=dA*(x)=(ρ*dρ*d θ)*(ρ*cos θ). it will be simplified to (ρ^2*dρ*cos θ*dθ).

For the first moment of area for the whole circle, we will use double integration since we have to integrate for ρ from ρ=0 to ρ=a. The second integration os for the angle dθ from θ equal to zero to dθ equal to 2*π or 360 degrees. dA*y=∬(ρ^2*dρ*cos θ*dθ).

∫ρ^2*dρ from zero to a=1/3*ρ^3 after substitution will lead to 1/3*(a^3-0)=a^3/3, while the ∫(cos θ*dθ)=-cos(θ), after substitute from zero to 2*π. The value will be (+ (sin(2*π)-sin(0))=zero.

We have estimated the area of the circle as equal to π*a^2. x bar is equal to A*X/A= zero/π*a^2 =0. This includes that the Cg lies on the Y’ axis.

The details of the calculations for the first moment of area for the circle about the Y’ axis and the value of the area are shown in the next slide image.

The Cg distances are x bar=a and y bar=a and are shown in the next slide image.

We have completed the subject of the area and Cg for a circle.

The next post will be on how to estimate the area and Cg for a circular shaft.

This is a link to a very useful site: Engineering statics open and interactive.