Last Updated on July 1, 2025 by Maged kamel
Round numbers to Decimal points, Significant Digits.
What is the place value for a number?
Place value, in mathematics, describes the value of every digit in a number depending on its position. These positions start from the unit’s place (one place). The order of the place value of digits in a number from right to left is expressed as ones/units, tens, hundreds, thousands, and thousands.

How do we round numbers?
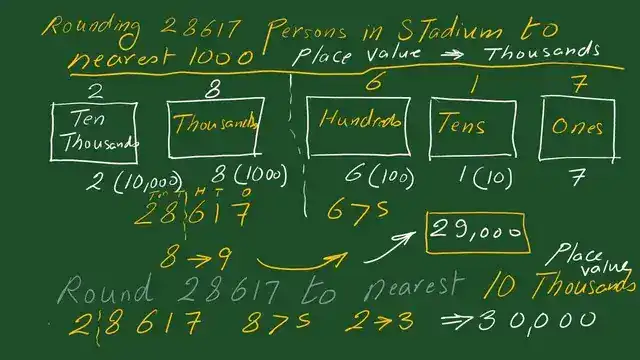
This is the fourth lecture about sets. This is the revision for rounding numbers. It is a part of discrete math. To round the number of 28617 persons in a stadium, it is required to round to the nearest 1000. The place value is 8, and the number to the right is 6, which is bigger than 5, so 28617 will be rounded to 29000 to the nearest thousand.
To round the number of 28617 persons in a stadium to the nearest ten thousand, the place value will be 2. The correct number is 8, which is bigger than 5. Thus, 28617 will be rounded to 30000 in ten thousand.
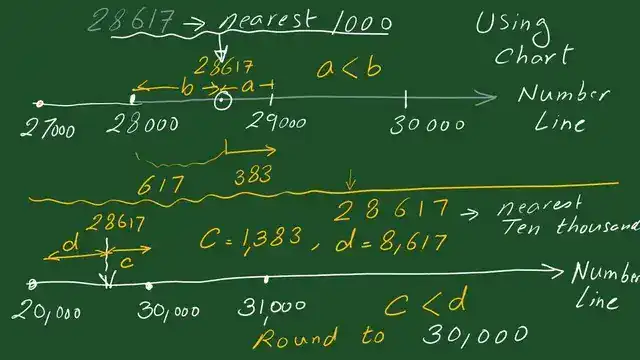
We can use the number line to verify our solution; please refer to the next slide Image..
Rounding numbers to decimal points.
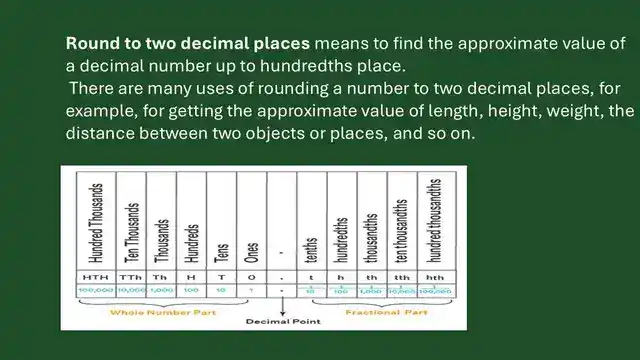
Let’s examine a new subject: rounding decimals. What is the decimal point? This point differentiates between the whole number on the left and the decimals on the right.
The number can be approximated to a decimal point. The number at the left of a decimal point is a whole number. The first number to the left of the decimal point is ones, followed by tens and thousands. The number at the right of a decimal point is a length, followed by one thousandth, followed by ten thousand, etc. Please refer to the next slide image.
To round 7.8764 to one DP, the place value is on the Number 8. At the right of Number 8, there is number 6, which is bigger than 5, and the rounded number is 7.9 to the nearest one Dp.

The following slide image shows a chart for rounding to the required decimal points and the corresponding values.
Solved examples for rounding numbers to decimal points.
Another example is Round 5.574, which is the nearest two decimal points. Consider that the number 4 is less than 5, so the found rounded value is 5.57.
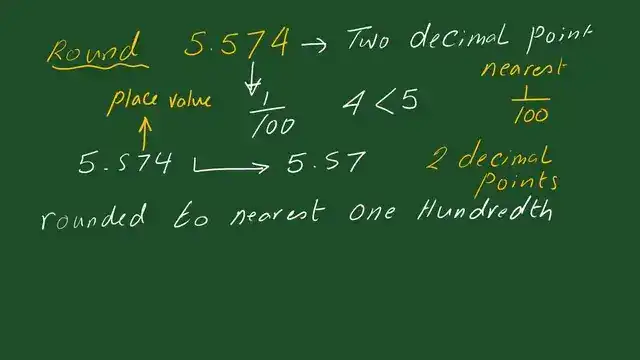
Another example: it is required to round 3.146. It is required to be rounded to hundreds; it will be rounded to 3.14 since it is required to have two decimal places. Why? because 1 is 1/10, while 4 is 4/100
1 is < 5, then 4 will not be changed.
The same 3.1416 is to be rounded to thousands or (3)(d.ps), then 6 is >5, then 1 will be upgraded to 2, and the final value is 3.142.

Rounding numbers to significant figures.
The next item is Significant figures, which are helpful in experiments for people who need accurate measurements. That is why they are referred to as significant figures. This can count from left to right. Four rules cover rounding to the nearest significant figure. Please refer to the next slide image.
For example, 1.239. It is required to round to three significant digits. To count the three digits, start from the left and consider 1 as the first significant figure, based on rule number 1.
Look at the right side of the third number and check that if the number is < 5, then the number will be unchanged. In our case, the 1.239 will be rounded to 1.24 since 9 is<5, which is three Significant digits.

The number 1.239 will be rounded to one significant figure, consider the first significant figure is 1 and the right number is 2, the final rounded to one SF is 1.
Round 134.9 to one scientific digit. The place value is 1, and the right number is three less 5, then the final rounded number is 100 to one SF value.

Solved examples for Rounding significant figures.
For example, a given number is 0.0043, which must be rounded to one significant. To solve, we will forget the leading zeros based on rule 3, and start with the first figure, which is 4; check whether 5; if less than 5, then keep 4 without an upgrade, and the final round is 0.004. to round 0.0165 to 2Sf the round is 0.017.

To round 1.0055 to a different SF, we start by rounding to 1 SF. The round will be 1, and the place value is 1. To round the same number to 2SF, the round is 1.0 since zero is less than 5. Again, round 1.0055 to 3SF, the round is 1.01. Please refer to the next slide image for another example.

Rounding Numbers to the nearest whole number.
As we agreed before, round to the nearest whole number. The decimal point separates the whole number on the left from the fractions or decimals on the right.
To round to the nearest whole number, check the number to the right of the decimal point. If <5, then disregard; if >5, then upgrade. For our case, the six will be upgraded to 7. The nearest whole number will be 107. No fractions or decimals are to be included.
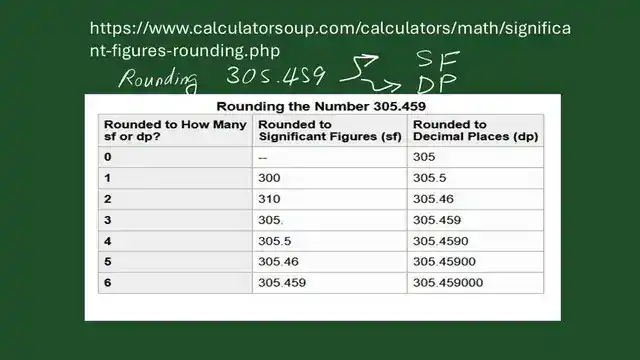
There is a good SF calculator, and I use the table to show the difference between rounding 305.459 and different Df and Sf.
Another example is round 239.456 to the nearest hundred; this is a mixed number. For the nearest 100, the answer is to neglect the fraction.
For the Nearest hundredth, make a line between 2 and 3, check that 3 is<5, 2 will be left, and place 0 and 0 to the right; the round will be 200. There will be no fractions. The idea is to consider the whole number at the left of the decimal. Another example is Round 78.546 to two decimal points; the result is shown in the slide image.
For an external link, math is fun for more details about the subject.
The following post is no-5, Solved quizzes.
You can use a calculator from Calculator Soup to find the corresponding rounding for any number you wish.