Last Updated on June 2, 2025 by Maged kamel
Solved Problem 13-28 For Compression Members-FE Exam.
This is a summary of the content of the post-8 introduction to solved problem 13-28, how to use Table 4-1 from NCEES Reference Handbook 10.4?

Detailed illustration for the Solved problem13-28 from Prof. Iqbal’s handbook.
We are going to have to look for a solved problem from Prof. Iqbal’s book, and the main title is How to use table AISC table 4-1 from the table; we can estimate the Φ*Pn available strength.
A solved problem is from M. Iqbal. A W12 x50 is used as a column, as shown, Fy=50 ksi, and the support is fixed at the bottom.
With a guided roller at the top, it is required to estimate the available strength in axial compression in kips; select the correct answer from the following options: 1) 90 kips, 2) 270 kips, 3) 360 kips, and 4) 660 kips.

For the solution, the roller allows movement in a delta, but the slope is zero. And provide a moment. As if fixed from the top, it contains both movement and Fixation For both directions, x and y. We will choose type C. The Euler buckling value of Pcr=pi^2 EI/ (k*L)^2 is shown in the next slide image.
Table C-A-7-1 gives six cases of support conditions and the corresponding and recommended effective value K values.
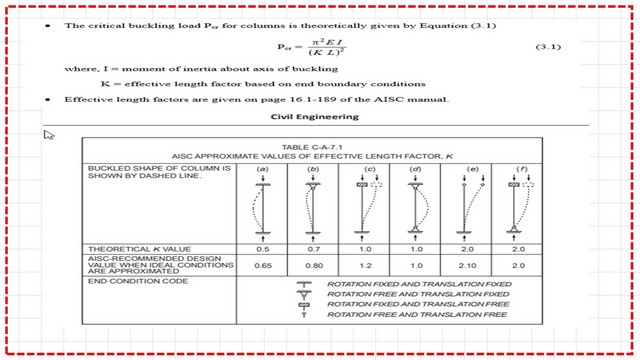
What is the selected K value?
The K value of Type C is the same =1.2 as recommended from Table C-A-7-1 for both X and y directions.
For solved problem 13-28. the value of Lex equals Ley and is 18 feet. The next step is to use Table 1-1 for the data of the W12x50.

How to use Table 1-1-FE-Handbook 10.4?
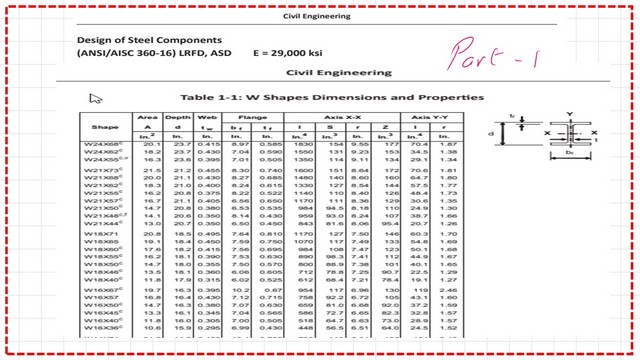
This is a table quoted from the FE Reference handbook in which there is a list of W sections, for which the area, depth, flange width & thickness, and inertias about axis x and axis y are given; for E value of 29000 ksi and yield stress of 50 ksi. The next slide image shows the first part of Table 1-1.
The required W12x50 is not a slender column since no C letter is included in W12x50.
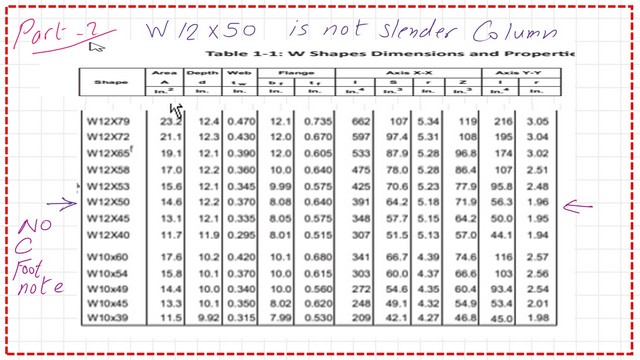
What is the data for W 12x 50 from Table 1-1? The area equals 14.60 inches 2; the rx is 5.18 inches, and the ry= 1.96 inches.

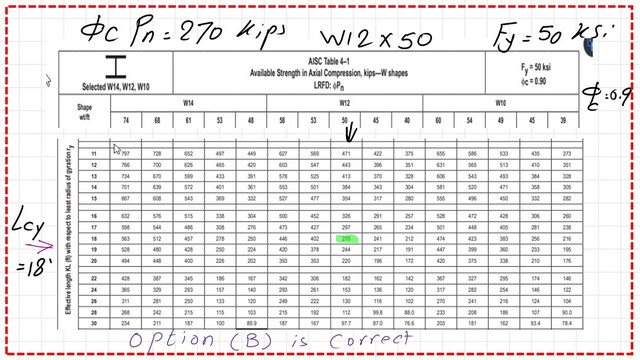
What is the requirement of Table 4-1?
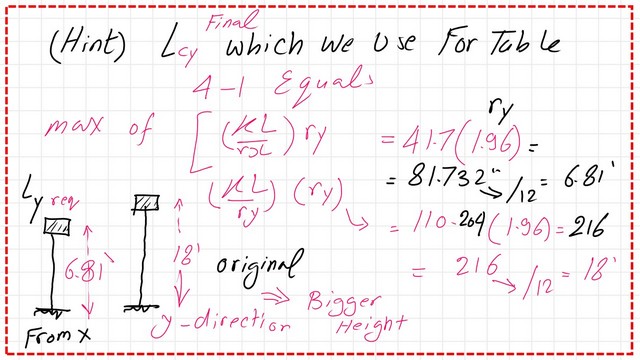
Table 4-1 requires that the tabulated effective length concerning ry is the maximum value of ly required from the X, or the ly modified which is Lex*(ry/rx), direction, and the ly value from the y-direction, the lc y required equals 6.81 feet; please refer to the following slide image.
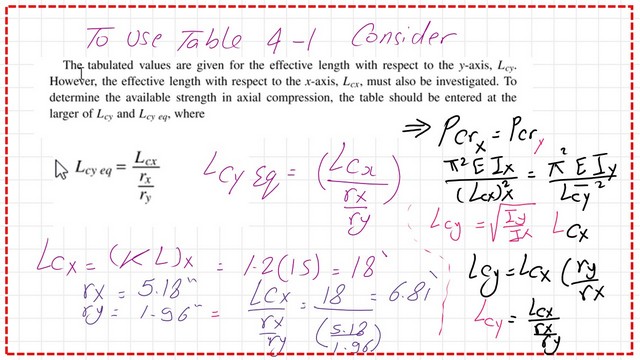
The ly value equals 18 feet; the selected effective length is the maximum value of 8.61, and 18 feet, it will be 18 feet; we will proceed to Table 4-1 to get the available strength or the LRfd value.

What is the available strength from Table 4-1?
We use Ly equals 18 feet, we move horizontally, and we drop a line from the top W12x50 section; the intersection will give the available strength, which is equal to 270 kips; the solution matches with option B).
Solved problem 13-28-Use Provision E-1 from AISC-360-16.
We could get the same result by using The E-1 provision of AISC-360-16, checking whether the given column is short or long, and then choosing the appropriate Fcr equation to use.
This curve we have already discussed, at the value for KL/r = 4.71* sqrt of (E/Fy), is the parameter that distinguishes between short columns and long columns.
If the value of Le/r= KL/r > 4.71* sqrt of (E/Fy), then the column is considered as a Long column, and hence the Euler Elastic stress. The division of pi^2 EI/ (kl)^2 over the area, then the stress will be (pi^2 E/ (le/r)^2).
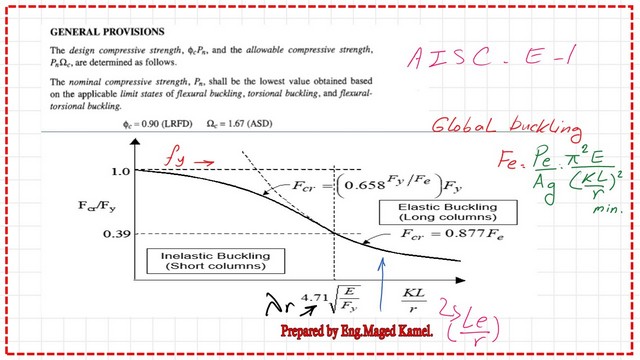
The limiting value for Fy=50 ksi, E=29000 ksi is 113.43.
We will estimate the lengths of column lcx, Lcy, the value for KL/r = 4.71* sqrt of (E/Fy), which is the parameter that distinguishes between short columns and long columns.
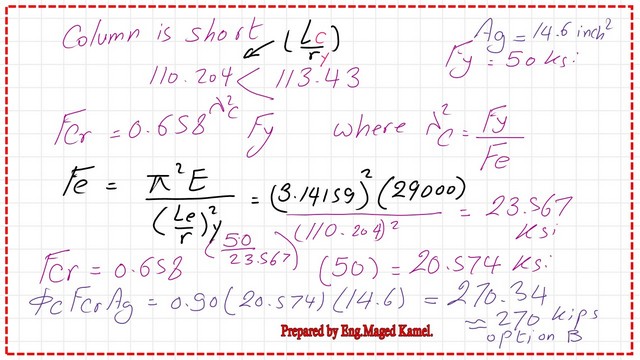
If the value of KL/r > 4.71* sqrt of (E/Fy), then the column is considered as a Long column, and hence the Euler Elastic stress. The division of pi^2 EI/ (kl)^2 over the area, then the stress will be (pi^2 E/ (kl/r)^2). The value of Lcx=41.70; the value of the lcy equals 110.240. Select the larger value, which is 110.204.

In the next slide, there is a hint: what is Lcye from the column in the x direction as compared to the Lcy in the Y direction?
We will apply the Fcr equation for the short column; the critical stress equals 0.658^lambda2 *Fy, where lambda ^2=Fy/Fe. The Euler stress equals 23.567 ksi, the fcr=20.574 ksi, multiplied by phi by the area to get the LRFD value of the available strength, which equals 270.34, very close to option B.
Please refer to the following slide image for more details.
This is the next post, Two Solved Problems for Column Analysis.
For a valuable external resource, Concentrically Loaded Compression Members.
