35-Solved problem 8-22-for Plastic nominal load( 1/2).

Last Updated on June 7, 2024 by Maged kamel

Solved problem 8-22-Plastic nominal load (1/2).

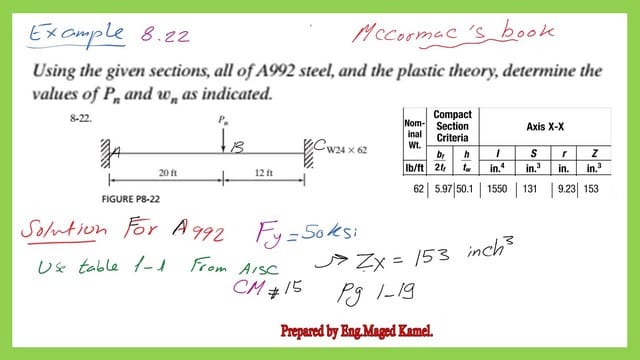
In the first solved problem 8-22, we use the given section of ASTM A992 and plastic theory. Determine the value of the P-nominal and W-nominal as indicated. We have several examples for M nominal, including concentrated loads, P loads, and others, containing uniformly distributed loads, W nominal to be estimated. This example is from Prof. McCormick’s chapter 8. We have Fy=50 ksi, and the Zx value is 153 inch3 from CM#15, pages 1-19.

How many plastic hinges are needed for collapse?

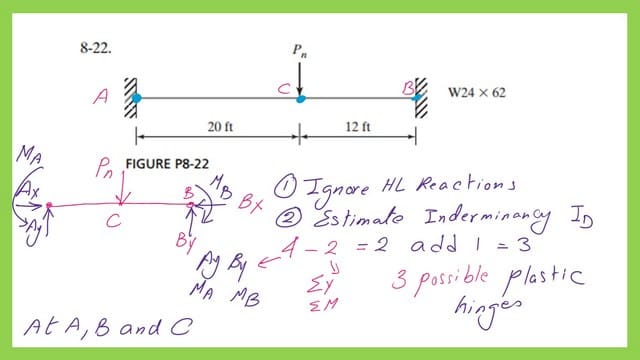
In the next slide image, we will examine the number of inderminancyId and add one to find the number of possible plastic hinges after we ignore the horizontal reactions. As we can see, there are three possible plastic hinges, two at A and B and one at point C.

Solve for the value of Nominal load by the lower bound theory.

We will estimate the reactions at A and b and find the maximum value of the moment at point C, which will equal 60*P/8. for three plastic hinges created, we have 2Mp value equals to =60P/8, which in terms equal to 2*Zx*Fy. We have Zx from the first slide equals 153 inch3 and Fy=50 ksi.

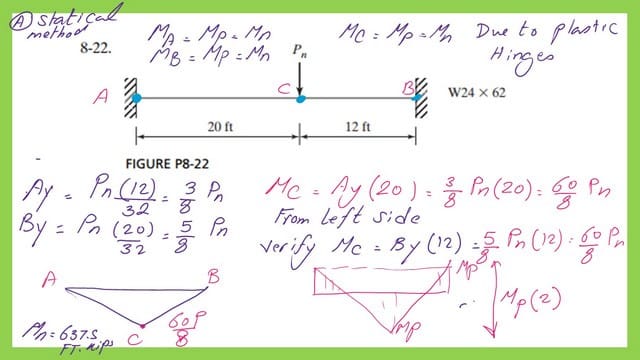
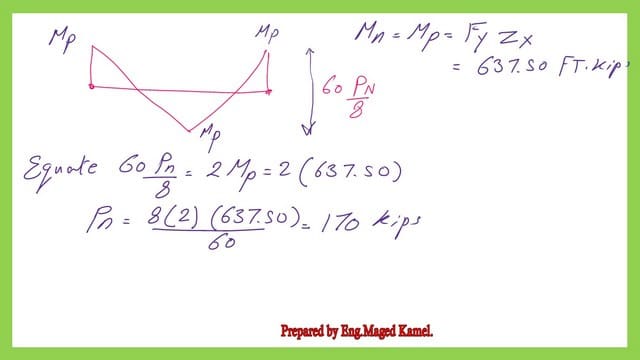
Evaluate the nominal Moment value, which is equal to Fy*Zx=50*153=7650 inch. Kips. Divide by 12, and we will have Mp=637.50 Feet. Kips. We can find that 60*Pn/8=2*637.50. The final value of Pn=170.0 kips.

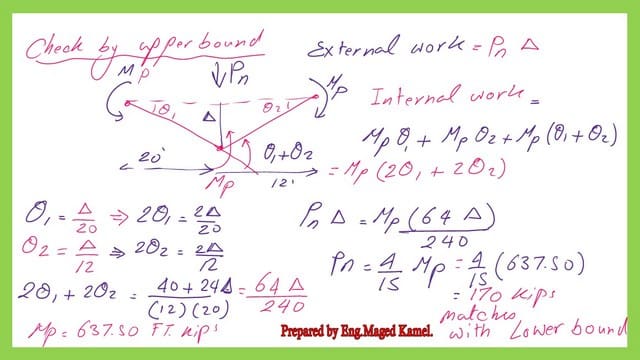
Find the value of Pn by the upper bound theorem.

In the next slide, this is the Upper bound, with the mechanism’s assumed hinge location under the load. There is a slope of θ value at the left and θ1 at the right support. Equate the external work to the internal work. The external work equals Pn*delta, while the internal work equals Mp*Δ.The value of θ1=Δ/20, θ2=Δ/12.
We have Mp, which is a positive plastic moment with an angle=θ+θ1, and two plastic moments at the two supports, at support A, for which the angle is θ, while at support B, the angle is θ1.

The internal Work =Mp*θ1+Mp*θ2+Mp*(θ1+θ2). For 2*(θ1+θ2)=2*(Δ/20)+(Δ/12)=64*Δ/240. the Mp value is 637.50 Ft. we find that Pn=170.0 Kips.

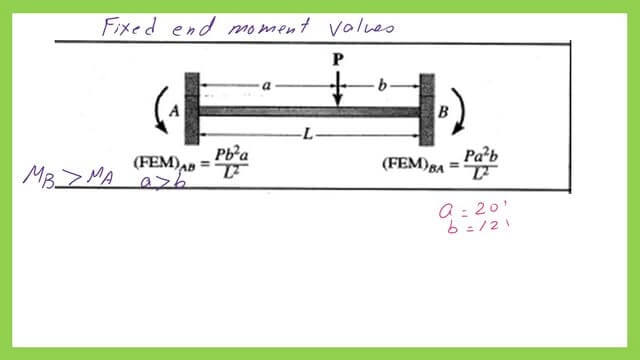
Fixed end moments values.

In the next slide image, we can find the values of the fixed end moments at both ends A and B; in our case, a=20 Feet while b=12Ft.

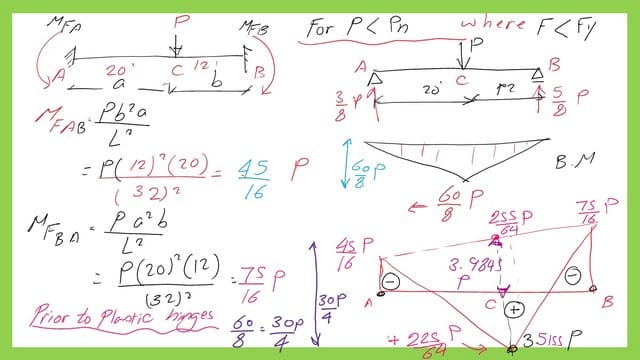
The fixed-end beam with maximum moment values is important. These points will have plastic hinges at the nominal load, so we will consider the fixed-end beam in the case of a working load and these places.

Consider the case of a fixed-end beam with a working concentrated load P at a distance equal to 20 feet from the left support A.

The fixed Moment at A equals P*12^2*20/32^2=45*P/16. The fixed Moment at B equals P*20^2*12/32^2=75*P/16. The positive moment value at point C equals to 60*P/8. Finally, we have the sketch for the values of moments at A, b, and C. The largest value of the moment is at Point B, followed by the moment at c, and the least at Point A. The first plastic hinge will occur at point B, the second plastic hinge at point C, and the third at point A.

The final positive value will be equal to 3.51155P. Please refer to the next slide image for more details.

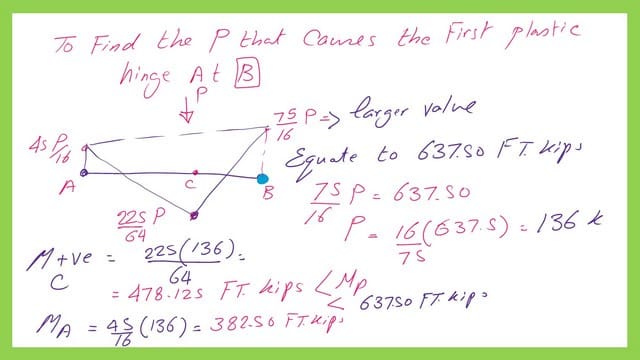
What is the load that creates the first plastic hinge?

We have found that Pn=170 kips creates three hinges, but a lesser load creates the first and second hinges. We will return to the working load condition. Equate the end moment at b to mp, which is 637.50 Ft.kips, and we can find that Pn=136.0 kips.

We substitute by Pn=136.0 kips to get MA and Mc values.

The fixed moment at A will be equal to 382.50 ft. kips. The positive moment will be equal to is 45/16 *P =382.50 Ft.kips; this moment is less than the Mp value, so there is no second plastic hinge yet at Pn=136.0 kips. hinge

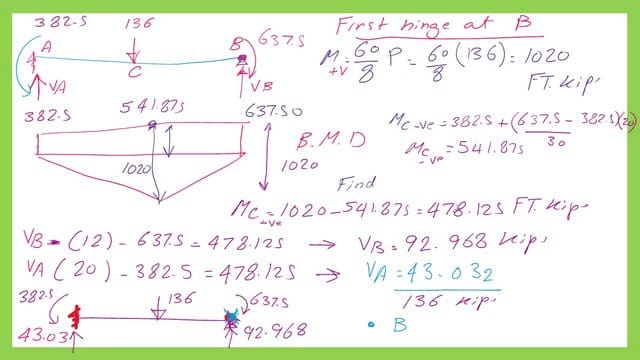
Shear and moment values for P=136 Kips.

Due to a load of P=136 Kips, there will be two end moments, one at the left support with 382.50 Ft. kips and a right moment of the value of 637.50 Ft. kips. The positive moment will be equal to 478.1255 Ft. kips. We can find the shear value VA=43.032 kips and Vb=92.968 Kips.

What is the load that creates the second plastic hinge?

The second hinge will be at point c. When the moment at c equals 637.50 ft. Kips, we will increase the load by more than 136 kips and estimate the corresponding positive moment. At P=160 kips, the fixed moment at A is equal to 450 ft. Kips, the moment at b is unchanged, and the positive moment at C will be equal to 632.81 ft. Kips; which is less than 637.50 Ft.kips.

There is a little remaining moment for the second plastic hinge to be formed. The next slide image shows the shear values at A and B.

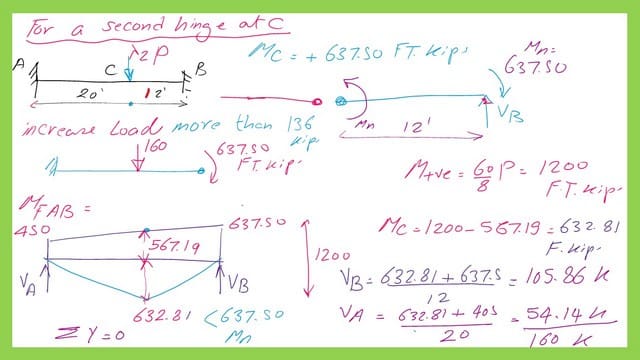
If we wish to have a Plastic moment at C, we take a moment at C and find the value of Vb, which equals 2Mp/12 or 637.50*2/12=106.25 Kips. The shear value is bigger than Vb for P=160.0 kips.

The Load is bigger than 160.0 kips; by trials, we will find that P=160.726 kips. Estimate the M positive value, which equals 60*P/8=60*160.726/8=1205.445 ft. kips. Find the fixed moment at A, equal to 452.04 Ft.kips. The corresponding reaction value at Ah is equal to 54.477 kips. The summation of vertical forces equals zero.

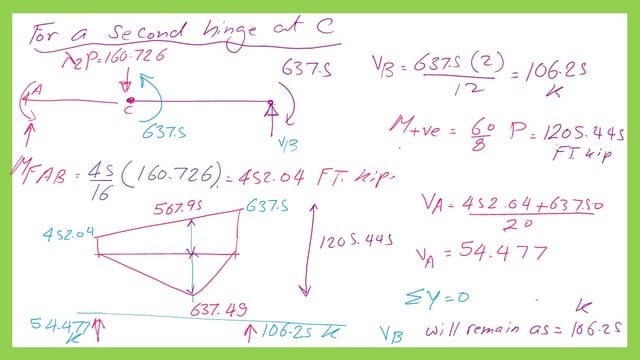
Before the third plastic hinge formation.

Let us try P=165 kips; before the third hinge formation, we will estimate the Moment at A. it is important to note that the reaction at B is kept unchanged and equal to 106.25 kips; if we increase this will create a bigger moment at c more than Mp which is not correct.

We estimate the shear VA, will be equal to 58.75 kips. Take a moment at c and find the value of MA; it will equal 537.50ft. kips, which is less than Mp of 637.50 ft. kips.

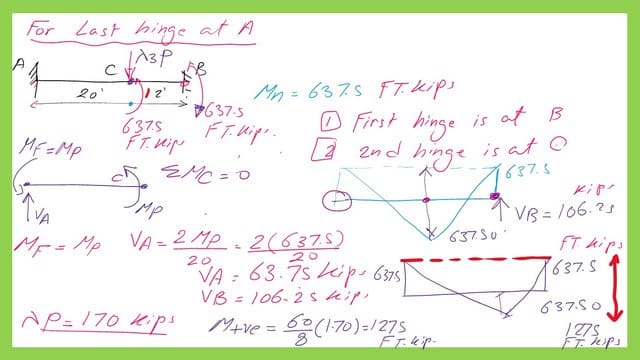
The Third plastic hinge nominal load

Consider moment at c equals Mp and estimate the shear value at A; consider MA equals Mp, Ay=2*Mp/20=63.75 kips. The final load Pn=VA+Vb=63.75+106.25=170.0 kips, which we estimated earlier by both the lower and upper bounds theorem.

Thanks a lot. I hope to see you in the next post, in which we will verify our solution using MASTAN-2. This is a link to part -2 post 35A.

Have more information about the structural analysis –III.
for another solved problems, Solved problems 8-32 for the plastic Nominal Uniform load.