Last Updated on July 11, 2025 by Maged kamel
Collapse load for an indeterminate beam.
What is the difference between the equilibrium or static method and the kinematics or mechanism method? From Prof. Capprani’s structural analysis III.
The Collapse load for an indeterminate beam by the static method.
We want to estimate the collapse load for an indeterminate beam. which has fixed support at point A and roller support at point B, which is statically indeterminate of degree-1. There is a P load at point C at a distance of L/2 from the left support.
It is required to estimate the collapse load P collapse and plastic moment Mp and find out their relation, by using the static method.
For the static method, the value of M -ve at the left support should be known, the bending moment is to be drawn, and the sagging moment of PL/4 to be subtracted from the moment value as will be shown to get M+ve. value.
It is required to estimate the collapse load P collapse and plastic moment Mp and find out their relation, by using the static method.
For the static method, the value of M -ve at the left support should be known, the bending moment is to be drawn, and the sagging moment of PL/4 to be subtracted from the moment value as will be shown to get the positive M+ve. value.
The Fixed-beam at A and with roller support at b is considered as a combination of two beams or Superposition of two cases, the first case where a simple beam acted upon by load P and the second case, due to fixation at A, Moment M will act at the left support A.
Then, we add these two cases to get the final moment diagram. At support A, we have Ma=(3/16)*P*L.
and zero value at B. and for the load case, we have moment value =P*L/4, due to the Reaction at A=P/2, causing moment=(P/2*L/4)=P*L/4. The final moment at c=P*L/4-(0.50)*(3/16)*P*L=(5/32)*P*L.
The proposed positions of plastic hinges.
The load P is applied gradually until the value of the P collapse is reached, that P collapse creates M-plastic at the fixed end.
Let us check the mechanism of collapse. We have A as fixed support with 3 reactions, and there is roller support with one reaction only, adding together we will have (3+1)=4.
We have one degree of indeterminacy. Add 1 to this one-degree indeterminacy. Then the number of hinges required to create collapse to that system is =2.
The proposed location for the first hinge is at the location of the load that creates a maximum moment. The first hinge will be placed at point C, and the second hinge will be located at the fixed support, resulting in two hinges.
The direction of Mp is at the fixed-end is anti-clockwise, while the second Mp is at the middle of the span, at point C. Here is the sketch of the two moments.
The next sketch is created by placing the two diagrams together. On joint A we have Mp value and Mp= zero at the other joint, and for the +ve moment, we have 0, at point A, PL/4 at point C, and zero at point b.
Check the moment at C, (1/2Mp)+Mp positive at b, we add (1/2Mp)+Mp=(P*L/4). While applying P, Mp -ve at the support A is created first, then further increase of load, then M positive reaches Mp at C, the total positive moment =P collapse*L/4.
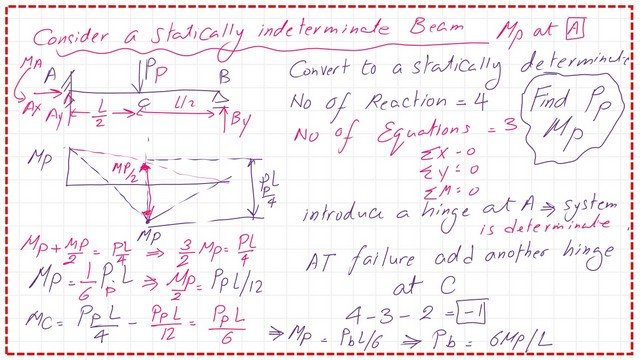
Then (0.50Mp+Mp)+ Pp*L/4, 2 will go with 2, Mp=(1/6)*Pp*L. The Plastic Load or the collapse load Pp=6*Mp/L.
The Collapse load for an indeterminate beam by the kinematic method.
To create a collapse, two hinges will be placed; the first will be at point A, and the second will be at point B. Due to the acting P collapse load, deflection Δ will occur, and failure will happen.
The mechanism of failure is due to the two hinges, at the collapse, there will be angle θ, at the collapse, there will be angle θ at both points A, B. θ= tan(θ), since the angle is small. Accordingly, θ= tan θ=(Δ)/(L/2), there is symmetry at point C.
The external work PpΔ= internal work=Mpθ+Mp(2θ). PpΔ=3Mpθ.While θ=2Δ/L, Pp==6*Mp/l, which is the same result obtained from the static method.
To determine the reactions due to the acting load P. Two reactions of P/2 at the two supports will exist, but due to Mp, another reaction will be created, an upward reaction at A, Ra=M*P/L to resist the end moment at A.
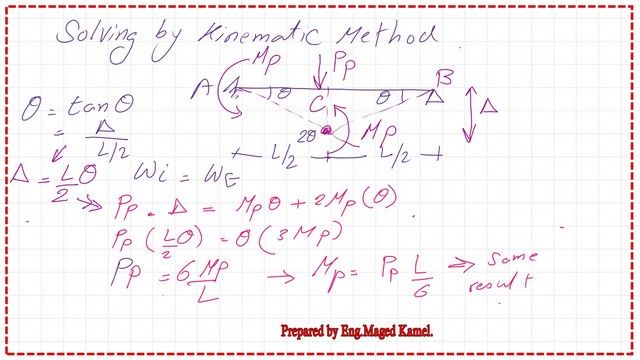
Rb=M*P/L acting downwards to resist the anti-clockwise moment Mp. Taking the Moment at joint B, Mp acting in anti-clockwise, Ra= P/2 +M*P/L.
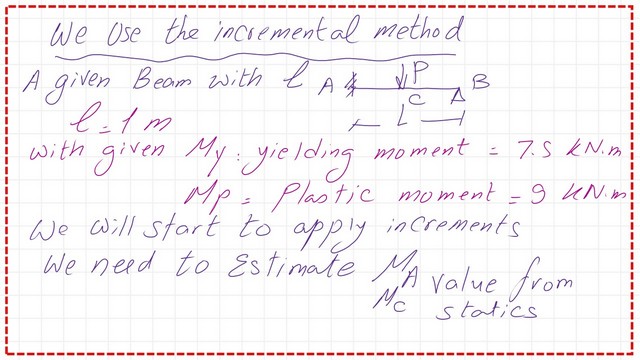
Using the incremental method, incremental loads.
To explain the incremental method, we consider the length of the indeterminate beam to be equal to 1 m. We assume that the yielding moment, My, equals 7.50 kN · m, while the plastic moment equals 9 kN · m. The source is quoted from Dr. C. Caprani, “Structural analysis III.”
We will start to use a workload that creates Moments but with less values than My and Mp.
Due to the fixation at A, the slope of the field end is zero, so we will find the slope due to the negative moment Ma and due the positive moment due to load; using any approved method of structural analysis, we see the slope as the area of the moment values, finally we get the Ma=(3/16)*PL while the positive moment at point C equals 5*PL/32. Please refer to the following slide image for more details.
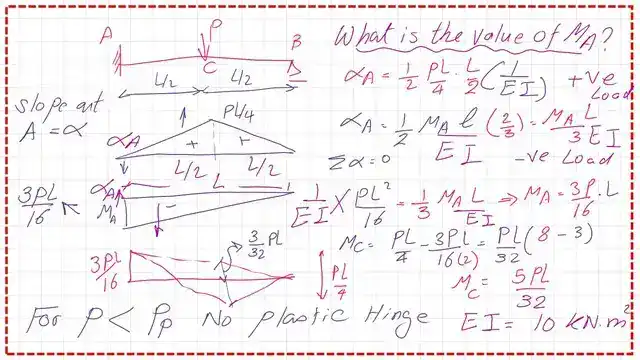
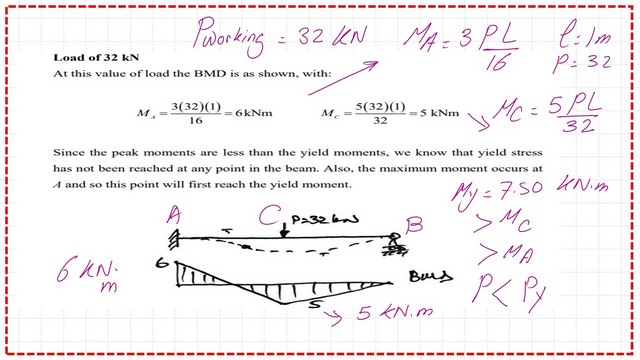
Consider the working load to be 32 kN and estimate the values of the fixed-end Moment at A and the positive moment at C. Compare these values with the value of the Yield Moment. We find that the fixed moment has a bigger value than the positive moment at c.
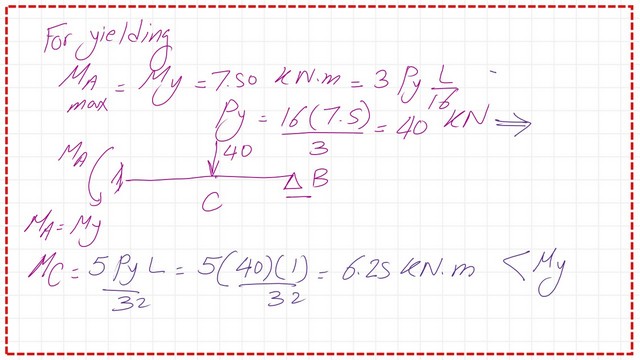
To get the working load that causes the Yielding moment at A, equate that moment to 3Py*L/16; the Py is found to be equal to 40 KN. The positive Moment is 6.25 KN.m, which is less than the My.
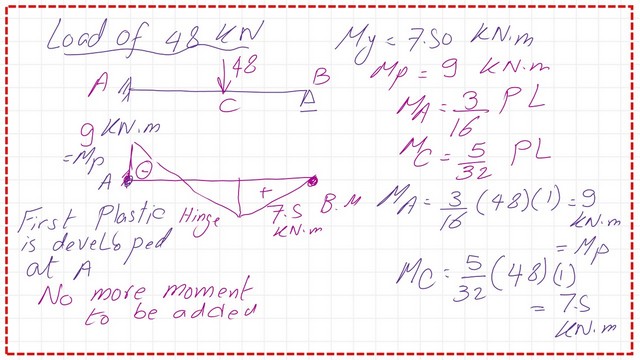
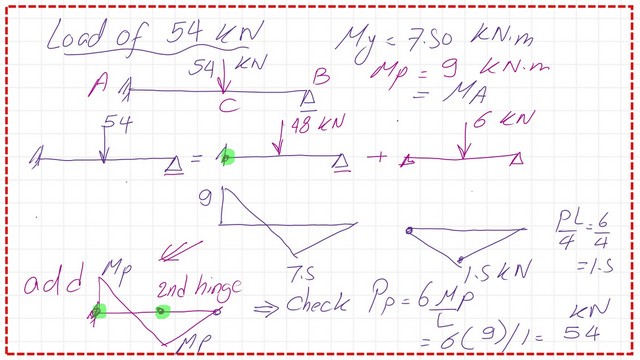
Add More load until you have P = 48 kN; this load creates a first plastic Hinge at A, since Ma = 9 kN · m = Mp. Still, the Mc value is less than Mp and equals 7.50 kN · m.
Increase the Load to 54 KN · m. Since the first hinge forms at a lower load (48 KN · m), there will be no additional moment at the first plastic hinge. The final moments are shown in the following slide image. We have two plastic hinges at Pn = 54 kN. We can use the equation we have derived that Pp=6Mp/L, to verify our answer.
Expressions for the plastic theory.
The first expression is the load factor for a possible load mechanism. λi, lambda i, which is the collapse load divided by the working load to reach Mp. The lambda can be found to be 54/32 = 1.6875.
To calculate the FOS, use the formula: First yield load / Working load. The value equals 40/32=1.25.

The Factor of safety = =P-first yield /P working=40/32=5/4=1.125.
This is valuable data regarding the structural analysis – III.colincaprani.com
The following post discusses upper-bound definitions. The post is an introduction to the theory of upper bound.
