Last Updated on October 21, 2024 by Maged kamel
What is Newton-divided difference interpolation?
Newton has developed a new form for the interpolation of functions. In this post, we will be discussing Newton-divided difference interpolation.
A new form of Quadratic expression was adopted. For a Quadratic function where n=2, we need n+1 points, three points. Our n+1=3 points, namely (xo, x1, x2), and their y coordinates are (yo, y1, y2).
The quadratic function will be written as Q(x) = b0+b1(x-x0) +b2(x-x0) (x-x1). It can be further expanded as Q(x) = bo+b1x- b1*xo +b2(x^2-x*x1-x0*x+x0 *x1). Recalling our polynomial expressed as P2(x)= ao+a1*x+a2*x^2.
Since both functions are the same, we will equate both. For the item of x, we have a1*x=+b1*x-b2*x1*x-b2*x0*x. a1= b1 -b2*x1 -b2*x0.
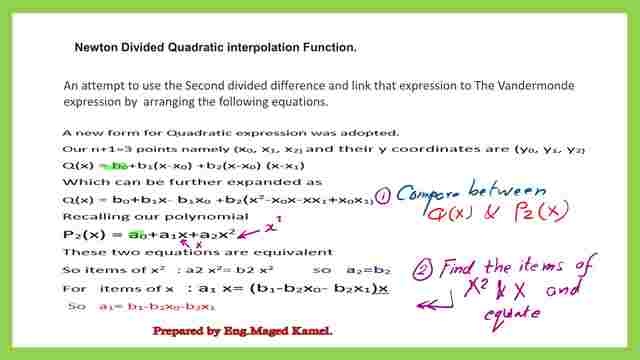
Similarly, For the item of x^2, we have a2*x^2=b2*x^2. Then for the value of the term a2, it will be a2= b2
For the constant item, we have a0= b0-b1*x0+b2*x0*x1. To get values of b0,b1,b2, in terms of the given three Points. Use the first point(x0,y0) to get the value of b0. We can rewrite the Q(x0) as: x=x0,y=y0.
Q(x) = b0+b1(x-x0) +b2(x-x0) (x-x1). Q(x0) =y0= b0+b1(x0-x0) +b2(x0-x0) (x0-x1). b0=y0.
Back to our equation of Q(x), Q(x) = y0+b1(x-x0) +b2(x-x0) (x-x1). For the second point(x1,y1), we can rewrite the Q(x1) as: x=x1,y=y1. From the equation Q(x) = y0+b1(x-x0) +b2(x-x0) (x-x1).
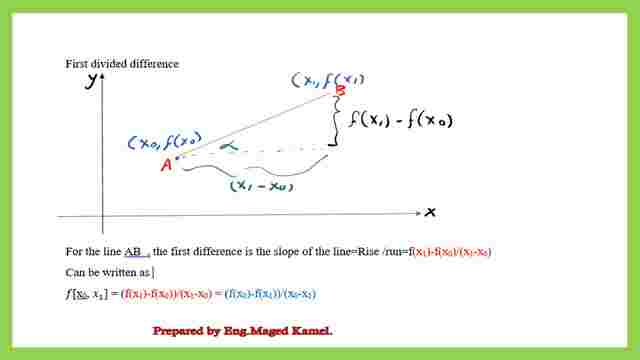
The expression of the first divided difference.
Q(x1) =y1= y0+b1(x1-x0) +b2(x1-x0) (x1-x1). y1= y0+b1(x1-x0)+0 then b1=(y1-y0)/(x1-x0). This is the first divided difference written as f, bracket x0,x1, then bracket.

Back to our equation of Q(x). Q(x) = y0+b1(x-x0) +b2(x-x0) (x-x1). Rewrite as: Q(x) = y0+((y1-y0)/(x1-x0))* (x-x0) +b2(x-x0) (x-x1). For the third point(x2,y2), we can rewrite the Q(x2) as: x=x2,y=y2. From the equation Q(x) = y0+((y1-y0)/(x1-x0))* (x-x0) +b2(x-x0) (x-x1).
Q(x2) =y2= y0+((y1-y0)/(x1-x0))* (x2-x0) +b2(x2-x0) (x2-x1).
The following steps in the next slide picture will illustrate the procedure to get the value of b2.
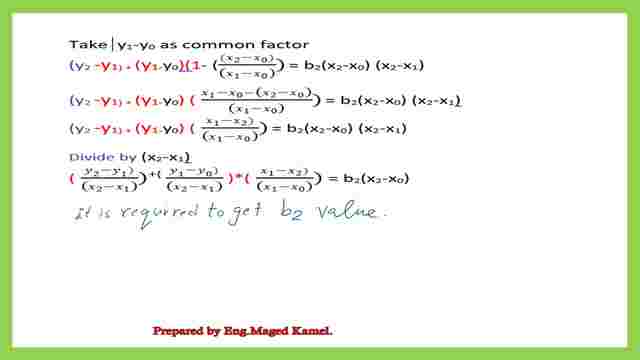
Newton-divided difference or second-divided difference.
For the second divided-difference, which is written as f, bracket x0,x1,x2, then bracket.

This is the final expression for the quadratic polynomial using Newton-divided difference interpolation.
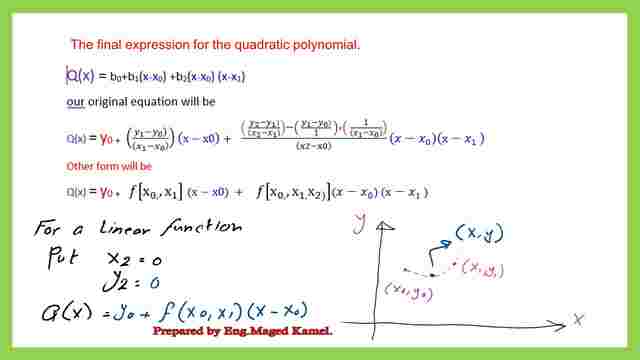
The polynomial is shown in the case of the first order.
The first divided difference is shown in the next slide. A line represents the first divided difference.
The polynomial is shown in the case of the first order. The first divided difference is shown in the next slide. A line represents the first divided difference.
For the Higher-order n value, an expression can be developed and made as a table.
We can make a table for the second divided difference. The next slide image shows the arrangement. The source is from Amos Gilat’s Numerical Methods for Engineers. The clouded area is for a Quadratic polynomial.
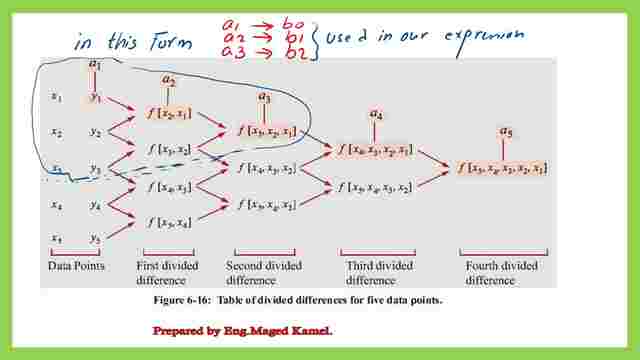
The main advantage of Newton’s divided difference interpolation is that we will not substitute it to make a solution for the n equations. In our case, n=2 is for a quadratic function.
The next post will solve two problems as practice problems for Newton-Divided difference polynomials.
This is a Wiki link for Numerical analysis.
This is a link to Holistic Numerical Methods-Newton divided Differences.
