Last Updated on October 12, 2024 by Maged kamel

Solved problem-5-1-part 1-2.

Solved problem-5-1-part 1-2 for deflection is the title of this post, which is a design example for a simply supported beam.

In this post, Deflection of Steel Beams Part 2, we will select the beam’s W section based on the Zx value.

A solved problem-5-1-part 1-2 for the design of a beam.

The solved problem 5-1 is quoted from the Book of Prof. Fredrick Roland’s book.
Zone -1 bending has M plastic moments, for which Mp=Fy*Zx; the plastic theory is discussed in previous posts.

We have a supported beam with a span of L=30 ft. The steel is ASTM A992, where Fy=50 Ksi and Fult=65 ksi. The dead load is 0.30 kip/ft, and the live load is 0.70 kip/ft.
This solved problem is a design example with given deflection criteria.

This problem was selected after reviewing the previous information for the LRFD and the ASD for the design under bending.
The beam is laterally supported for its entire length. Therefore, the section will be considered compact and in zone 1.

If we start with the LRFD design, we need to estimate the beam’s moment as M=W*L^2/8, cb=1, which is the moment coefficient. The section is fully braced.

Factored Load for LRFD design and the ultimate moment.

First, the Ultimate load =1.20D+1.60 L for D=0.30 kips/ft and L=0.70 Kips/ft, then Wult=1.20*0.30+1.60*0.70=1.48 Kips/ft, The value of Mult will be equal to 1.48*30^2/8=166.50 ft. kips. To convert to Inch kips, multiply by 12, 66.50*12= 1998 inch—kips in the LRFD design.

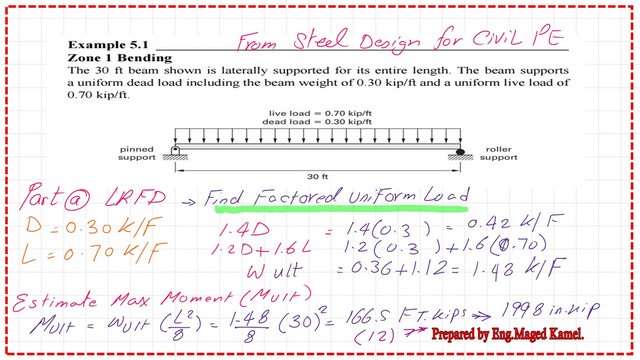
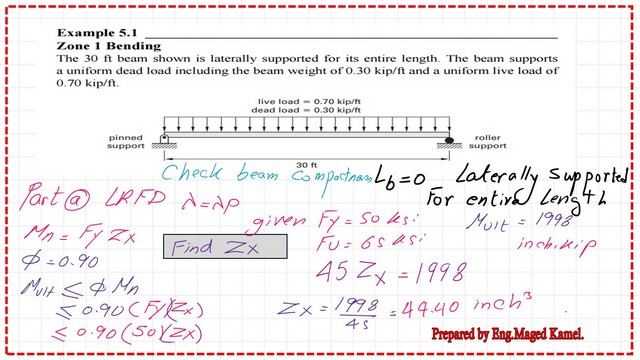
How do we get Zx, which is based on LRFD?

The reduction factor for strength based on the lRFd Φb=0.90 while Ωb=1.67, to check Φb* Ωb=1.50. For step-1. We will estimate Zx from the known formula Mn=Fy*Zx, Fy=50 ksi, then Φb*Mn =Φb*Fy*Zx=Mult, The yield street Fy=50 ksi. The plastic section modulus Zx= Mult/0.90*50* Zx=1998/45=44.50 inch3.

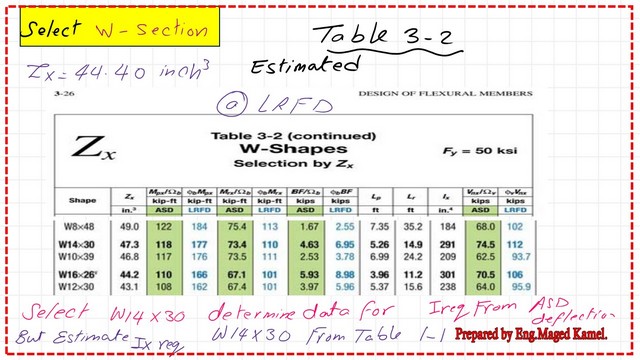
Once we have estimated the required Zx, we will get the necessary W section based on Table 3-2, which arranges sections based on Zx. The first selection is W14x30, but we must check the inertia based on serviceability requirements.

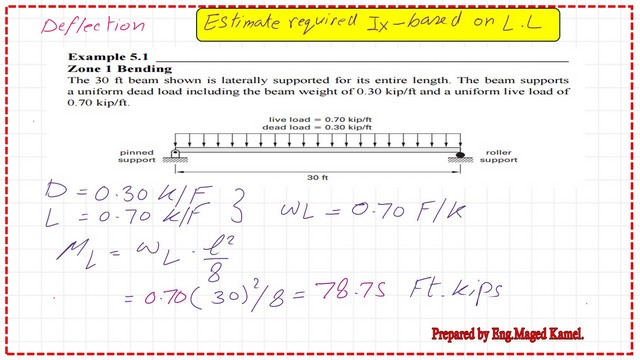
Determine the moments based on Live load and Moment for total load.

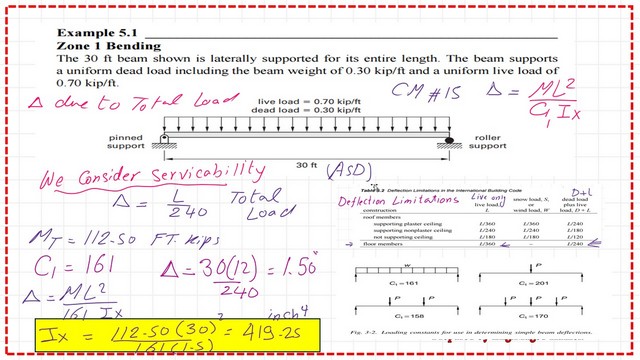
We will estimate the total load, the sum of D plus L, to equal 1.0 kip/Ft. The beam’s momentum based on the total load equals 1*30^2/8=112.50 Ft.kips; please refer to the next slide image.

We estimate the live load moment to be 0.7 k/ft, which equals 78.75 ft. kips.

We will need both moment values to find the deflection values.

Inertia for the beam to give a safe deflection case of D+L.

Deflection is based on Dead and live loads and will be estimated based on where the deflection delta equals L/240. The length is 30 feet. We convert to inches by multiplying by 12.

The deflection value due to the total load Δ-L=L/240=360/240=1.5″. The moment value equals 112.50 Ft.kips, which we estimated earlier.

Use the AISC relation for deflection as equal to ML^2/C1*Ix, where c1=161, equate M*L^2/C1*Ix to 1.50 inch. M is in Ft. Kips, L in Feet. We will get the Ix value as equal to 419.25 inch4.

Inertia for the beam to give a safe deflection case of L.

Checking the deflection of the steel beam based on L/360 for L is required.
We are in the serviceability criteria; we use L=0.70 kips/ft. The deflection due to total live load equals (30* 12/360)=1.00 inch.

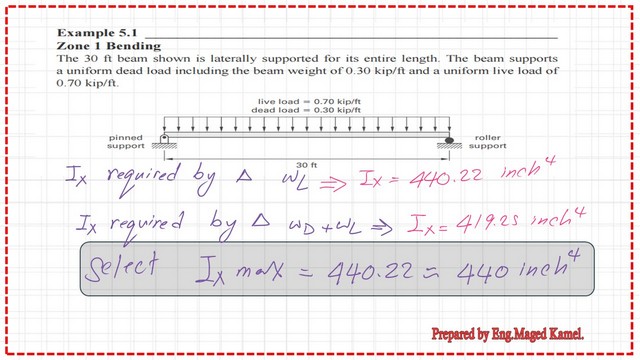
The moment used is the value due to the live load equal to 78.75 Ft.kips. Use the AISC relation for deflection as equal to ML^2/C1*Ix. We will get the Ix value as equal to 440.22 inch4. Please refer to the next slide image for more details.

The final selected Inertia for the beam.

We have two values: the first one is 440.22 inch4, and the second estimated inertia value is 419.25 inch4; we will select the bigger value, which is 440 inch4.
.

In the next post, we will continue our estimation for selecting the final economic section.

As an external resource for the deflection. Chapter 8 – Bending Members.
This is the next post, A solved problem 5-1 part 2-2, how to find LL for a given slender section?