Solved problem 4-16 for k value by using the French equation.
Full description of the content.
Our solved problem, 4-16, from Prof. Alan Williams’s book, Structural Engineering Reference Manual.
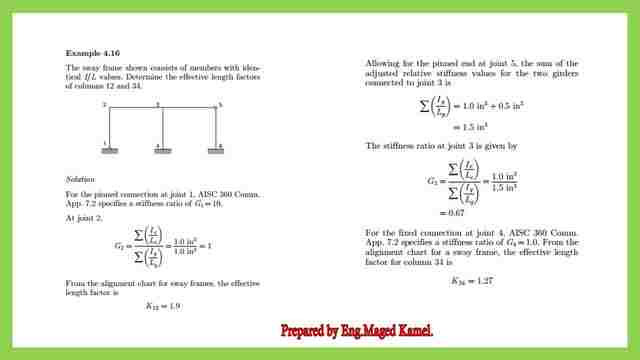

The sway-inhibited frame means Unbraced frame, an Un-stiffened frame, sway frame is shown consisting of members with identical I/L values. it is required to determine the effective length factor for columns here are columns. 12 and 34.
He has not included any values for the length of girders or height of columns, we can consider that h and L are the same, it is required to get the K value for two columns,12 and 34.
We have a solved problem, 4-16 in the previous post, I wanted to attach a copy of the solved problem and the author’s solution. the solution is summarized, but at least we have come to our final solution with more detailed steps.
The French equation was made, For side sway Uninhibited- unbraced frame, for the k value, which is k=sqrt(1.6*GA*Gb+4(Ga+Gb)+7.5)/ sqrt of(GA+Gb+7.5).
Let try our solved problem 4-16 from prof. Alan Williams’s book. as a remark for our Sway frame, our side-sway Uninhibited, for Ga=0, Gb=0, K =1 from the Nomograph chart.
Let us use the French equation, first check the value of k provided by the French equation, against the K value obtained from the Nomograph, put Ga=Gb=0 and substitute, then K=sqrt(0+)0+7.7, the denominator= sqrt(0+0+7.5), then k=sqrt(7.5/7.5)=1, k=1.
This value matches the k obtained from the Nomograph, let us try for Ga=Gb=100. From the chart, it is approximately k=9, the Nomograph rather has a narrow view, from the French equation k=sqrt(1.6100100+7.5)/ sqrt(100+100+7.5).


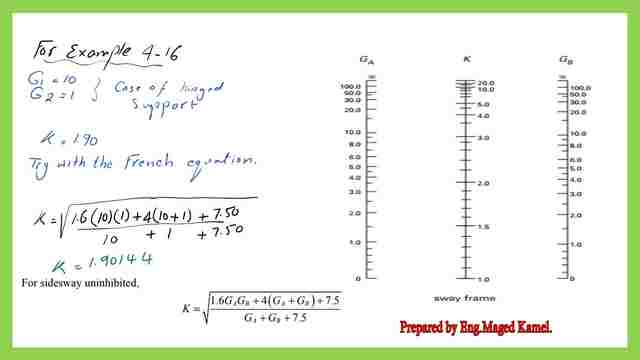
K value for the first column 12 by using the French equation.
Let us refer back to the solved problem 4-16, which consists of two parts, the first part is the hinged support at joint 1 and the second part was for corner joint 2, G1=10, at the hinged support, while G=1, for the joint 2, k from the graph was=1.9.
Using the French equation to get the value for K, then k=sqrt(1.6(10)(1)+4*(10+1)+7.5)/ sqrt (10+1+7.5), sqrt (10+1+7.5), using an excel sheet, we get k=1.90414, which is close to k=1.9 from nomograph to some extent.

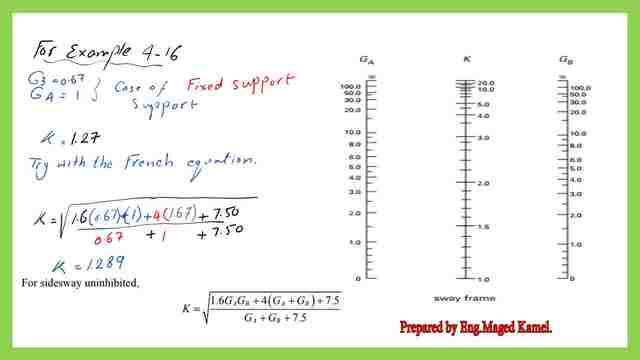
K value for the first column 34 by using the French equation.
The second part of the solved problem 4-16 for fixed support and the joint was ended by pinned supported by pinned support at the far point, while at the near joint was a corner, where m, for the far end, while m=1/2, k was =1.27 from Nomograph. Using the French equation, we get the k value as k=sqrt(1.60.671+4*(2/3+1)+7.5)/ sqrt(0.67+1+7.5), then k=1.289, which is near to the exact value can be used to find a closer figure to the K value estimate by the Nomograph.

This is the pdf file used in the illustration of this post.
A very useful external link is Concentrically Loaded Compression Members.
This is the next post, Modification to Alignment Chart for Braced Frame

