Last Updated on January 2, 2026 by Maged kamel
Product of Inertia Ixy for an isosceles Triangle.
Product of inertia Ixy for an isosceles triangle.
1-To get the expression for the Product of inertia, Ixy, for an isosceles triangle. We will use the previously estimated Ixy equation for the triangle, then readjust it to match an isosceles triangle.
2-The next step is substituting the value of (a ) as a=b/2. In the Ixy formula of an isosceles triangle, the Ixy formula for the triangle. This is the link to the related post. The expression for Ixy for an isosceles triangle is shown in the following formula.
3- In the case of an isosceles, we have a relationship between a and b values as we can substitute for a=b/2 in the previous expression for Ixy for the triangle as follows:
4-The expression for the product of inertia Ixy for an isosceles triangle at point a, which is at the left corner, where we have the intersection between the two axes and y, of the isosceles triangle, is shown in the next slide.
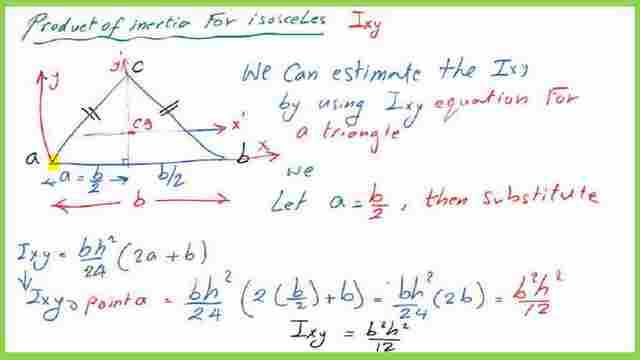
The product of inertia =base^2*height^2/(12).
Product of inertia Ixy for an isosceles at the CG.
For the product of inertia Ixy formula of the isosceles triangle at the CG, we will follow the parallel axis theorem, for which we will deduct the product of area by the x bar * y-bar distance from the CG, square distance from the CG axis from the value of Ixy of an isosceles at the external axis that is coincident with the base.
We have already estimated the Product of inertia Ixy for an isosceles triangle at point a, which is equal to base^2*height^2/(12).
The product of inertia at the Cg of an isosceles triangle Ixcg=Ixy at point a- A*(x-cg)*(y-cg).
The distance xcg=(1/2b), while y cg=(1/3)h, where b is the base length, while h is the height of the isosceles.
The area of the triangle is (1/2)*(b)*(h).
We apply the formula for the product of inertia of a triangle, and we will find the product of inertia for an isosceles triangle at its CG.
We will discover that the Ixy at the Cg equals zero.
The calculations for estimating the Product of Inertia Ixy for an isosceles at the CG are shown in the next slide.
You can download and review the content of this post through the following pdf file.
Here is a link to my YouTube playlist.
For an external resource, the definition of the moment of inertia is provided with solved problems for the 2nd moment of inertia.
For the next post, the moment of inertia Ix for the Trapezium. Step-by-step introduction to the method of estimating the inertia for a trapezium.