Mohr’s circle of inertia-First case.
In this post, we will be talking about Mohr’s circle of inertia-first case. Mohr’s circle of the first case is the case where the moment of inertia about the x-axis is bigger than the moment of inertia about axis Y, and the product of inertia Ixy is positive.
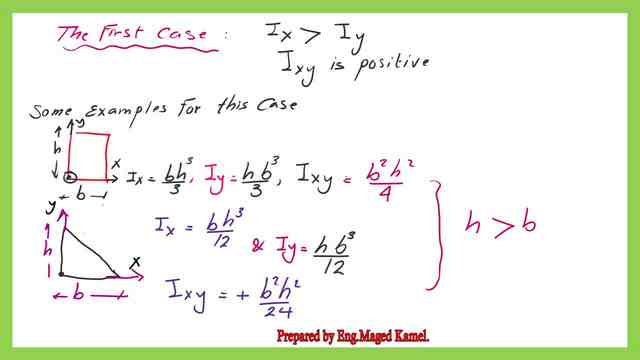
Mohr’s circle of inertia-first case can be found as examples in two cases, the first case is where we have a rectangular shape where the height is bigger than the width of the rectangle. The value of the moment of inertia about the x-axis and Y-axis together with the value of the product of inertia about the left corner is included in the next slide image.
The second case for Mohr’s circle of inertia-first case is the case of inertia about the left corner of a right-angle triangle of dimension (b,h).
The Ix value is (bh^3/12, Iy value is hb^3/12, and the height of the triangle is bigger than the breadth. The product of inertia Ixy is (b^2*h^2/124), which is a positive value.

What is the orientation of the principal axes for Mohr’s circle of inertia-first case?
We have the general expression for the moment of inertia for any oriented axis, we call it Ix’. For Mohr’s circle of the inertia-first case, the value can be a maximum value when the angle 2θ has a cosine of a positive value and the sine value is also negative, this angle is called 2φp1 or ( 2θp1).
This angle is measured from the x-axis. The other orthogonal direction has an angle of 2φp2 or ( 2θp2) is also measured from the x-axis, but this angle will have a negative cosine value and a positive sign value.
The angle 2φp1 will be located in the 4th quarter, while angle 2φp2 will be located in the second quarter.
While drawing Mohr’s circle of inertia, we locate Point A, with 2θ equal to zero, which means that Point A is located at the X-axis. Substituting in the general equation, for the values of Ix’ & Iy’ and Ixy’. The value of Ix’ is equal to Ix while Iy’ value will be equal to Iy, Ixy’ value will be equal to Ixy.
While drawing Mohr’s circle of inertia, we locate Point BA, with 2θ equal to 90 degrees, which means that point B is located at the Y-axis. Substituting in the general equation, for the values of Ix’ & Iy’ and Ixy’. The value of Ix’ is equal to Iy while Iy’ value will be equal to Ix, Ixy’ value will be equal to -Ixy.
The steps used to draw Mohr’s circle of inertia-first case.
The first two steps are to draw the two orthogonal axes.
The first axis represents the Moment of inertia values, Ix, Iy, and Imax, and minimum values., while the second axis represents the values of the product of Inertia Ixy. Locating Points A and B with their respective values of Ix and Iy. Note that the Ixy value of point B is (-Ixy).
Join Points A and B and get the middle point of line AB, this point is the point of the Circle center.
The radius value is the sqrt( (Ix-Iy/2)^2+Ixy^2).
Start from point O and draw the circle with the radius value just estimates. The circle will interest the line in two points C and E.
Angle 2φp1 is the angle between the x-axis and Major axis U, which is the angle enclosed between OA and Line OE. Point E is the point of the maximum value of inertia.
Angle 2φp2 is the angle between the x-axis and Minor axis v, which is the angle enclosed between OA and Line OC. Point C is the point of the minimum value of inertia.
The distance from the vertical axis Ixy to point c will give the value of the Minimum value of inertia, while the distance from the same axis to point E will give the maximum value of inertia.
Need for two mirror points A’ and B’ in Mohr’s circle of inertia-First case.
In the normal view the x-axis is a horizontal axis, while in Mohr’s circle of the inertia-first case, the x-axis is oriented by an angle of 2φp1 from the U-direction. We need a mirror point A’ to let the x-direction in Mohr’s circle of the inertia-first case be a horizontal line.
Setting point A’, which is a mirror of point A and has a coordinate of (ix,-Ixy) will enable us to rotate the x-axis or line OA a clockwise rotation by the value of 2φp1. the x-axis will be horizontal, The U line will also have a new direction represented by line OA’.The other mirror line B’ that has a coordinate of (Iy and Ixy) will be used to get the direction of the minor axis of inertia V.
From the relation of tan 2φp, we have a negative value of tangent which means that the U axis will have an enclosed angle measured in the clockwise direction.
The direction of U and V axes in Mohr’s circle of inertia-first case.
Since the range is a central angle, we can get the direction of U, the major axis in the normal view by joining point C, the point of minimum value of inertia by point A’.
We can use the mirror point b’ to get the direction of minor axes in the normal view. Join Point C with Point B’ to get the V- direction in the normal view.
This is my understanding of the orientation of two axes Ixy and Ix, so the x-axis is horizontal, we can find that point E is the point at which Ix’ = Ix at 2θ = zero and Ix’y’ =Ixy since the line OE is the horizontal line, while point B has Ix’=Iy and Ix’y’=-Ixy.
The line OE is the direction of U, This line has an angle equal to ( -2θp1) measured from the horizontal line OE.
In the next post, we will solve a problem that covers Mohr’s circle of inertia-first case.
This is a link to a useful external resource. Calculator for Cross Section, Mass, Axial and Polar Area Moment of Inertia, and Section Modulus.